Question

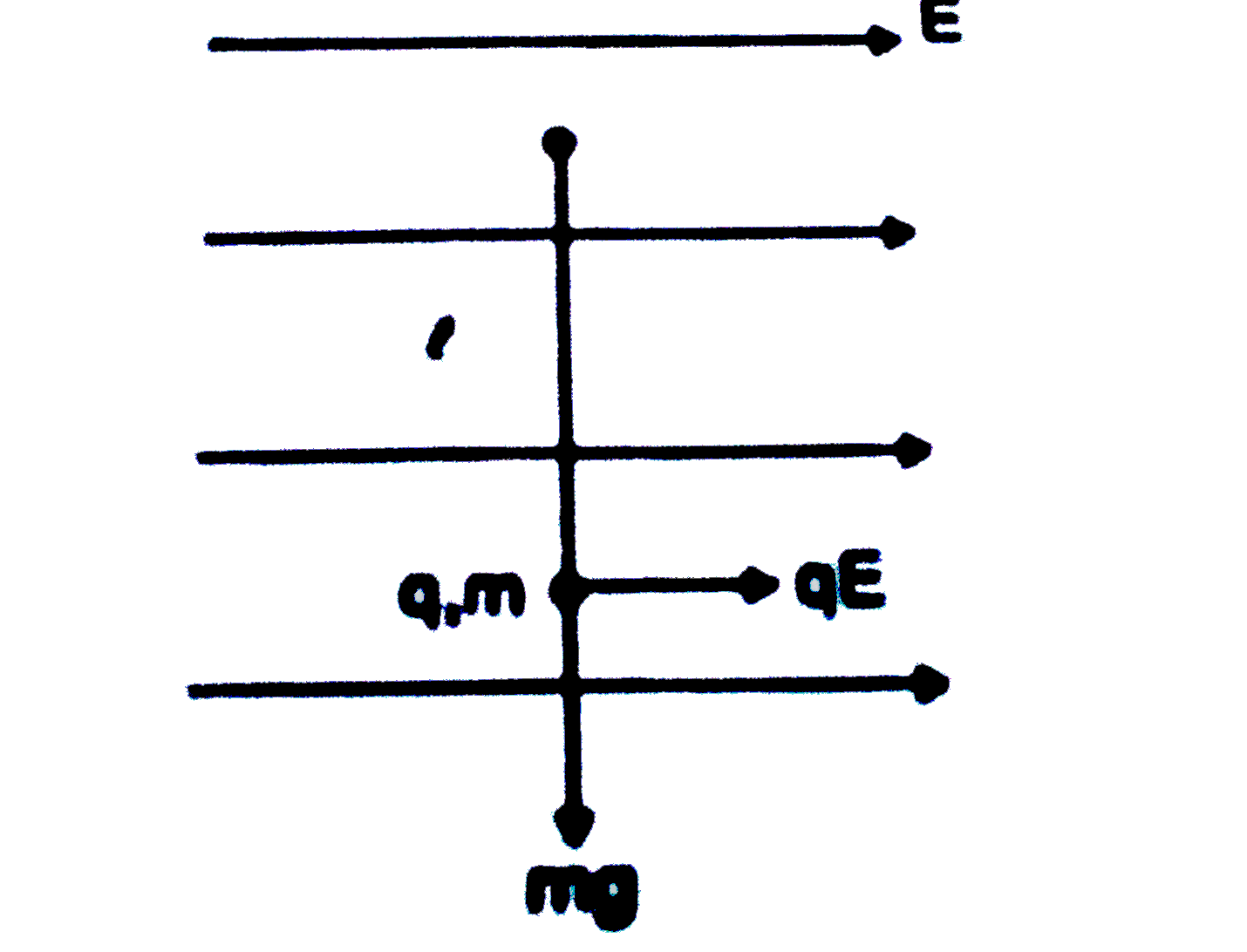
A simple pendulum consists of a small sphere of mass $m$ suspended by a thread of length $l$. the sphere carries a positive charge $q$. The pendulum is placed in a uniform electric field of strength $E$ directed vertically upwards. With what period will pendulum oscillate if the electrostatic force acting on the sphere is less than the gravitational force ?

Explanation

To solve the problem of finding the period of a simple pendulum with a charged sphere in an electric field, we can follow these steps:

1. Identify the Forces Acting on the Sphere:
- The gravitational force acting downward on the sphere is given by:
\( F_g = mg \)
- The electrostatic force acting upward due to the electric field is given by:
\( F_e = qE \)
- Here, \( m \) is the mass of the sphere, \( g \) is the acceleration due to gravity, \( q \) is the charge on the sphere, and \( E \) is the strength of the electric field.

2. Determine the Net Force:
- Since the electrostatic force is less than the gravitational force, the net force acting on the sphere can be expressed as:
\( F_{net} = F_g - F_e = mg - qE \)

3. Relate the Net Force to Effective Acceleration:
- The net force can also be expressed in terms of the effective acceleration (\( g_{effective} \)):
\( F_{net} = m g_{effective} \)
- Therefore, we can equate the two expressions for the net force:
\( mg - qE = mg_{effective} \)

4. Solve for Effective Acceleration:
- Rearranging the equation gives:
\( g_{effective} = g - \frac{qE}{m} \)

5. Write the Formula for the Period of the Pendulum:
- The period \( T \) of a simple pendulum is given by:
\( T = 2\pi \sqrt{\frac{l}{g_{effective}}} \)
- Substituting the expression for \( g_{effective} \) into the formula gives:
\( T = 2\pi \sqrt{\frac{l}{g - \frac{qE}{m}}} \)

Final Answer:
The period of the pendulum oscillating in the electric field is:
\( T = 2\pi \sqrt{\frac{l}{g - \frac{qE}{m}}} \)