A sphere of mass 50 gm is suspended by a string in an electric field of intensity 5NC−1 acting vertically upward. If the tension in the string is 520 millinewton, the charge on the sphere is (g=10ms−2)
−4×10−3C
4×103C
8×103C
−8×10−3C
The correct Answer is:A
To solve the problem, we need to analyze the forces acting on the sphere suspended in the electric field.
1. Identify the Given Values:
- Mass of the sphere, m=50g=0.05kg
- Electric field intensity, E=5N/C
- Tension in the string, T=520mN=520×10−3N=0.52N
- Acceleration due to gravity, g=10m/s2
2. Calculate the Weight of the Sphere:
Weight(W)=m⋅g=0.05kg⋅10m/s2=0.5N
3. Set Up the Force Equation:
- The forces acting on the sphere are the tension T acting upwards and the weight W acting downwards.
- The electric force Fe acting on the sphere due to the electric field is given by Fe=qE, where q is the charge on the sphere.
- According to the equilibrium of forces:
T+Fe=W
Substituting Fe=qE:
T+qE=W
4. Substitute the Known Values:
0.52N+q(5N/C)=0.5N
5. Rearranging the Equation:
q(5)=0.5−0.52
q(5)=−0.02
6. Solve for Charge q:
q=−0.025=−0.004C
q=−4×10−3C
Final Answer:
The charge on the sphere is q=−4mC (milliCoulombs).
---
- Mass of the sphere, m=50g=0.05kg
- Electric field intensity, E=5N/C
- Tension in the string, T=520mN=520×10−3N=0.52N
- Acceleration due to gravity, g=10m/s2
Weight(W)=m⋅g=0.05kg⋅10m/s2=0.5N
- The forces acting on the sphere are the tension T acting upwards and the weight W acting downwards.
- The electric force Fe acting on the sphere due to the electric field is given by Fe=qE, where q is the charge on the sphere.
- According to the equilibrium of forces:
T+Fe=W
Substituting Fe=qE:
T+qE=W
0.52N+q(5N/C)=0.5N
q(5)=0.5−0.52
q(5)=−0.02
q=−0.025=−0.004C
q=−4×10−3C
The charge on the sphere is q=−4mC (milliCoulombs).
Topper's Solved these Questions
- ELECTROSTATICSBook:AAKASH SERIESChapter:ELECTROSTATICSExercise:PRACTICE SHEET (EXERCISE - II INTENSITY OF ELECTRIC FIELD) (LEVEL - II (MAIN) STRAIGHT OBJECTIVE TYPE QUESTION)
Explore 10 Videos
- ELECTROSTATICSBook:AAKASH SERIESChapter:ELECTROSTATICSExercise:PRACTICE SHEET (EXERCISE - II INTENSITY OF ELECTRIC FIELD) (LEVEL - II (MAIN) MORE THAN ONE ANSWER TYPE QUESTION)
Explore 1 Video
- ELECTROSTATICSBook:AAKASH SERIESChapter:ELECTROSTATICSExercise:PRACTICE SHEET (EXERCISE - I COULOMB.S INVERSE SQUARE LAQW) (LEVEL - II (ADVANCED LINKED COMPREHENSION TYPE QUESTION)
Explore 3 Videos
- ELECTROMAGNETICSBook:AAKASH SERIESChapter:ELECTROMAGNETICSExercise:ADDITIONAL EXERCISE
Explore 13 Videos
- ELEMENTS OF VECTORSBook:AAKASH SERIESChapter:ELEMENTS OF VECTORSExercise:QUESTIONS FOR DESCRIPTIVE ANSWERS
Explore 10 Videos
Similar Questions
A sphere of mass 50gm is suspended by a string in an electric field of intensity 5NC−1 acting vertically upward. If the tension in the string is 520 milli newton, the charge on the sphere is (g=10ms−2)
View SolutionA 0.50 gm ball carries a charge of magnitude 10μC. It is suspended by a string in a downward electric field of intensity 300N/C. If the charge on the ball is positive, then the tension in the string is (g=10ms−2)
View SolutionA block of mass 10 kg is suspended by three strings as shown in figure . Tension T2 is

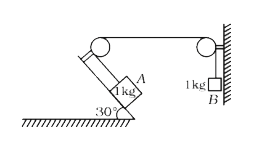
View SolutionCalculate the tension in the string shown in the figure. All the surfaces are frictionless. (Take g=10/ms−1)
View SolutionA charged particle of mass 5×10−5kg is held stationary in space by placing it in an electric field of strength 107NC−1 directed vertically downwards. The charge on the particle is
View SolutionA small metallic sphere of mass m is suspended from the ceiling of a car accelerating on a horizontal road with constant acceleration a. The tension in the string attached with metallic sphere is
View SolutionA pendulum consisting of a small sphere of mass m, suspended by a inextensible and massless string of length 1 , is made to swing in a vertical plane. If the breaking strength of the string is 2 mg, then the maximum angular amplitude of the displacement from the vertical can be
View SolutionA stone of mass 1 kg tied at the end of a string of length 1 m and is whirled in a verticle circle at a constant speed of 3ms−1. The tension in the string will be 19 N when the stone is (g=10ms−1)
View SolutionA sphere of mass 20 g is whirled around in a horizontal circular path on the end of a string, the other end of which is stationary. If the tension in the string is 12.62 N, calculate the length of the string.
View SolutionA pendulum consisting of a small sphere of mass m, suspended by a inextensible and massless string of length 1 , is made to swing in a verticle plane. If the breaking strength of the string is 2 mg, then the maximum angular amplitude of the displacement from the verticle can be
View Solution
