Inside a uniform shell
potential is variable
potential is zero
potential is constant
All of these
The correct Answer is:C
Step-by-Step Solution
1. Understanding the Concept: We need to analyze the gravitational field and gravitational potential inside a uniform spherical shell. According to the shell theorem, the gravitational field inside a uniform spherical shell is zero.
2. Gravitational Field Inside the Shell: Since the gravitational field g inside the shell is zero, we can write:
g=0
3. Relation Between Gravitational Field and Potential: The gravitational potential V is related to the gravitational field by the equation:
g=−dVdr
where r is the distance from the center of the shell. Since g=0 inside the shell, we can conclude:
−dVdr=0
4. Conclusion About Gravitational Potential: If the derivative of the potential V with respect to r is zero, it implies that the gravitational potential V is constant throughout the interior of the shell.
5. Final Statement: Thus, we conclude that the gravitational potential inside a uniform spherical shell is constant.
Summary
- Inside a uniform spherical shell, the gravitational field is zero.
- Consequently, the gravitational potential is constant throughout the interior of the shell.
g=0
g=−dVdr
where r is the distance from the center of the shell. Since g=0 inside the shell, we can conclude:
−dVdr=0
- Inside a uniform spherical shell, the gravitational field is zero.
- Consequently, the gravitational potential is constant throughout the interior of the shell.
Topper's Solved these Questions
- GRAVITATIONBook:DC PANDEY ENGLISHChapter:GRAVITATIONExercise:Check Point 10.4
Explore 10 Videos
- GRAVITATIONBook:DC PANDEY ENGLISHChapter:GRAVITATIONExercise:Check Point 10.5
Explore 20 Videos
- GRAVITATIONBook:DC PANDEY ENGLISHChapter:GRAVITATIONExercise:Check Point 10.2
Explore 20 Videos
- GENERAL PHYSICSBook:DC PANDEY ENGLISHChapter:GENERAL PHYSICSExercise:INTEGER_TYPE
Explore 2 Videos
- KINEMATICSBook:DC PANDEY ENGLISHChapter:KINEMATICSExercise:INTEGER_TYPE
Explore 10 Videos
Similar Questions
Inside a uniform spherical shell
View SolutionA : The gravitational field intensity is zero everywhere inside a uniform spherical shell
R: The net force on a point mass inside a uniform spherical shell is zero everywhere .
View SolutionA particle placed
1.inside a uniform spherical shell of mass M, but not at the center
2.inside a uniform spherical shell of mass M, at the center
3.outside a uniform spherical shell of mass M, a distance r from the center
4.outside a uniform solid sphere of mass M, a distance 2r from the center
If F1,F2,F3 and F4 are gravitational forces acting on the particle in four cases.
View SolutionA current carrying loop of radius R and mass 'm' is placed inside a uniform magnetic field an shown

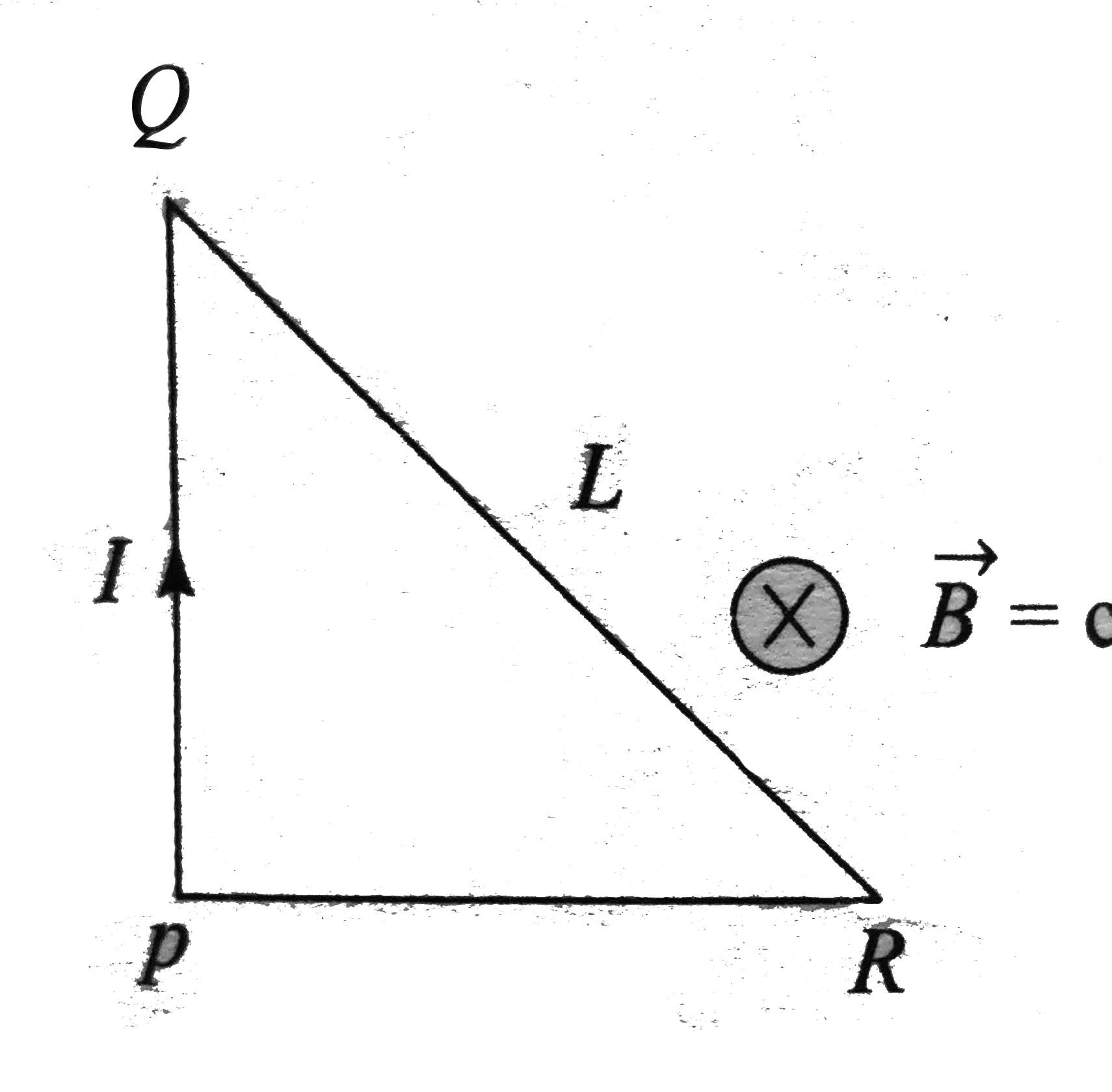
View SolutionThe rigid conducting thin wire frame carries an electric current I and this frame is inside a uniform magnetic field →B as shown in Fig. 1.146. Then,
View SolutionConsider a thin uniform spherical layer of mass M and radius R. The potential energy of gravitational interaction of matter forming this shell is :
View SolutionCalculate the self-gravitational potential energy of matter forming a. a thin uniform shell of mass M and radius R, b. a uniform sphere of mass m and radius R.
View SolutionAn empty thick conducting shell of inner radius a and outer radius b is shown in figure. If it is obseved that the inner face of the carries a uniform charge density −σ and the surface carries a uniform charge density .σ.

If a point charge qA is placed at the center of the shell, then choose the correct statement(s)
View SolutionA particle of mass M is placed at the centre of a uniform spherical shell of equal mass and radius a. Find the gravitational potential at a point P at a distance a2 from the centre.
View SolutionA particle of mass M is placed at the centre of a uniform spherical shell of equal mass and radius a. Find the gravitational potential at a point P at a distance a2 from the centre.
View Solution