A 2 kg block of wood rests on a long table top. A 5 g bullet moving horizontally with a speed of 150ms−1 is shot into the block and sticks to it. The block then slides 2.7m along the table top and comes to a stop. The force of friction between the block and the table is
0.052 N
3.63 N
2.50 N
1.04 N
The correct Answer is:A
To solve the problem, we will follow these steps:
Step 1: Identify the given data
- Mass of the block (M) = 2 kg
- Mass of the bullet (m) = 5 g = 0.005 kg (convert grams to kilograms)
- Initial speed of the bullet (v₁) = 150 m/s
- Distance traveled by the block after the bullet sticks (s) = 2.7 m
Step 2: Use conservation of momentum to find the final velocity (v_f) after the bullet sticks to the block
According to the law of conservation of momentum:
Initial momentum=Final momentum
m⋅v1+M⋅0=(M+m)⋅vf
Substituting the values:
0.005⋅150+2⋅0=(2+0.005)⋅vf
Calculating the left side:
0.75=2.005⋅vf
Now, solving for vf:
vf=0.752.005≈0.374 m/s
Step 3: Calculate the deceleration (a) using the equation of motion
We will use the equation:
v2=u2+2as
Where:
- v = final velocity = 0 (the block comes to a stop)
- u = initial velocity = vf = 0.374 m/s
- s = distance = 2.7 m
- a = acceleration (deceleration in this case)
Rearranging the equation gives:
0=(0.374)2+2a(2.7)
0.139876=−5.4a
Now solving for a:
a=−0.1398765.4≈−0.0259 m/s2
Step 4: Calculate the frictional force (F_f)
The frictional force can be calculated using Newton's second law:
Ff=(M+m)⋅a
Substituting the values:
Ff=(2+0.005)⋅(−0.0259)
Ff=2.005⋅(−0.0259)≈−0.0519 N
The negative sign indicates that the frictional force acts in the opposite direction of motion.
Step 5: Present the final answer
The magnitude of the frictional force is approximately:
Ff≈0.052 N
- Mass of the block (M) = 2 kg
- Mass of the bullet (m) = 5 g = 0.005 kg (convert grams to kilograms)
- Initial speed of the bullet (v₁) = 150 m/s
- Distance traveled by the block after the bullet sticks (s) = 2.7 m
According to the law of conservation of momentum:
Initial momentum=Final momentum
m⋅v1+M⋅0=(M+m)⋅vf
Substituting the values:
0.005⋅150+2⋅0=(2+0.005)⋅vf
Calculating the left side:
0.75=2.005⋅vf
Now, solving for vf:
vf=0.752.005≈0.374 m/s
We will use the equation:
v2=u2+2as
Where:
- v = final velocity = 0 (the block comes to a stop)
- u = initial velocity = vf = 0.374 m/s
- s = distance = 2.7 m
- a = acceleration (deceleration in this case)
0=(0.374)2+2a(2.7)
0.139876=−5.4a
Now solving for a:
a=−0.1398765.4≈−0.0259 m/s2
The frictional force can be calculated using Newton's second law:
Ff=(M+m)⋅a
Substituting the values:
Ff=(2+0.005)⋅(−0.0259)
Ff=2.005⋅(−0.0259)≈−0.0519 N
The negative sign indicates that the frictional force acts in the opposite direction of motion.
The magnitude of the frictional force is approximately:
Ff≈0.052 N
Topper's Solved these Questions
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion and reason
21 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Match the coloumns
9 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Check points
50 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery
38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
Similar Questions
A block on table shown in figure is just on the wedge of slipping. Find the coefficient of static friction between the block and table top.

View SolutionA block A of mass m1 rests on a horizontal table. A light string connected to it passes over a frictionless pulley at the edge of table and from its other end another block B of mass m2 is suspended. The coefficient of knetic friction between the block and table is μk . When the block A is sliding on the table, the tension in the string is.
View SolutionA horizontal force of 20 N is applied to a block of mass 4 kg resting on a rough horizontal table. If the block does not move on the table, how much frictional force the table is applying on the block. What can be said about the coefficient of static friction between the block and the table? Takeg=10ms2.
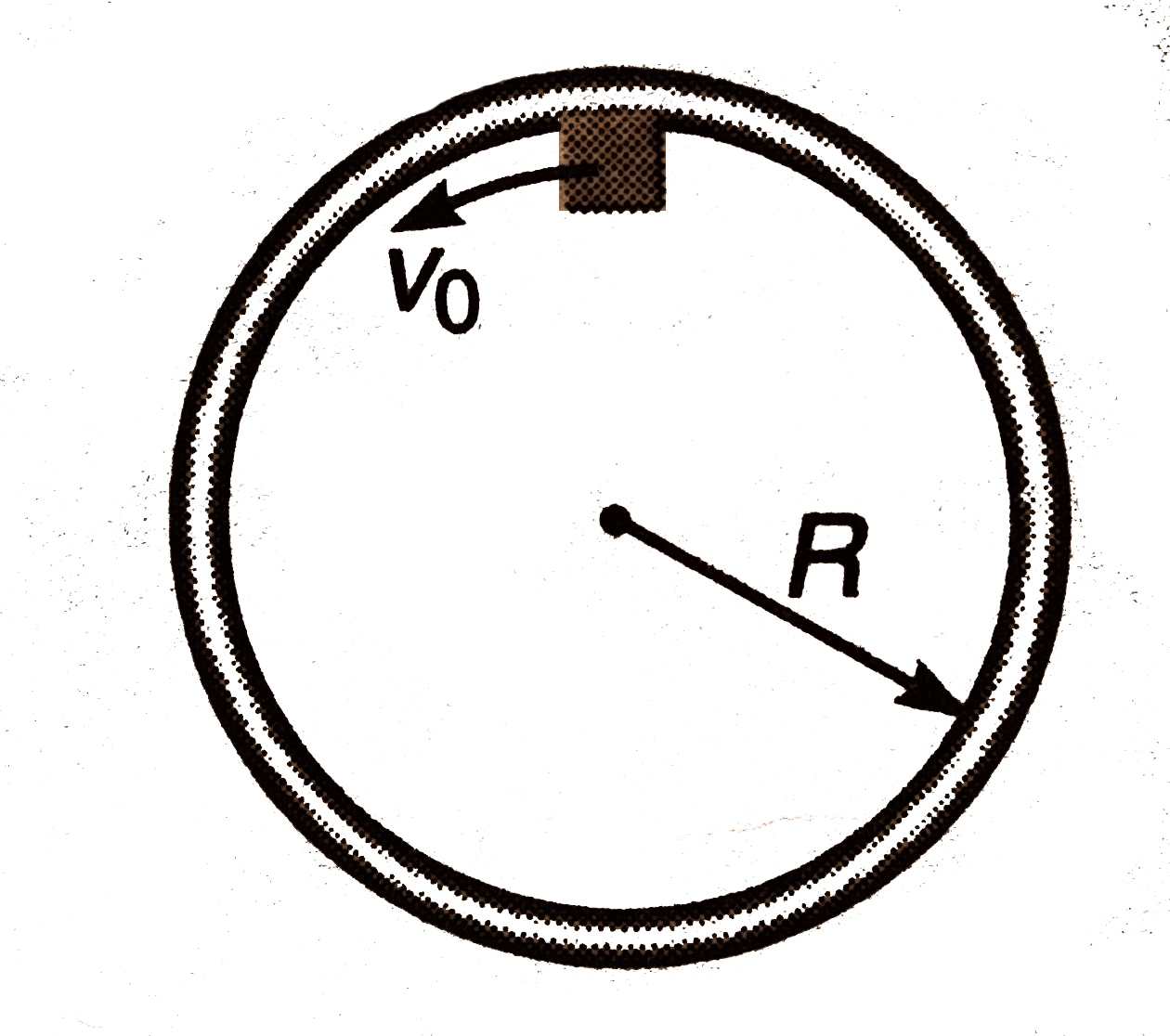
View SolutionA block of mass m slides 0n a frictionless table. It is constrained to move inside a ring of radius R . At time t=0 , block is moving along the inside of the ring (i.e. in the tangential direction) with velocity v0 . The coefficient of friction between the block and the ring is μ . Find the speed of the block at time t .
View SolutionA bullet of mass 40 g moving with a speed of 90ms−1 enters a heavy wooden block and is stopped after a distance of 60 cm . The average resistive force exerted by the block on the bullet is :
View SolutionA block of mass m is stationary on a rough inclined plane making an angle θ with the horizontal. Find the contact force between block and the plane
View SolutionA man of mass 60 kg sitting on ice pushes a block of mass of 12kg on ice horizontally with a speed of 5 ms−1. The coefficient of friction between the man and ice and between block and ice is 0.2. If g =10 ms−2, the distances between man and the block, when they come to rest is
View SolutionTwo blocks A and B are connected to each other by a string and a spring, the string passes over a frictionless pulley as shown in the figure. Block B slides over the horizontal top surface of a stationary block C and the block A slides along the vertical side of C, both with the same uniform speed. The coefficient of friction between the surfaces of blocks is 0.2. Force constant of the spring is 1960 newtons/m. If mass of block A is 2 kg. The mass of block B and the energy stored in the spring are _____ kg and _____ J.

View SolutionA block of mass 1kg moving with a speed of 4ms−1, collides with another block of mass 2kg which is at rest. The lighter block comes to rest after collision. The loss in KE of the system is
View SolutionA bullet of mass 10g travelling horizontally with a velocity of 150ms−1 strikes a stationary wooden block and come to rest in 0.03s. Calculate the distance of penetration of the bullet into the block. Also, Calculate the magnitude of the force exerted by the wooden block on the bullet,
View Solution
