Two blocks of msses 10 kg and 30 kg are placed along a vertical line. The first block is raised through a height of 7 cm. By what distance should the second mass be moved to raise the centre of mass by 1 cm?
2 cm upward
1 cm upward
2cm downward
1 cm downward
The correct Answer is:D
To solve the problem step by step, let's denote the masses and their movements clearly:
1. Identify the masses and their initial positions:
- Let m1=10kg (first block)
- Let m2=30kg (second block)
- The first block is raised through a height of h1=7cm.
2. Determine the initial position of the center of mass (CM):
- The initial position of the center of mass can be calculated using the formula:
yCM=m1y1+m2y2m1+m2
- Assume the initial position of m1 (10 kg) is at y1=0 cm and m2 (30 kg) is at y2=h cm (we can assume h is the height of the second block).
3. Calculate the initial center of mass:
- The initial center of mass is:
yCM,initial=10⋅0+30⋅h10+30=30h40=3h4
4. Calculate the new position of the first block after being raised:
- After raising the first block by 7 cm, its new position becomes y′1=7cm.
5. Calculate the new center of mass:
- The new center of mass after raising the first block is:
yCM,new=10⋅7+30⋅y′210+30=70+30y′240
6. Set the change in center of mass to 1 cm:
- The problem states that the center of mass is raised by 1 cm:
yCM,new−yCM,initial=1cm
- Substituting the expressions we derived:
70+30y′240−3h4=1
7. Simplify and solve for y′2:
- Multiply through by 40 to eliminate the denominator:
70+30y′2−30h=40
- Rearranging gives:
30y′2=40−70+30h
30y′2=30h−30
y′2=h−1
8. Determine the distance the second mass should be moved:
- Since we need to find the distance d that the second mass should be moved, we can express it as:
d=h−y2
- If the second mass is initially at height h and needs to be moved to height h−1, then:
d=−1cm
- This indicates that the second mass must be moved downward by 1 cm.
Final Answer: The second mass should be moved downward by 1 cm.
- Let m1=10kg (first block)
- Let m2=30kg (second block)
- The first block is raised through a height of h1=7cm.
- The initial position of the center of mass can be calculated using the formula:
yCM=m1y1+m2y2m1+m2
- Assume the initial position of m1 (10 kg) is at y1=0 cm and m2 (30 kg) is at y2=h cm (we can assume h is the height of the second block).
- The initial center of mass is:
yCM,initial=10⋅0+30⋅h10+30=30h40=3h4
- After raising the first block by 7 cm, its new position becomes y′1=7cm.
- The new center of mass after raising the first block is:
yCM,new=10⋅7+30⋅y′210+30=70+30y′240
- The problem states that the center of mass is raised by 1 cm:
yCM,new−yCM,initial=1cm
- Substituting the expressions we derived:
70+30y′240−3h4=1
- Multiply through by 40 to eliminate the denominator:
70+30y′2−30h=40
- Rearranging gives:
30y′2=40−70+30h
30y′2=30h−30
y′2=h−1
- Since we need to find the distance d that the second mass should be moved, we can express it as:
d=h−y2
- If the second mass is initially at height h and needs to be moved to height h−1, then:
d=−1cm
- This indicates that the second mass must be moved downward by 1 cm.
Topper's Solved these Questions
- CENTRE OF MASSBook:DC PANDEY ENGLISHChapter:CENTRE OF MASSExercise:Assertion and reason
Explore 21 Videos
- CENTRE OF MASSBook:DC PANDEY ENGLISHChapter:CENTRE OF MASSExercise:Match the coloumns
Explore 9 Videos
- CENTRE OF MASSBook:DC PANDEY ENGLISHChapter:CENTRE OF MASSExercise:Check points
Explore 50 Videos
- CALORIMETRY AND HEAT TRANSFERBook:DC PANDEY ENGLISHChapter:CALORIMETRY AND HEAT TRANSFERExercise:Medical entrance s gallery
Explore 38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, IMPULSE AND MOMENTUMExercise:Comprehension type questions
Explore 15 Videos
Similar Questions
Two bocks of masses 10 g and 20 kg are plced on the X-axis. The first mass is moved on the axis by a distance of 2 cm. By what distance should the second mass be moved to keep the position of the centre of mass unchanged?
View SolutionConsider a system of two particles having masses 2kg and 5 kg the particle of mass 2 kg is pushed towards the centre of mass of particles through a distance 5 m,by what distance would particle of mass 5kg move so as to keep the centre of mass of particles at the original position?
View Solution
Knowledge Check
Two blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.
 A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
A0.7ms−2B0.8ms−2C0.6ms−2D0.5ms−2
Two blocks of masses 2 kg and 1 kg are in contact with each other on a frictionless table. When a horizontal force of 3.0 N is applied to the block of mass 2 kg the value of the force of contact between the two blocks is:
View SolutionTwo blocks of masses 2 kg and 1 kg are in contact with each other on a frictionless table. When a horizontal force of 3.0 N is applied to the block of mass 2 kg the value of the force of contact between the two blocks is:
View SolutionA block of mass 1 kg is placed on a horizontal surface . The reaction force to the weight of the block is
View SolutionFour particles of masses 1kg, 2kg, 3kg and 4kg are placed at the four vertices A,B,C and D of a square of side 1m. Find square of distance of their centre of mass from A.
View SolutionTwo blocks of mass 10kg and 30kg are kept as shown in the figure. The surface between the block is fricitional while the ground is smooth. A horizontal force of magnitude 60N is applied horizontally on the upper block. Find the frictional force on block of mass 30kg ?

View SolutionTwo block of masses 2kg and 4kg are released from rest over a smooth inclined place of inclination 30∘ as shown in figure what is the normal force between the two blocks?
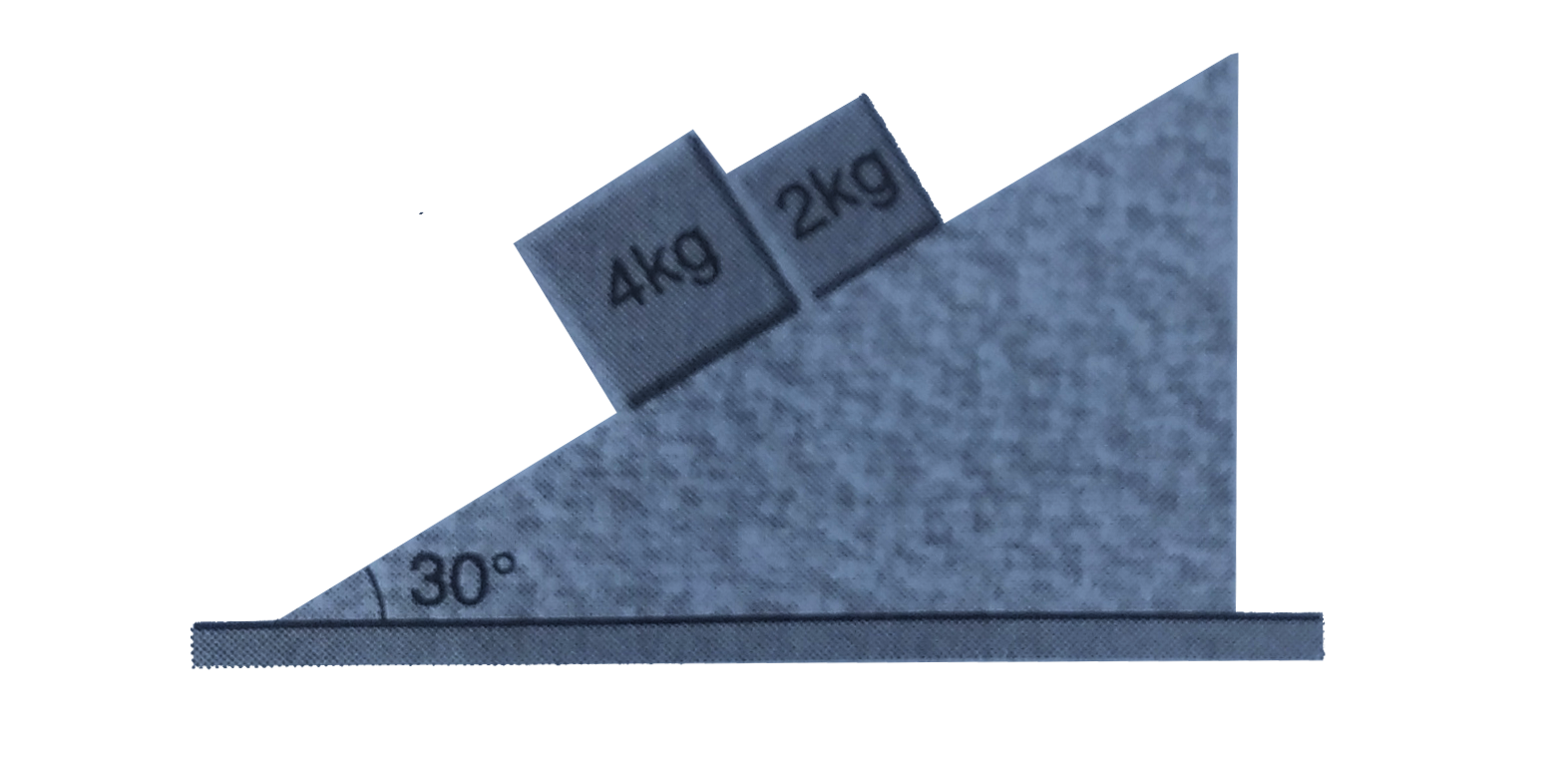
View SolutionTwo blocks of masses 1 kg and 2 kg are placed in contact on a smooth horizontal surface as shown in fig. A horizontal force of 6N is applied d(a) on a 1-kg block. Find the force of interaction of the blocks.

 .
.
View Solution