In YDSE, both slits produce equal intensities on the screen. A 100% transparent thin film is placed in front of one of the slits. Now, the intensity on the centre becomes 75% of the previous intensity. The wavelength of light is 6000Å and refractive index of glass is 1.5. Thus, minimum thickness of the glass slab is
0.2μm
0.3μm
0.4μm
0.5μm
The correct Answer is:A
To solve the problem, we need to find the minimum thickness of the glass slab that causes the intensity at the center of the interference pattern to reduce to 75% of its original value. Here’s the step-by-step solution:
Step 1: Understand the Initial Condition
In the Young's Double Slit Experiment (YDSE), both slits produce equal intensities on the screen. Let's denote the initial intensity at the center as I0.
Step 2: Determine the New Intensity
After placing the 100% transparent thin film in front of one of the slits, the intensity at the center becomes 75% of the original intensity. Therefore, the new intensity I can be expressed as:
I=0.75I0=34I0
Step 3: Relate Intensity to Phase Difference
In YDSE, the intensity at the center can be expressed in terms of the phase difference ϕ as:
I=Imaxcos2(ϕ2)
where Imax is the maximum intensity.
Step 4: Set Up the Equation
Since the new intensity is 34Imax, we can set up the equation:
34Imax=Imaxcos2(ϕ2)
Dividing both sides by Imax gives:
34=cos2(ϕ2)
Step 5: Solve for Phase Difference
Taking the square root of both sides, we find:
cos(ϕ2)=√32
This implies:
ϕ2=π6⇒ϕ=π3
Step 6: Relate Phase Difference to Path Difference
The phase difference ϕ is related to the path difference Δx by the equation:
ϕ=2πλΔx
Substituting ϕ=π3:
π3=2πλΔx
Solving for Δx:
Δx=λ6
Step 7: Calculate the Path Difference Due to the Glass Slab
When a glass slab of thickness t is placed in front of one of the slits, the effective path difference Δx is given by:
Δx=(n−1)t
where n is the refractive index of the glass. Here, n=1.5.
Step 8: Set Up the Equation for Thickness
Equating the two expressions for path difference:
(n−1)t=λ6
Substituting n=1.5 and λ=6000Å=6000×10−10m:
(1.5−1)t=6000×10−106
0.5t=6000×10−106
t=6000×10−103=2000×10−10m=2000Å
Final Answer
The minimum thickness of the glass slab is:
2000Åor0.2μm
In the Young's Double Slit Experiment (YDSE), both slits produce equal intensities on the screen. Let's denote the initial intensity at the center as I0.
After placing the 100% transparent thin film in front of one of the slits, the intensity at the center becomes 75% of the original intensity. Therefore, the new intensity I can be expressed as:
I=0.75I0=34I0
In YDSE, the intensity at the center can be expressed in terms of the phase difference ϕ as:
I=Imaxcos2(ϕ2)
where Imax is the maximum intensity.
Since the new intensity is 34Imax, we can set up the equation:
34Imax=Imaxcos2(ϕ2)
Dividing both sides by Imax gives:
34=cos2(ϕ2)
Taking the square root of both sides, we find:
cos(ϕ2)=√32
This implies:
ϕ2=π6⇒ϕ=π3
The phase difference ϕ is related to the path difference Δx by the equation:
ϕ=2πλΔx
Substituting ϕ=π3:
π3=2πλΔx
Solving for Δx:
Δx=λ6
When a glass slab of thickness t is placed in front of one of the slits, the effective path difference Δx is given by:
Δx=(n−1)t
where n is the refractive index of the glass. Here, n=1.5.
Equating the two expressions for path difference:
(n−1)t=λ6
Substituting n=1.5 and λ=6000Å=6000×10−10m:
(1.5−1)t=6000×10−106
0.5t=6000×10−106
t=6000×10−103=2000×10−10m=2000Å
The minimum thickness of the glass slab is:
2000Åor0.2μm
Topper's Solved these Questions
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - LEVEL 2 (single correct option)
1 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 2 More Than One Correct
6 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Subjective Questions
5 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - All Questions
135 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
Similar Questions
In young's double-slit experiment, both the slits produce equal intensities on a screen. A 100% transparent thin film of refractive index μ=1.5 is kept in front of one of the slits, due to which the intensity at the point O on the screen becomes 75% of its initial value. If the wavelength of monochromatic light is 720 nm, then what is the minimum thickness (in nm) of the film?
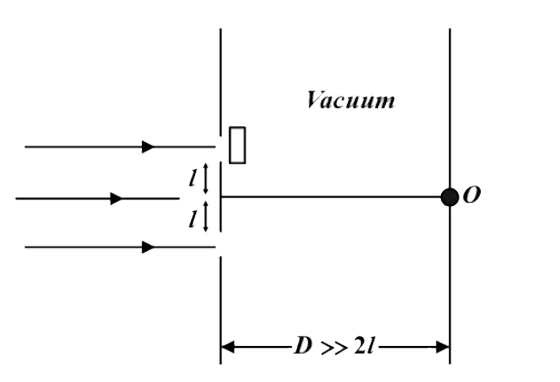
View SolutionIn YDSE the distance between the slits is 1mm and screen is 25nm away from intensities IF the wavelength of light is 6000A the fringe width on the screen is
View SolutionMinimum thickness of a mica sheet having μ=32 which should be placed in front of one of the slits in YDSE is required to reduce the intensity at the centre of screen to half of maximum intensity is
View SolutionIn a doulble slit experiment when a thin film of thickness t having refractive index μ is introduced in from of one of the slits, the maximum at the centre of the fringe pattern shifts by one width. The value of t is (λ is the wavelength of the light used)
View SolutionIn YDSE if a slab whose refractive index can be varied is placed in front of one of the slits. Then, the variation of resultant intensity at mid-point of screen with μ will be best represented by (mu is greater than or equal to 1)
View SolutionIn YDSE if a slab whose refractive index can be varied is placed in front of one of the slits. Then, the variation of resultant intensity at mid-point of screen with μ will be best represented by (mu is greater than or equal to 1)
View SolutionIn YDSE, D = 1.2m and d= 0.25cm, the slits are illuminated with coherent 600nm light. Calculate the distance y above the central maximum for which the average intensity on the screen is 75% of the maximum.
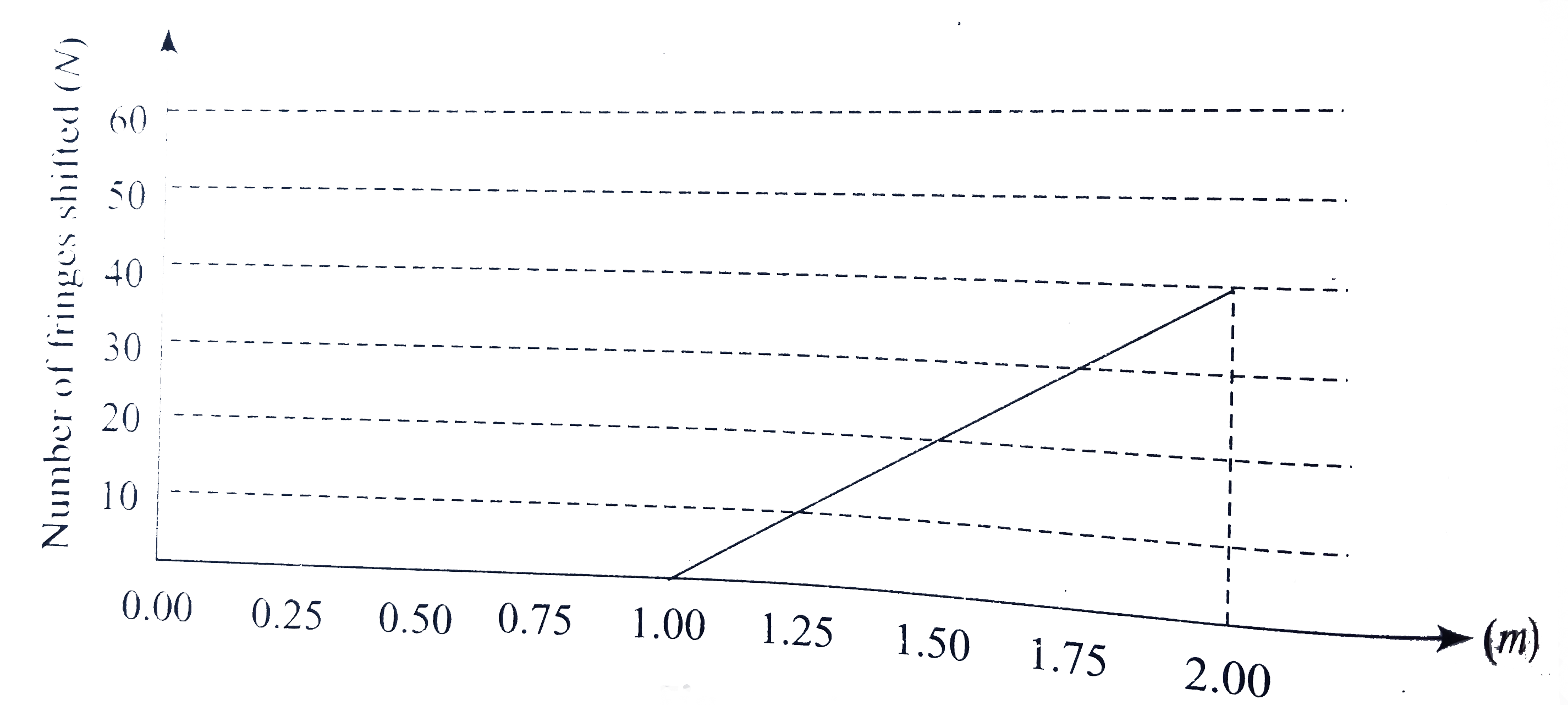
View SolutionThe slits in a double-slit interference experiment are illuminated by orange light (λ=60nm). A thin transparent plastic of thickness t is placed in front of one of the slits. The nunber of fringes shifting on screen is plotted versus the refractive index μ of the plastic in graph shown in figure. The value of t is
View SolutionIn the ideal double-slit experiment, when a glass-plate (refractive index 1.5) of thickness t is introduced in the path of one of the interfering beams (wavelength λ), the intensity at the position where the central maximum occurred previously remains unchanged. The minimum thickness of the glass-plate is
View SolutionIn the ideal double-slit experiment, when a glass-plate (refractive index 1.5) of thickness t is introduced in the path of one of the interfering beams (wavelength λ), the intensity at the position where the central maximum occurred previously remains unchanged. The minimum thickness of the glass-plate is
View Solution
