A ray incident on the droplet of water at an angle of incidence i undergoes two reflections (not total) and emerges. If the deviation suffered by the ray within the drop is minimum and the refractive index of the droplet be μ, then show that cosi=√(u2−1)8.
The correct Answer is:A
To solve the problem, we will analyze the situation step-by-step and derive the required relationship for the angle of incidence i in terms of the refractive index μ.
Step 1: Understanding the Geometry of Refraction and Reflection
When a ray of light enters a water droplet, it refracts at the surface, undergoes two reflections within the droplet, and then refracts again as it exits.
Step 2: Define Angles
Let:
- i = angle of incidence
- r = angle of refraction
According to Snell's law:
sini=μsinr
Step 3: Calculate Total Deviation
The total deviation D of the ray can be expressed as:
D=(deviation due to refraction)+(deviation due to reflection)
The deviation due to refraction at the entry is i−r and at the exit is also i−r. The deviation due to reflection is 180∘−2r for each reflection. Thus, the total deviation becomes:
D=2(i−r)+2(180∘−2r)=2(i−r)+360∘−4r
Simplifying this gives:
D=2i−6r+360∘
Step 4: Minimize the Deviation
To find the angle i that minimizes the deviation D, we differentiate D with respect to i and set the derivative to zero:
dDdi=2−6drdi=0
From this, we find:
drdi=13
Step 5: Applying Snell's Law
Using Snell's law at the point of incidence:
sini=μsinr
Differentiating both sides with respect to i:
cosi=μcosrdrdi
Substituting drdi=13:
cosi=μ3cosr
Step 6: Relating Angles Using Trigonometric Identities
Using the identity sin2r+cos2r=1, we can express cosr in terms of sinr:
cosr=√1−sin2r
From Snell's law, we have:
sinr=siniμ
Substituting this into the expression for cosr:
cosr=√1−(siniμ)2
Step 7: Substitute and Rearrange
Substituting cosr back into our equation:
cosi=μ3√1−(siniμ)2
Squaring both sides:
cos2i=μ29(1−sin2iμ2)
This simplifies to:
9cos2i=μ2−sin2i
Using sin2i=1−cos2i:
9cos2i+cos2i=μ2
Thus:
10cos2i=μ2
Rearranging gives:
cos2i=μ2−18
Taking the square root:
cosi=√μ2−18
Final Result
Thus, we have shown that:
cosi=√μ2−18
When a ray of light enters a water droplet, it refracts at the surface, undergoes two reflections within the droplet, and then refracts again as it exits.
Let:
- i = angle of incidence
- r = angle of refraction
sini=μsinr
The total deviation D of the ray can be expressed as:
D=(deviation due to refraction)+(deviation due to reflection)
The deviation due to refraction at the entry is i−r and at the exit is also i−r. The deviation due to reflection is 180∘−2r for each reflection. Thus, the total deviation becomes:
D=2(i−r)+2(180∘−2r)=2(i−r)+360∘−4r
Simplifying this gives:
D=2i−6r+360∘
To find the angle i that minimizes the deviation D, we differentiate D with respect to i and set the derivative to zero:
dDdi=2−6drdi=0
From this, we find:
drdi=13
Using Snell's law at the point of incidence:
sini=μsinr
Differentiating both sides with respect to i:
cosi=μcosrdrdi
Substituting drdi=13:
cosi=μ3cosr
Using the identity sin2r+cos2r=1, we can express cosr in terms of sinr:
cosr=√1−sin2r
From Snell's law, we have:
sinr=siniμ
Substituting this into the expression for cosr:
cosr=√1−(siniμ)2
Substituting cosr back into our equation:
cosi=μ3√1−(siniμ)2
Squaring both sides:
cos2i=μ29(1−sin2iμ2)
This simplifies to:
9cos2i=μ2−sin2i
Using sin2i=1−cos2i:
9cos2i+cos2i=μ2
Thus:
10cos2i=μ2
Rearranging gives:
cos2i=μ2−18
Taking the square root:
cosi=√μ2−18
Thus, we have shown that:
cosi=√μ2−18
Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Level 2 Comprehension Based
3 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
- SEMICONDUCTORSBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Subjective
12 Videos
Similar Questions
A ray incident on a droplet of water from air at an angle of incidence 30∘ undergoes two reflections and emerges. If the deviation suffered by the ray is minimum then the requried refractive index of the drop μ=√x. Find the value of x.
View Solution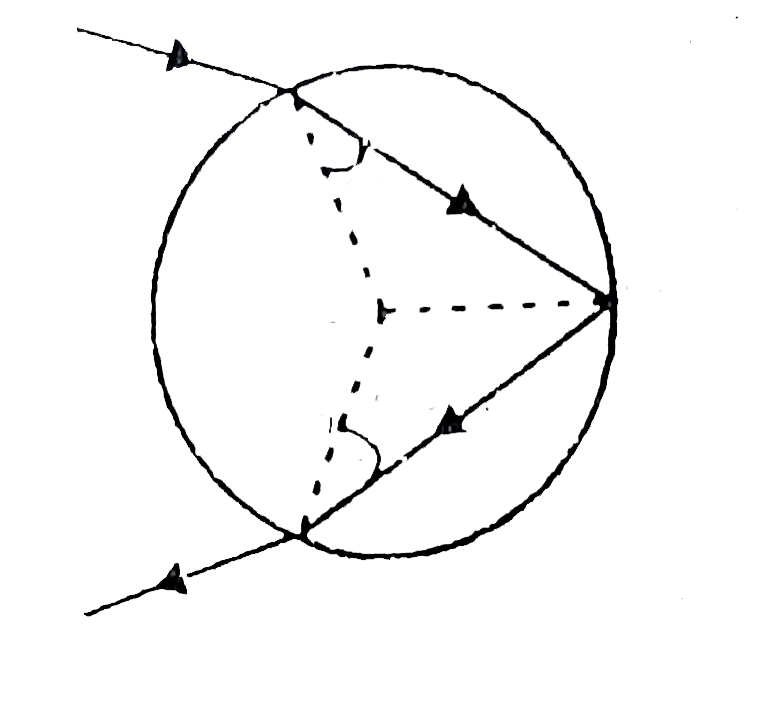
A ray is incident on a sphere of refractive index √2 as shown in figure. Angle of refraction of the ray inside sphere is 30∘ if total deviation suffered by the ray is 30x0, then find x.
View SolutionA light ray is incident on a glass sphere at an angle of incidence 60∘ as shown. Find the angles r,r1,e and the total deviation after two refractions.

View SolutionA ray of light incident at an angle of incidence 48∘ on a prism of refracting angle 60∘ suffers minimum deviation. Calculate the angle of minimum deviation.
View SolutionA light ray is incident on a transparent slab of refractive index μ=√2 at an angle of incidence π/4. Find the ratio of the lateral displacement suffered by the light ray to the maximum value which it could have suffered.
View SolutionA ray of light is incident on a rectangular plate at an angle of incidence 60∘. The light ray suffers a deviation which is 25% of the angle of incidence. The refractive index of the glass will be
View SolutionFor total internal reflection to take place, the angle of incidence i and the refractive index μ of the medium must satisfy the inequality
View SolutionA ray of light is incident normally on one of the faces of a prism of apex angle 30 degree and refractive index √2. The angle of deviation of the ray in degrees is
View SolutionA ray is ihncident at an angle of incidence ii on one surface of a prism of small angle A and emerge normally from opposite surface. If the refractive index of the material of prism is μ. the angel of incidance I is nearly equal to
View SolutionUnpolarized light is incident on a plane surface of glass of refractive index μ at angle i. If the reflected light gets totally polarized, write the relation between the angle i and refractive index μ.
View Solution
