A glass full of water upto a height of 10 cm has a bottom of are 10cm2, top of area 30cm2 and volume 1 litre.
(a) Find the force exerted by the water on the bottom.
(b) Find the resultant force exerted by the sides of the glass on the water.
(c ) If the glass is convered by a jar and the air inside the jar is completely pumped out, what will be the answer to parts (a) and (b).
(d) If a glass of different shape is used, provided the height, the bottom area, the top area and the volume are unchanged, will the answer to parts (a) and (b) change.
Take g=10m/s3, density of water=103kg/m3 and atmospheric pressure =1.01×105N/m2.
The correct Answer is:A, B, C, D
(a) Force exerted by the water on the bottom
F1=(p0+ρgh)A1 …(i)
Here, p0=atmospheric pressure =1.01×105N/m2
ρ=density of water =103k/gm3
g=10m/s2,h=10cm=0.1m
and A1= area of base =10cm2=10−3m2
Subsituting in Eq. (i) and we get,
F1=(1.01×105+103×10×0.1)×10−3
or, F1=102N (downwards)
(b) Force exerted by atmosphere on water
F2=(p0)A2
Here,A2= area of top =30cm2=3×10−3m2
=303N (downwards).
Force exerted by bottom on the water
F3=−F1 or F3=102N(upwards)
weight of water W=(volume)(density)(g)=103103(10)
=10N (downwards)
LetF be the force= net downward froce
or, F+F3=F2+W
∴F=F2+W−F3=303+10−102
or,F=211N (upwards)
(c ) If the air inside the jar is completely pumped out,
F1=(ρgh)A1(asp0=0)
=103(10)(0.1)103=1N (downwards)
In this case,
F2=0
and F3=1N (upwards)
∴F=F2+W−F3=0+10−1=9N (upwards)
(d) No, the answer will remain the same. Because the answer depend upon p0ρ,g,h,A1 and A2.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise16.1
6 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Introductory Exercise
1 Videos
- FLUID MECHANICSBOOK - DC PANDEY ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Solved Examples
21 Videos
- EXPERIMENTSBOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A curved glass vessel full of water upto a height of 10 cm has a bottom of area 10cm2, top of area 30cm2 and volume 1 L.
(i) Find the force exerted by the water on the bottom.
(ii) Find the resultant force exerted by the sides of the glass on the water.
(iii) If the glass vessel is covered by a jar and the air inside the jar is completely pumped out, what will be the answers to parts (a) and (b)
(iv) If a glass vessel of different shape is used provided the height, the bottom area and the volume are unchanged, will the answers to parts (a) and (b) change.
(Take, g=10ms−2 , density of water =103kgm−2 and atmospheric pressure =1.01×105Nm−2)
View SolutionA glass full of water has a bottom of area 20cm2, top of area 20cm, height 20cm and volume half a litre.
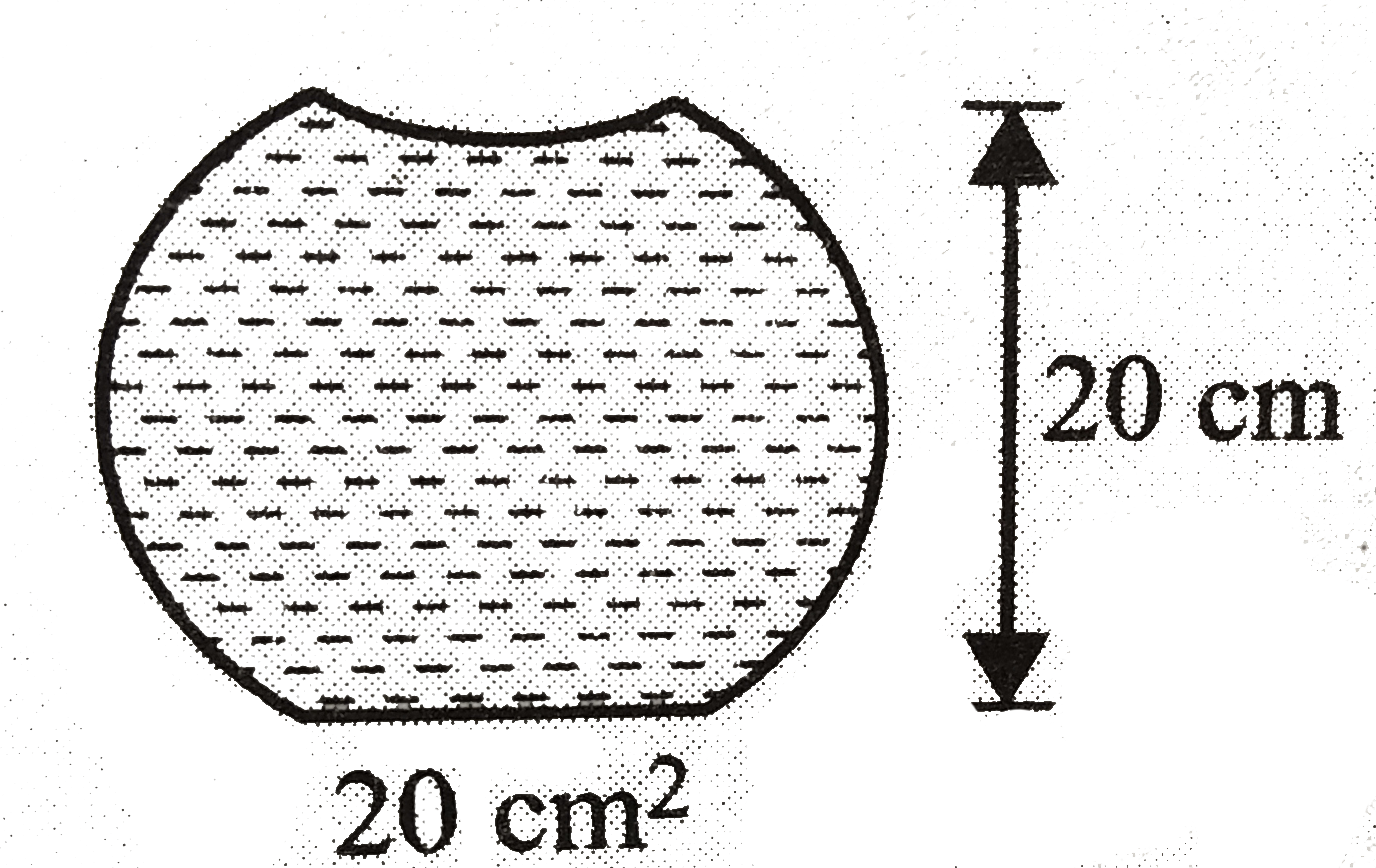
a. Find the force exerted by the water on the bottom.
b. Considering the equilibrium of the v, after. find the resultant force exerted by the side, of the glass on the water. Atmospheric pressure =1.0×105N/m2. Density of water =1000kg/m−3 and g=10m/s2
View SolutionWater is filled in a flask up to a height of 20 cm. The bottom of the flask is circular with radius 10 cm. If the atmospheric pressure is 1.01×105 Pa, find the force exerted by the water on the bottom. Take g=10ms−2 and density of water =1000 kgm^-3.
View SolutionThe neck and bottom of a bottle are 3 cm and 15 cm in radius respectively. If the cork is pressed with a force 12 N in the neck of the bottle, then force exerted on the bottom of the bottle is :-
View SolutionFind the force exerted by the water on a 2m2 plane surface of a large stone placed at the bottom of a sea 500 m deep. Does the force depend on the orientation of the surface? Neglect the size of the stone in comparison to the depth of the sea.
View SolutionRefractive index of glass with respect to water is (9/8).
Refractive index of glass with respect to air is (3/2). find the refractive index of water with respect to air .
View SolutionRefractive index of glass with respect to water is (9/8). Refractive index of glass with respect to air is (3/2). Find the refractive index of water with respect to air
View SolutionA metal piece of mass 160 g lies in equilibrium inside a glass of water as shown in figure. The piece touches the bottom of the glass at a small number of points. If the density of the metal is 8000kgm−3, find the normal fore exerted by the bottom of the glass on the metal piece.

View SolutionA metal piece of mass 160 g lies iln equilibrium inside a glass of water figure. The ppece touches the bottom of the glass at a small number of points. If the density of the metl is 8000 kg m−3, find the normal fore exerted by the bottom of the glass on the metal piece.

View Solution(a) The diameter of neck and bottom of a bottle are 2 cm and 10 cm respectively. The bottle is completely filled with oil. If the cork in the neck is pressed in with a force of 1.2kgf , what force is exerted on the bottom of the bottle ?
(b) Name the law/ principle you have used to find the force in part (a)
View Solution
