With what minmum speed should m be projected from point C in presence of two fixed masses M each at A and B as shows in the figure such that mass m should escape the gravitational of A and B ?
√2GMR
√2√2GMR
2√GMR
2√2√GMR
The correct Answer is:B
To solve the problem of determining the minimum speed v with which mass m should be projected from point C to escape the gravitational influence of two fixed masses M located at points A and B, we can follow these steps:
1. Understanding the System:
- We have two fixed masses M at points A and B.
- The distance between the two masses is 2R.
- The point C is located at the midpoint between A and B, at a distance R from each mass.
2. Initial Energy Calculation:
- The initial kinetic energy (KE) of mass m when projected with speed v is given by:
KE=12mv2
- The initial potential energy (PE) due to the gravitational attraction from both masses M is:
PE=−GMmR−GMmR=−2GMmR
- Therefore, the total initial energy Ei is:
Ei=KE+PE=12mv2−2GMmR
3. Final Energy Calculation:
- For mass m to escape the gravitational influence of M, it must reach a point where the potential energy is zero and the kinetic energy is also zero (at infinity):
Ef=0
4. Applying Conservation of Energy:
- According to the conservation of energy, the initial energy must equal the final energy:
Ei=Ef
12mv2−2GMmR=0
5. Solving for Minimum Speed:
- Rearranging the equation gives:
12mv2=2GMmR
- Dividing both sides by m (assuming m≠0):
12v2=2GMR
- Multiplying both sides by 2:
v2=4GMR
- Taking the square root:
v=√4GMR=2√GMR
Final Answer
The minimum speed v with which mass m should be projected from point C is:
v=2√GMR
- We have two fixed masses M at points A and B.
- The distance between the two masses is 2R.
- The point C is located at the midpoint between A and B, at a distance R from each mass.
- The initial kinetic energy (KE) of mass m when projected with speed v is given by:
KE=12mv2
- The initial potential energy (PE) due to the gravitational attraction from both masses M is:
PE=−GMmR−GMmR=−2GMmR
- Therefore, the total initial energy Ei is:
Ei=KE+PE=12mv2−2GMmR
- For mass m to escape the gravitational influence of M, it must reach a point where the potential energy is zero and the kinetic energy is also zero (at infinity):
Ef=0
- According to the conservation of energy, the initial energy must equal the final energy:
Ei=Ef
12mv2−2GMmR=0
- Rearranging the equation gives:
12mv2=2GMmR
- Dividing both sides by m (assuming m≠0):
12v2=2GMR
- Multiplying both sides by 2:
v2=4GMR
- Taking the square root:
v=√4GMR=2√GMR
The minimum speed v with which mass m should be projected from point C is:
v=2√GMR
Topper's Solved these Questions
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Level 2 More Than One Correct
10 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Level 2 Subjective
14 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Level 1 Subjective
19 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
Similar Questions
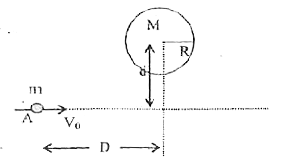
With what speed v0 should a body be projected as shown in the figure, with respect to a planet of mass M so that it would just be able to graze the planet and escape ? The radius of the planet is R. (Assume that the planet is fixed ).
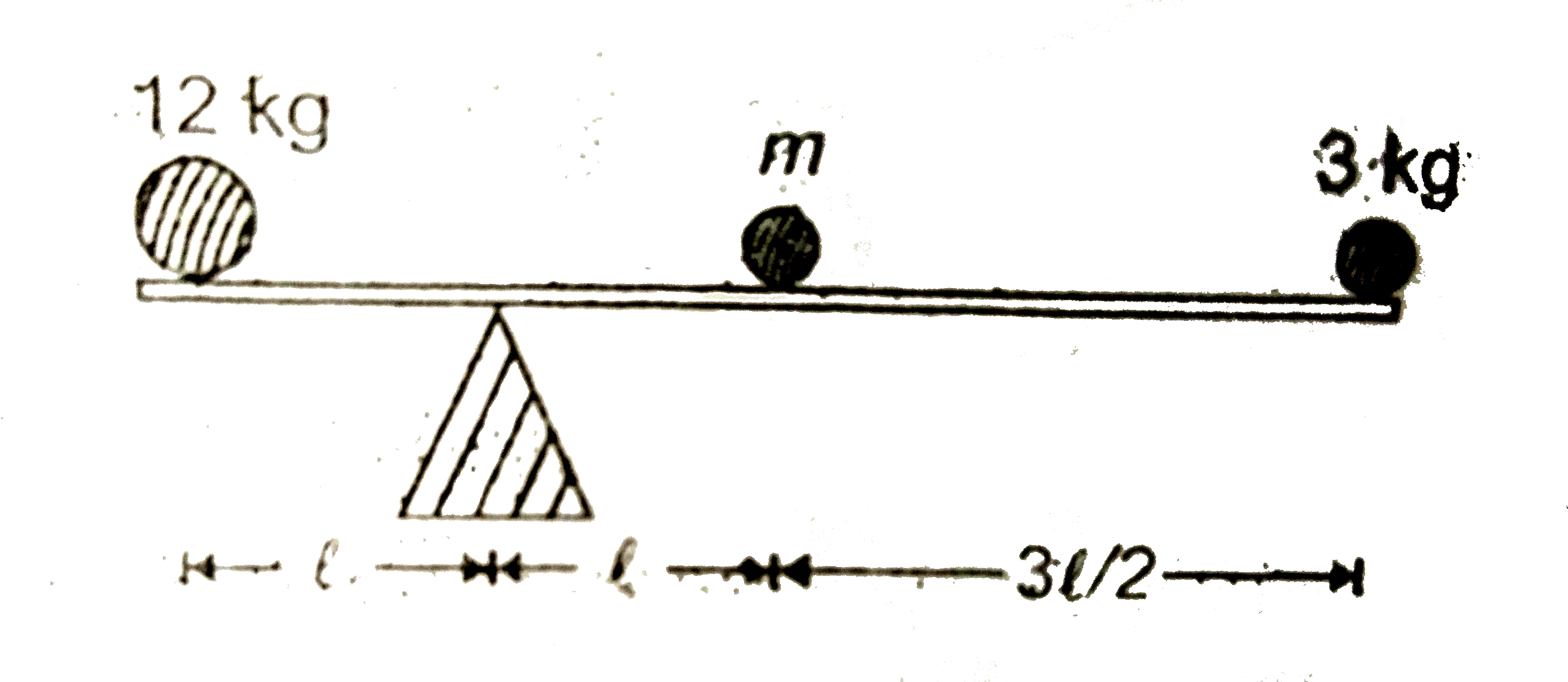
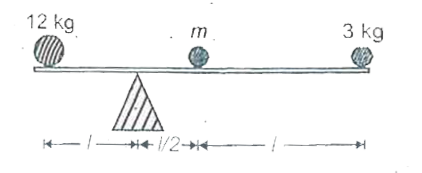
View SolutionFor the equilibrium of the system shown, the value of mass m should be
View SolutionA particle of mass m is moved from A to B as show in figure. Then potential energy of the the particle
View SolutionTwo uniform thin rods each of length L and mass m are joined as shown in the figure. Find the distance of centre of mass the system from point O

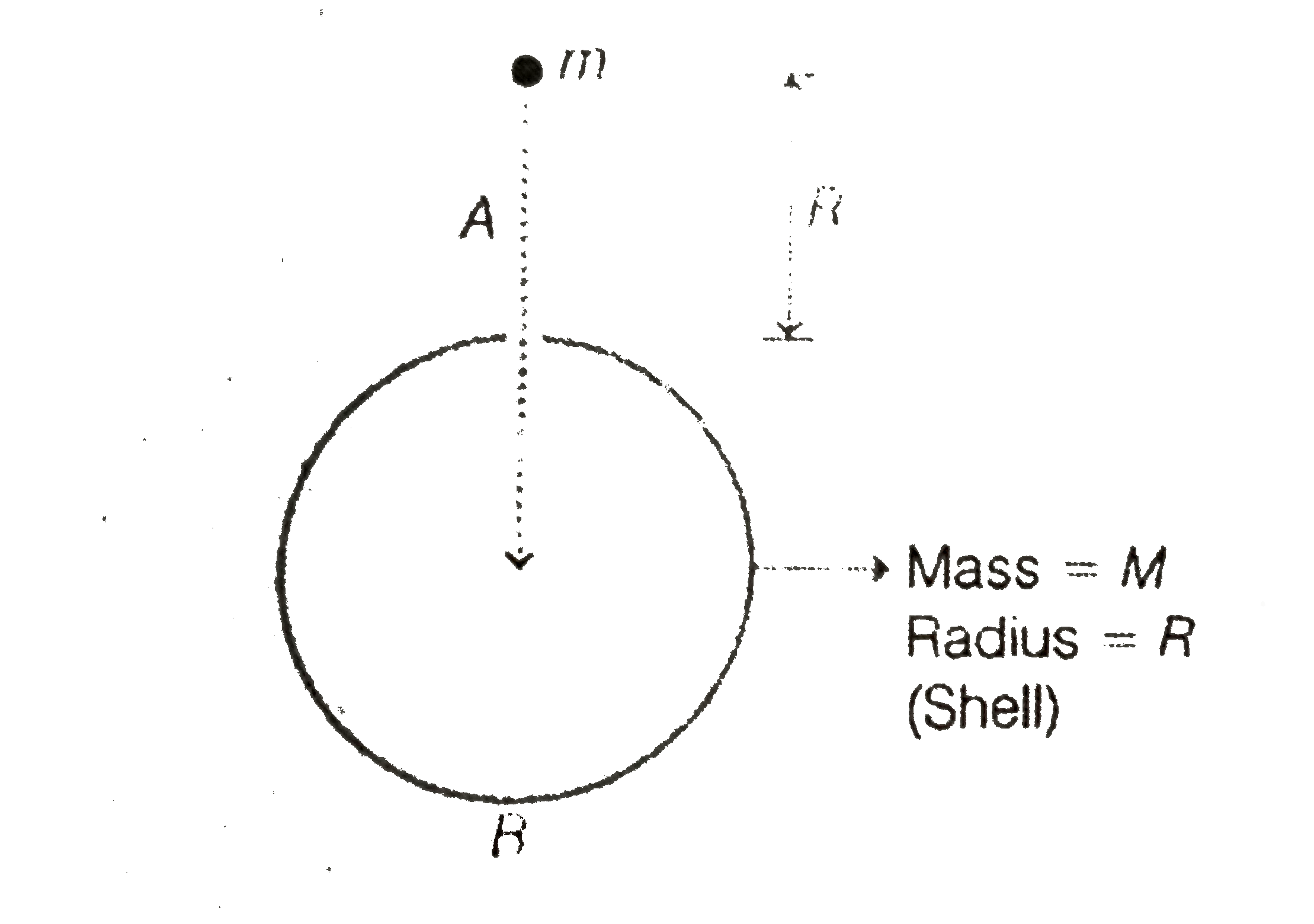
View SolutionFind the potential V at P in the gravitational field of fixed point mass M.
View SolutionTwo point masses m and 2m are kept at points A and B as shown. E represents magnitude of gravitational field strength and V the gravitational potential. As we move from A to B

View SolutionTwo particles are projected with speed 4m/s and 3m/s simultaneously from same point as shown in the figure. Then:

View SolutionFor equilibrium of the system, value of mass m should be
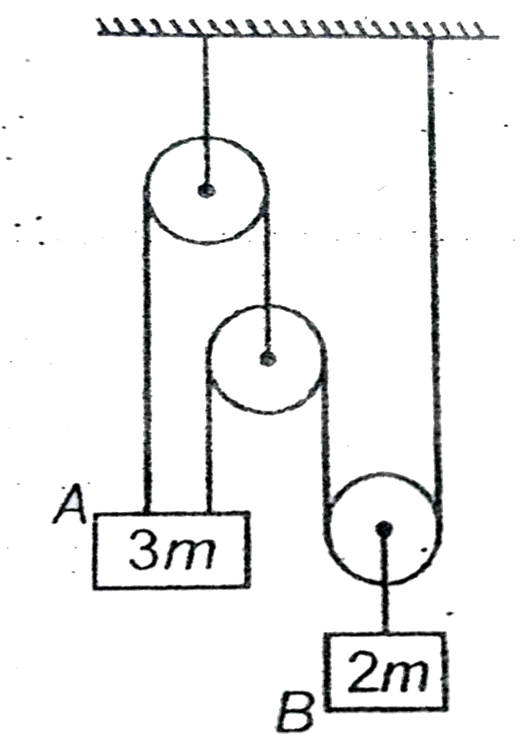
View SolutionConsider the system shown in figure
Mass of block A= 3m ,
Mass of block B= 2m .
Find the acceleration of A and B.
View SolutionA particle is projected from the mid-point of the line joining two fixed particles each of mass m. If the separation between the fixed particles is l, the minimum velocity of projection of the particle so as to escape is equal to
View Solution
