At time t=0, a car moving along a straight line has a velocity of 16ms−1. It slows down with an acceleration of −0.5tms−2, where t is in second. Mark the correct statement (s).
The direction of velocity changes at t=8s
The distance travelled in 4 s is approximately 58.67 m
The distance travelled by the particle in 10 s is 94 m
The speed of particle at t=10 s is 9ms−1
The correct Answer is:A, B, C, D
To solve the problem step by step, we need to analyze the motion of the car given its initial conditions and the acceleration function.
Step 1: Understand the given information
- Initial velocity of the car, v0=16m/s
- Acceleration, a(t)=−0.5tm/s2
Step 2: Relate acceleration to velocity
We know that acceleration is the rate of change of velocity:
a=dvdt
Thus, we can write:
dv=adt=−0.5tdt
Step 3: Integrate to find velocity as a function of time
Integrating both sides:
∫dv=∫−0.5tdt
This gives:
v=−0.25t2+C
Where C is the constant of integration. We know that at t=0, v=16m/s:
16=−0.25(0)2+C⟹C=16
Thus, the velocity function is:
v(t)=16−0.25t2
Step 4: Determine when the velocity becomes zero
To find when the car stops (velocity becomes zero):
0=16−0.25t2
Solving for t:
0.25t2=16⟹t2=64⟹t=8s
So, the direction of the velocity changes at t=8s.
Step 5: Find the distance traveled in 4 seconds
Using the velocity function to find the distance:
s(t)=∫v(t)dt=∫(16−0.25t2)dt
Integrating:
s(t)=16t−0.25t33+C
At t=0, s(0)=0, so C=0:
s(t)=16t−0.25t33
Now, substituting t=4:
s(4)=16(4)−0.25(43)3=64−0.25(64)3=64−163=64−5.33≈58.67m
Step 6: Find the distance traveled in 10 seconds
Substituting t=10:
s(10)=16(10)−0.25(103)3=160−0.25(1000)3=160−2503=160−83.33≈76.67m
Step 7: Calculate the total distance traveled until t=10
The distance traveled from t=0 to t=8 is s(8):
s(8)=16(8)−0.25(83)3=128−0.25(512)3=128−1283=128−42.67≈85.33m
After t=8, the car is moving backward. The distance from t=8 to t=10 is:
\text{Distance from } t = 8 \text{ to } t = 10 = s(8) - s(10) = 85.33 - 76.67 = 8.66 \, \text{m
Thus, the total distance traveled in 10 seconds is:
D=85.33+8.66≈94m
Step 8: Find the speed at t=10
Using the velocity function:
v(10)=16−0.25(102)=16−25=−9m/s
The speed (magnitude of velocity) is:
Speed=9m/s
Conclusion
The correct statements are:
- The direction of velocity changes at t=8s.
- The distance traveled by the particle in 10 seconds is 94m.
- The distance traveled in 4 seconds is approximately 58.67m.
- The speed of the particle at t=10s is 9m/s.
- Initial velocity of the car, v0=16m/s
- Acceleration, a(t)=−0.5tm/s2
We know that acceleration is the rate of change of velocity:
a=dvdt
Thus, we can write:
dv=adt=−0.5tdt
Integrating both sides:
∫dv=∫−0.5tdt
This gives:
v=−0.25t2+C
Where C is the constant of integration. We know that at t=0, v=16m/s:
16=−0.25(0)2+C⟹C=16
Thus, the velocity function is:
v(t)=16−0.25t2
To find when the car stops (velocity becomes zero):
0=16−0.25t2
Solving for t:
0.25t2=16⟹t2=64⟹t=8s
So, the direction of the velocity changes at t=8s.
Using the velocity function to find the distance:
s(t)=∫v(t)dt=∫(16−0.25t2)dt
Integrating:
s(t)=16t−0.25t33+C
At t=0, s(0)=0, so C=0:
s(t)=16t−0.25t33
Now, substituting t=4:
s(4)=16(4)−0.25(43)3=64−0.25(64)3=64−163=64−5.33≈58.67m
Substituting t=10:
s(10)=16(10)−0.25(103)3=160−0.25(1000)3=160−2503=160−83.33≈76.67m
The distance traveled from t=0 to t=8 is s(8):
s(8)=16(8)−0.25(83)3=128−0.25(512)3=128−1283=128−42.67≈85.33m
After t=8, the car is moving backward. The distance from t=8 to t=10 is:
\text{Distance from } t = 8 \text{ to } t = 10 = s(8) - s(10) = 85.33 - 76.67 = 8.66 \, \text{m
Thus, the total distance traveled in 10 seconds is:
D=85.33+8.66≈94m
Using the velocity function:
v(10)=16−0.25(102)=16−25=−9m/s
The speed (magnitude of velocity) is:
Speed=9m/s
The correct statements are:
- The direction of velocity changes at t=8s.
- The distance traveled by the particle in 10 seconds is 94m.
- The distance traveled in 4 seconds is approximately 58.67m.
- The speed of the particle at t=10s is 9m/s.
Topper's Solved these Questions
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - More Than One Correct
6 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Comprehension
7 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Objective
45 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
Similar Questions
A particle moving in a straight line has its velocit varying with time according to relation v=t2−6t+8(m/s) where t is in seconds. The CORRECT statement(s) about motion of this particle is/are:-
View SolutionAcceleration time graph of a particle moving along a straight line is given. Then average acceleration between t=0 & t=4 is :−
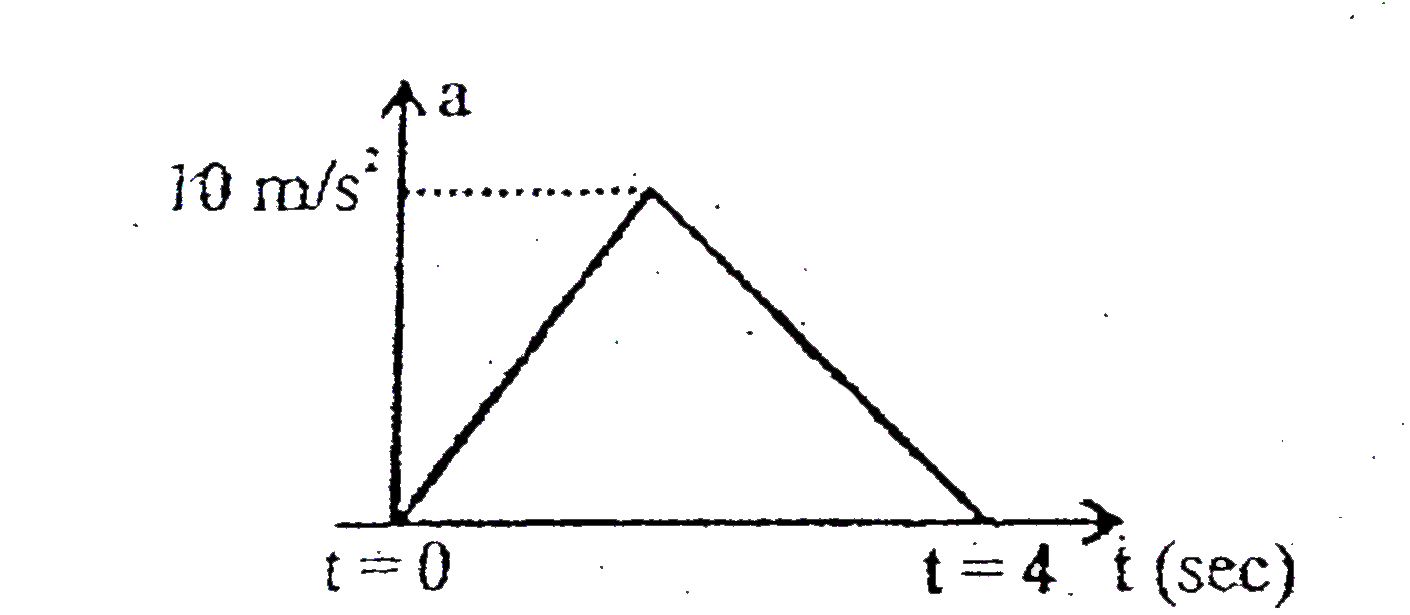
View SolutionA particle moves along a straight line its velocity dipends on time as v=4t−t2. Then for first 5s:
View SolutionAt time t , the displacement of a particle moving in a straight line by x=−4t2+2t . Find the velocity and acceleration a when t = (1/2) s .
View SolutionA particle moving along straight line has velocity v=μs where s is in the displacement . If s=s0 then which of the following graph best represent s versus t
View SolutionA car moves along a straight line whose equation of motion is given by
s=12t+3t2−2t3
where s is in metres and t is in seconds. The velocity of the car at start will be :-
View SolutionParticle A is moving along X-axis. At time t = 0, it has velocity of 10ms−1 and acceleration −4ms−2. Particle B has velocity of 20ms−1 and acceleration −2ms−2. Initially both the particles are at origion. At time t = 2 distance between the particles are at origin. At time t = 2 s distance between the particles is
View SolutionA particle is moving along a circle and its linear velocity is 0.5ms when its angular velocity is 2.5rads−1. Its tangential acceleration is 0.2ms−2 then its angular acceleration is
View SolutionA particle is moving on a straight line. Its velocity at time t is (8−2t)m/s. What is the total distance covered from t=0 to t=6s?
View SolutionThe velocity v of a body moving along a straight line varies with time t as v=2t2e−t, where v is in m/s and t is in second. The acceleration of body is zero at t =
View Solution
