At time t , the displacement of a particle moving in a straight line by x=−4t2+2t . Find the velocity and acceleration a when t = (1/2) s .
To solve the problem step by step, we will find the velocity and acceleration of the particle at time t=12 seconds, given the displacement function x(t)=−4t2+2t.
Step 1: Find the Velocity
The velocity v of a particle is defined as the rate of change of displacement with respect to time. Mathematically, it is given by:
v=dxdt
Given the displacement function:
x(t)=−4t2+2t
we will differentiate x(t) with respect to t.
Differentiation:
dxdt=ddt(−4t2+2t)
Using the power rule of differentiation:
dxdt=−4⋅2t2−1+2⋅1t1−1=−8t+2
Thus, the velocity function is:
v(t)=−8t+2
Step 2: Calculate Velocity at t=12 seconds
Now we substitute t=12 into the velocity function:
v(12)=−8(12)+2
Calculating this gives:
v(12)=−4+2=−2m/s
Step 3: Find the Acceleration
The acceleration a of a particle is defined as the rate of change of velocity with respect to time. Mathematically, it is given by:
a=dvdt
We have already found the velocity function:
v(t)=−8t+2
Now we will differentiate v(t) with respect to t.
Differentiation:
dvdt=ddt(−8t+2)
Using the power rule of differentiation:
dvdt=−8
Thus, the acceleration function is:
a(t)=−8m/s2
Step 4: Calculate Acceleration at t=12 seconds
Since the acceleration is constant:
a(12)=−8m/s2
Final Answers
- The velocity at t=12 seconds is −2m/s.
- The acceleration at t=12 seconds is −8m/s2.
The velocity v of a particle is defined as the rate of change of displacement with respect to time. Mathematically, it is given by:
v=dxdt
Given the displacement function:
x(t)=−4t2+2t
we will differentiate x(t) with respect to t.
dxdt=ddt(−4t2+2t)
Using the power rule of differentiation:
dxdt=−4⋅2t2−1+2⋅1t1−1=−8t+2
Thus, the velocity function is:
v(t)=−8t+2
Now we substitute t=12 into the velocity function:
v(12)=−8(12)+2
Calculating this gives:
v(12)=−4+2=−2m/s
The acceleration a of a particle is defined as the rate of change of velocity with respect to time. Mathematically, it is given by:
a=dvdt
We have already found the velocity function:
v(t)=−8t+2
Now we will differentiate v(t) with respect to t.
dvdt=ddt(−8t+2)
Using the power rule of differentiation:
dvdt=−8
Thus, the acceleration function is:
a(t)=−8m/s2
Since the acceleration is constant:
a(12)=−8m/s2
- The velocity at t=12 seconds is −2m/s.
- The acceleration at t=12 seconds is −8m/s2.
Topper's Solved these Questions
- VECTORS SCALARS ELEMENTARY CALCULUS BOOK - ICSECHAPTER - VECTORS SCALARS ELEMENTARY CALCULUS EXERCISE - FROM SCALAR PRODUCT AND VECTOR PRODUCT
20 Videos
- UNITSBOOK - ICSECHAPTER - UNITSEXERCISE - MODULE 3 (SELECTED PROBLEMS)
38 Videos
- WAVESBOOK - ICSECHAPTER - WAVESEXERCISE - From Musical Sound
10 Videos
Similar Questions
If the displacement of a particle is givne by s=(12t2+4√t)m. Find the velocity and acceleration at t = 4 seconds.
View SolutionThe displacement of a particle along the x-axis is given by x=3+8t+7t2. Obtain its velocity and acceleration at t=2s.
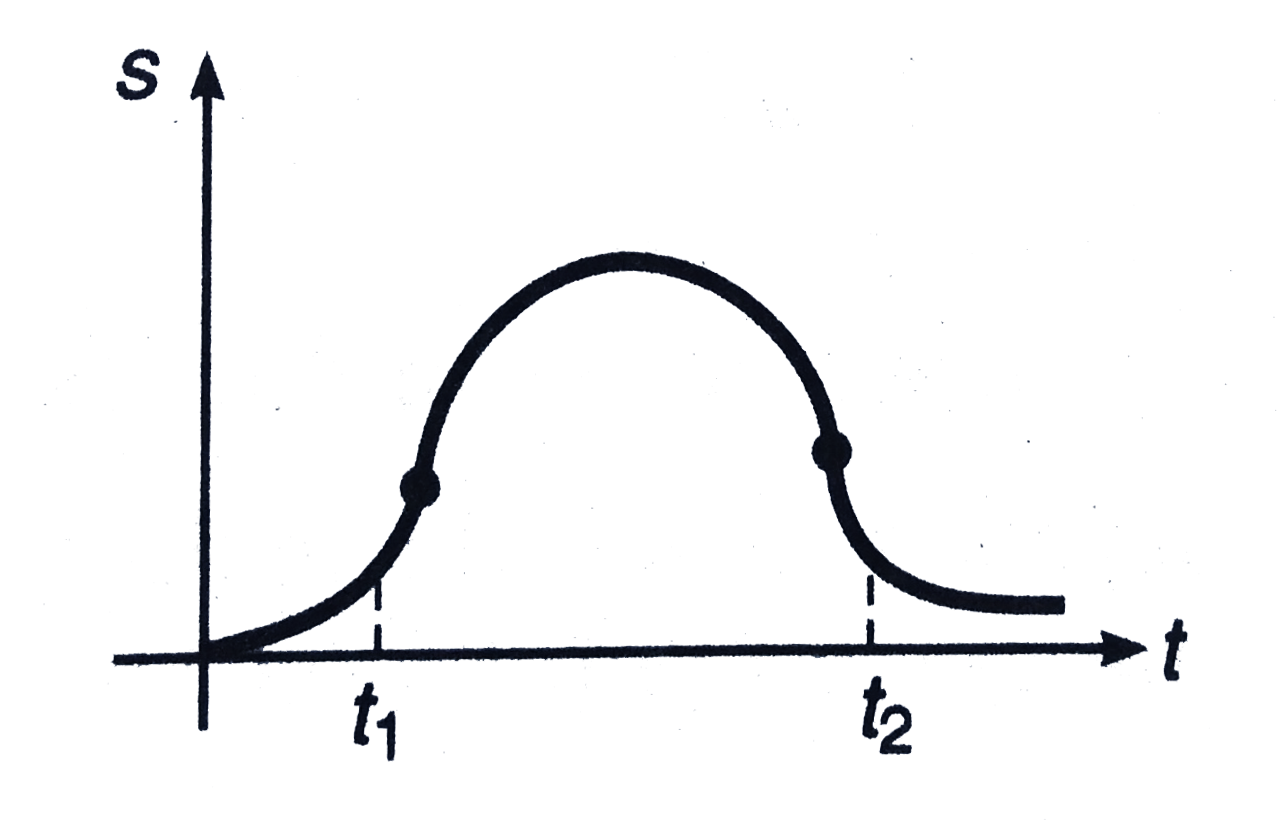
View SolutionFigure shows the displacement-time graph of a particle moving in a straight line. Find the signs of velocity and acceleration of particle at time t=t1andt=t2.
View SolutionThe displacement x of a particle moving along x-axis at time t is given by x2=2t2+6t. The velocity at any time t is
View SolutionThe displacement x of a particle in a straight line motion is given by x=1−t−t2. The correct representation of the motion is
View SolutionIf the displacement of a particle varies with time as x=5t2+7t.
Then find its velocity.
View SolutionThe motion of a particle along a straight line is described by the function s=6+4t2−t4 in SI units. Find the velocity, acceleration, at t = 2s, and the average velocity during 3rd second.
View SolutionThe displacement x of a particle at time t along a straight line is given by x=α−βt+γt2. The acceleraion of the particle is
View SolutionThe displacement x of a particle along a straight line at time t is given by x=a0+a1t+a2t2. The acceleration of the particle is
View SolutionThe displacement x of a particle along the x-axis at time t is given by x=a12t+a23t2. Find the acceleration of the particle.
View Solution
