Consider a compound slab consisting of two different material having equal thickness and thermal conductivities K and 2K respectively. The equivalent thermal conductivity of the slab is
23K
√2K
3K
43K
The correct Answer is:D
To find the equivalent thermal conductivity of a compound slab consisting of two different materials with equal thickness and thermal conductivities K and 2K, we can follow these steps:
Step 1: Understand the Configuration
We have two slabs of equal thickness L:
- The first slab has thermal conductivity K.
- The second slab has thermal conductivity 2K.
Step 2: Calculate the Thermal Resistance
The thermal resistance R for each slab can be calculated using the formula:
R=LK⋅A
where L is the thickness of the slab, K is the thermal conductivity, and A is the cross-sectional area.
For the first slab (thermal conductivity K):
R1=LK⋅A
For the second slab (thermal conductivity 2K):
R2=L2K⋅A
Step 3: Combine the Thermal Resistances
Since the two slabs are in series, the total thermal resistance Rtotal is the sum of the individual resistances:
Rtotal=R1+R2
Substituting the values we calculated:
Rtotal=LK⋅A+L2K⋅A
Step 4: Simplify the Expression
To simplify Rtotal:
Rtotal=LK⋅A+L2K⋅A=2L2K⋅A+L2K⋅A=3L2K⋅A
Step 5: Calculate the Equivalent Thermal Conductivity
The equivalent thermal conductivity Keq can be found using the relationship:
Rtotal=LKeq⋅A
Setting the two expressions for Rtotal equal to each other:
3L2K⋅A=LKeq⋅A
Step 6: Solve for Keq
Cancelling L and A from both sides, we get:
32K=1Keq
Taking the reciprocal:
Keq=2K3
Conclusion
Thus, the equivalent thermal conductivity of the slab is:
Keq=4K3
We have two slabs of equal thickness L:
- The first slab has thermal conductivity K.
- The second slab has thermal conductivity 2K.
The thermal resistance R for each slab can be calculated using the formula:
R=LK⋅A
where L is the thickness of the slab, K is the thermal conductivity, and A is the cross-sectional area.
R1=LK⋅A
R2=L2K⋅A
Since the two slabs are in series, the total thermal resistance Rtotal is the sum of the individual resistances:
Rtotal=R1+R2
Substituting the values we calculated:
Rtotal=LK⋅A+L2K⋅A
To simplify Rtotal:
Rtotal=LK⋅A+L2K⋅A=2L2K⋅A+L2K⋅A=3L2K⋅A
The equivalent thermal conductivity Keq can be found using the relationship:
Rtotal=LKeq⋅A
Setting the two expressions for Rtotal equal to each other:
3L2K⋅A=LKeq⋅A
Cancelling L and A from both sides, we get:
32K=1Keq
Taking the reciprocal:
Keq=2K3
Thus, the equivalent thermal conductivity of the slab is:
Keq=4K3
Topper's Solved these Questions
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Higher Order8videos
THERMAL PROPERTIES OF MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Exampler Problems8videos
THERMAL PROPERTIES OF MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Change of State13videos
THERMAL PROPERTIES OF MATTER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - NCERT Exemplar8videos
SYSTEM OF PARTICLES AND ROTATIONAL MOTIONS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - Assertion And Reason15videos
THERMODYNAMICS
Similar Questions
A slab consists of two layers of different materials of the same thickness and having thermal conductivities K1 and K2. The equivalent thermal conductivity of the slab is
View SolutionA composite slab is made of two parallel layers of two different materials of thermal conductivities K1andK2 are of same thickness. Show that the equivalent thermal conductivity of the slab is 2K1K2K1+K2
View Solution
Knowledge Check
- Question 1 - Select One
The temperature of the two outer surfaces of a composite slab, consisting of two materials having coefficients of thermal conductivity K and 2K and thickness x and 4x respectively. Temperatures on the opposite faces of composite slab are T1andT2 where T2>T1, as shown in fig. what is the rate of flow of heat through the slab in a steady state?
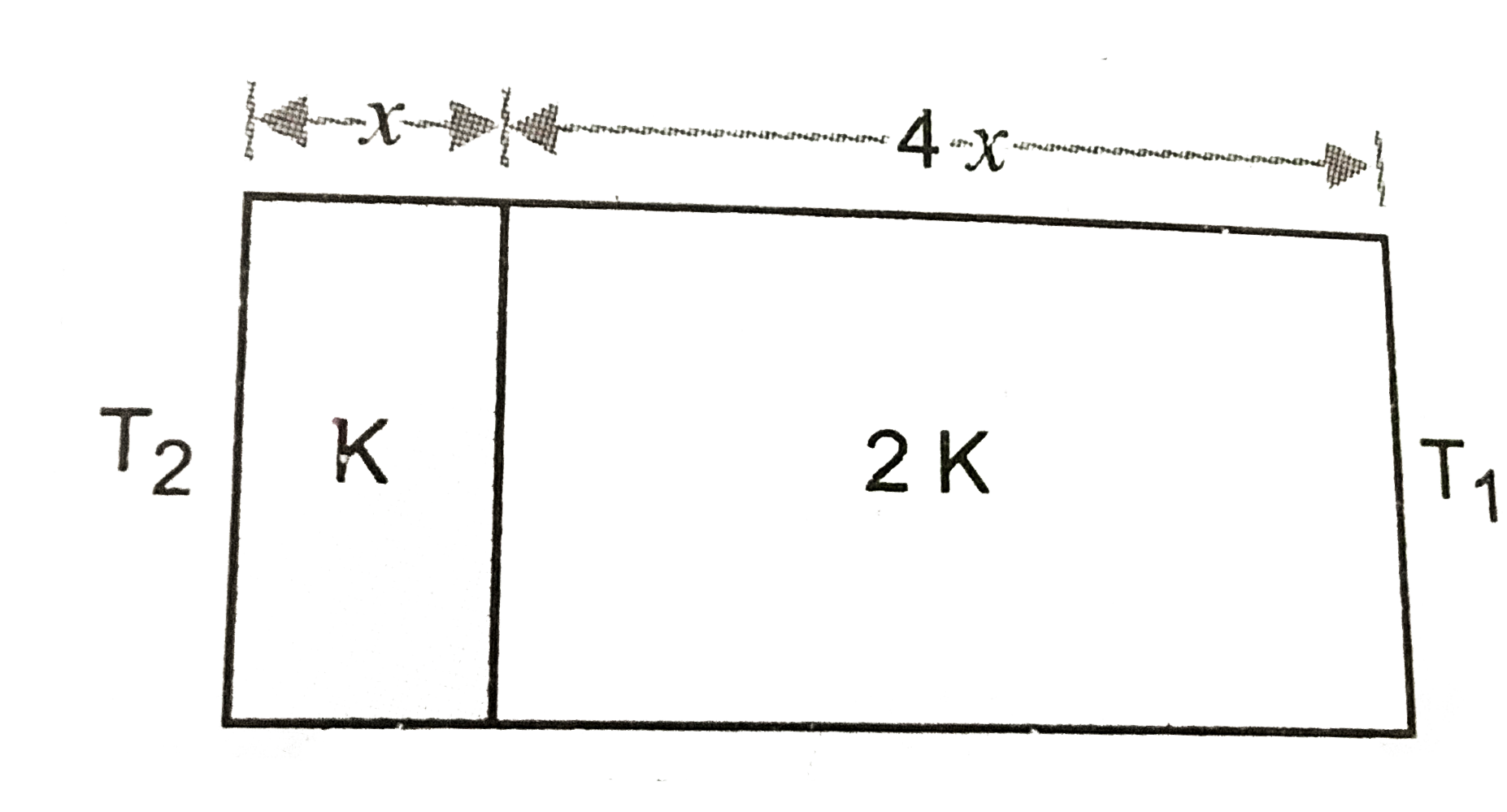 A1B12C23D13
A1B12C23D13 - Question 2 - Select One
In the question number of 45, the equivalent thermal conductivity of the compound bar is
AK1K2K1+K2B2K1K2K1+K2CK1K1+K2DK2K1+K2
Three rods of same dimension are joined in series having thermal conductivity k1 , k2 and k3. The equivalent thermal conductivity is
View SolutionThe temperature of the two outer surfaces of a composite slab consisting of two materials having coefficient of thermal conductivity K and 2K and thickness x and 4x respectively are T2 and T1(T2>T1). The rate of heat transfer through the slab in steady state is (AK(T2−T1)x)f. where, f is equal to
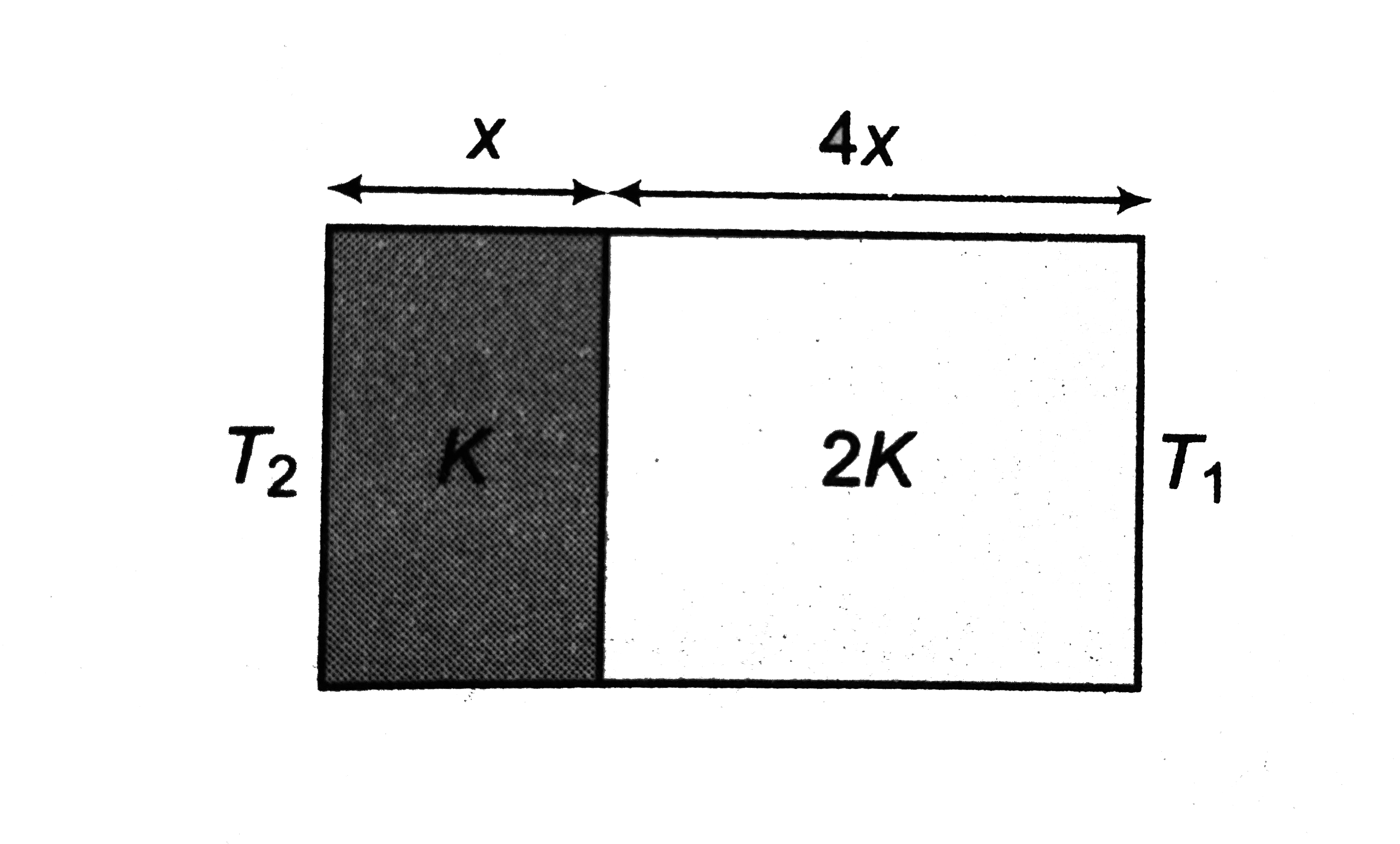 .View Solution
.View Solution
Statement I: Two solid cylindrical rods of identical size and different thermal conductivity K1 and K2 are connected in series. Then the equivalent thermal conductivity of two rods system is less than that value of thermal conductivity of either rod.
Statement II: For two cylindrical rods of identical size and different thermal conductivity K1 and K2 connected in series, the equivalent thermal conductivity K is given by 2K=1K1+1K2View Solution
Statement I: Two solid cylindrical rods of identical size and different thermal conductivity K1 and K2 are connected in series. Then the equivalent thermal conductivity of two rods system is less than that value of thermal conductivity of either rod.
Statement II: For two cylindrical rods of identical size and different thermal conductivity K1 and K2 connected in series, the equivalent thermal conductivity K is given by 2K=1K1+1K2View SolutionTwo rods A and B of different materials are welded together as shown in figure. Their thermal conductivities are K1 and K2. The thermal conductivity of the composite rod will be
 View Solution
View SolutionThe temperature of the two outer surfaces of a composite slab, consisting of two materials having coefficients of thermal conductivity K and 2K and thicknesses x and 4x, respectively are T2 and T1(T2>T1). The rate of heat of heat transfer through the slab, in ? steady state is [(AT2−T1x]f, with f equal to :-
 View Solution
View Solution
