The height of the water in a tank is H. The range of the liquid emerging out from a hole in the wall of the tank at a depth 3H4 from the upper surface of water, will be
H
H2
3H2
√3H2
The correct Answer is:D
To solve the problem step by step, we will follow the reasoning presented in the video transcript.
Step 1: Understand the Problem
We have a tank filled with water to a height H. There is a hole in the wall of the tank at a depth of 3H4 from the top of the water surface. We need to find the range R of the liquid emerging from this hole.
Step 2: Determine the Depth of Water Above the Hole
The depth of water above the hole is:
h=H−3H4=H4
Step 3: Calculate the Velocity of Water Emerging from the Hole
Using Torricelli's theorem, the velocity V of the water emerging from the hole can be calculated using the formula derived from the principle of conservation of energy:
V=√2gh
Substituting h=H4:
V=√2g⋅H4=√gH2
Step 4: Determine the Time of Flight for the Water
The water will fall a vertical distance of H4 before it hits the ground. We can calculate the time t it takes to fall this distance using the equation of motion:
h=12gt2
Substituting h=H4:
H4=12gt2
Rearranging gives:
t2=H2g⇒t=√H2g
Step 5: Calculate the Range of the Water
The range R of the projectile (the water) can be calculated using the formula:
R=V⋅t
Substituting the values of V and t:
R=√gH2⋅√H2g=√gH2⋅H2g=√H24=H2
Final Answer
Thus, the range of the liquid emerging from the hole is:
R=H2
We have a tank filled with water to a height H. There is a hole in the wall of the tank at a depth of 3H4 from the top of the water surface. We need to find the range R of the liquid emerging from this hole.
The depth of water above the hole is:
h=H−3H4=H4
Using Torricelli's theorem, the velocity V of the water emerging from the hole can be calculated using the formula derived from the principle of conservation of energy:
V=√2gh
Substituting h=H4:
V=√2g⋅H4=√gH2
The water will fall a vertical distance of H4 before it hits the ground. We can calculate the time t it takes to fall this distance using the equation of motion:
h=12gt2
Substituting h=H4:
H4=12gt2
Rearranging gives:
t2=H2g⇒t=√H2g
The range R of the projectile (the water) can be calculated using the formula:
R=V⋅t
Substituting the values of V and t:
R=√gH2⋅√H2g=√gH2⋅H2g=√H24=H2
Thus, the range of the liquid emerging from the hole is:
R=H2
Topper's Solved these Questions
Similar Questions
A tank is filled to a height H. The range of water coming out of a hole which is a depth H/4 from the surface of water level is
View SolutionThe level of water in a tank is 5 m high . A hole of the area 10cm2 is made in the bottom of the tank . The rate of leakage of water from the hole is
View Solution
Knowledge Check
A closed rectangular tank contains a certain amount of water. When the tank is placed on its 3 ft. by 4 ft. side, the height of the water in the tank is 5 ft. when the tank is placed on another side of dimension 4 ft. by 5 ft., what is the height, in feet, of the surface of the water above the ground?
A2B3C4D5
Figure shows a large closed cylindrical tank containing water. Initially, the air trapped above the water surface has a height h0 and pressure 2p0 where rh0 is the atmospheric pressure. There is a hole in the wall of the tank at a depth h1 below the top from which water comes out. A long vertical tube is connected as shown.
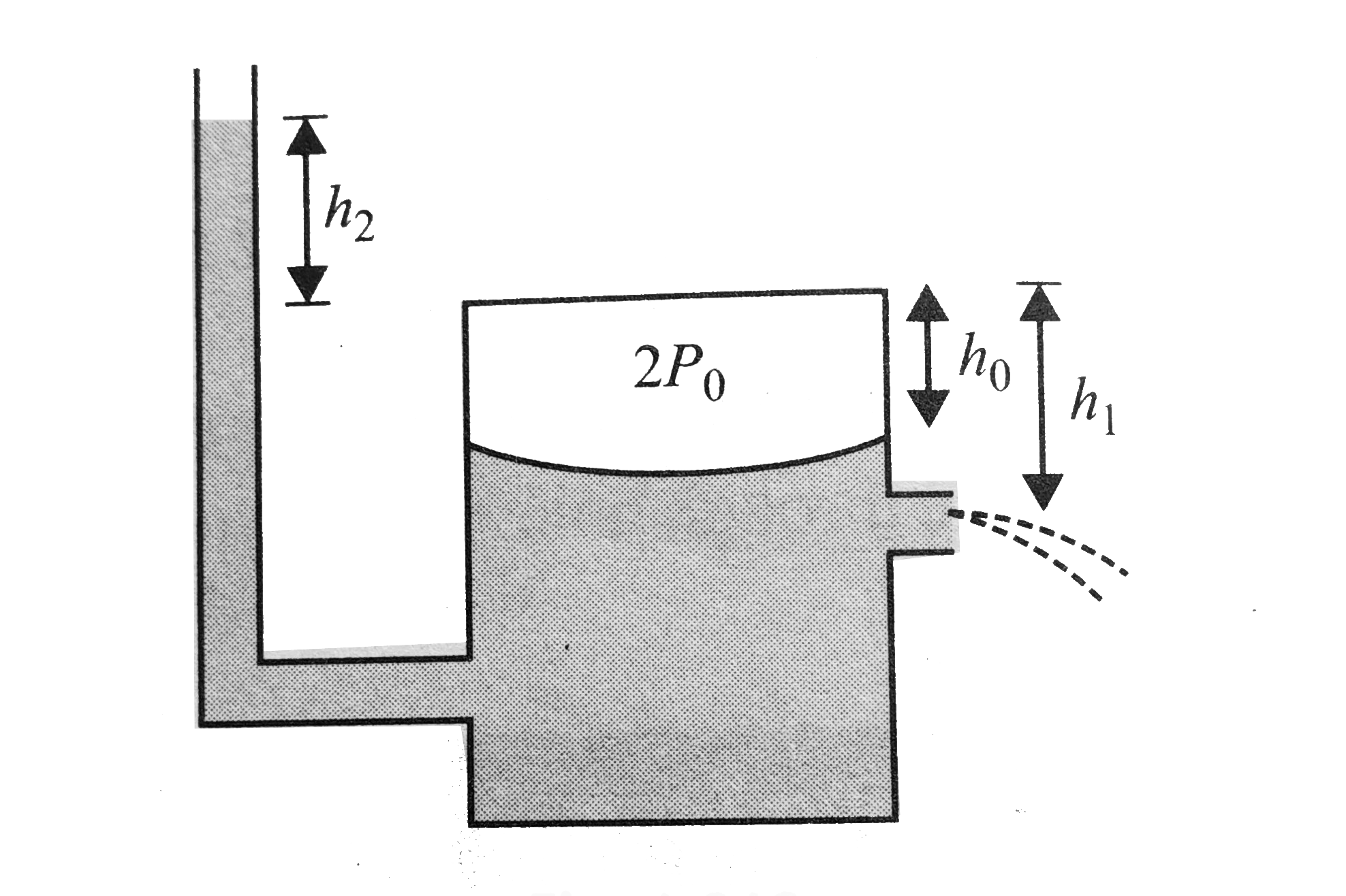
Find the height of the water in the long tube above the top when the water stops coming out of the hole.
View SolutionFigure shows a large closed cylindrical tank containing water. Initially, the air trapped above the water surface has a height h0 and pressure 2p0 where rh0 is the atmospheric pressure. There is a hole in the wall of the tank at a depth h1 below the top from which water comes out. A long vertical tube is connected as shown.
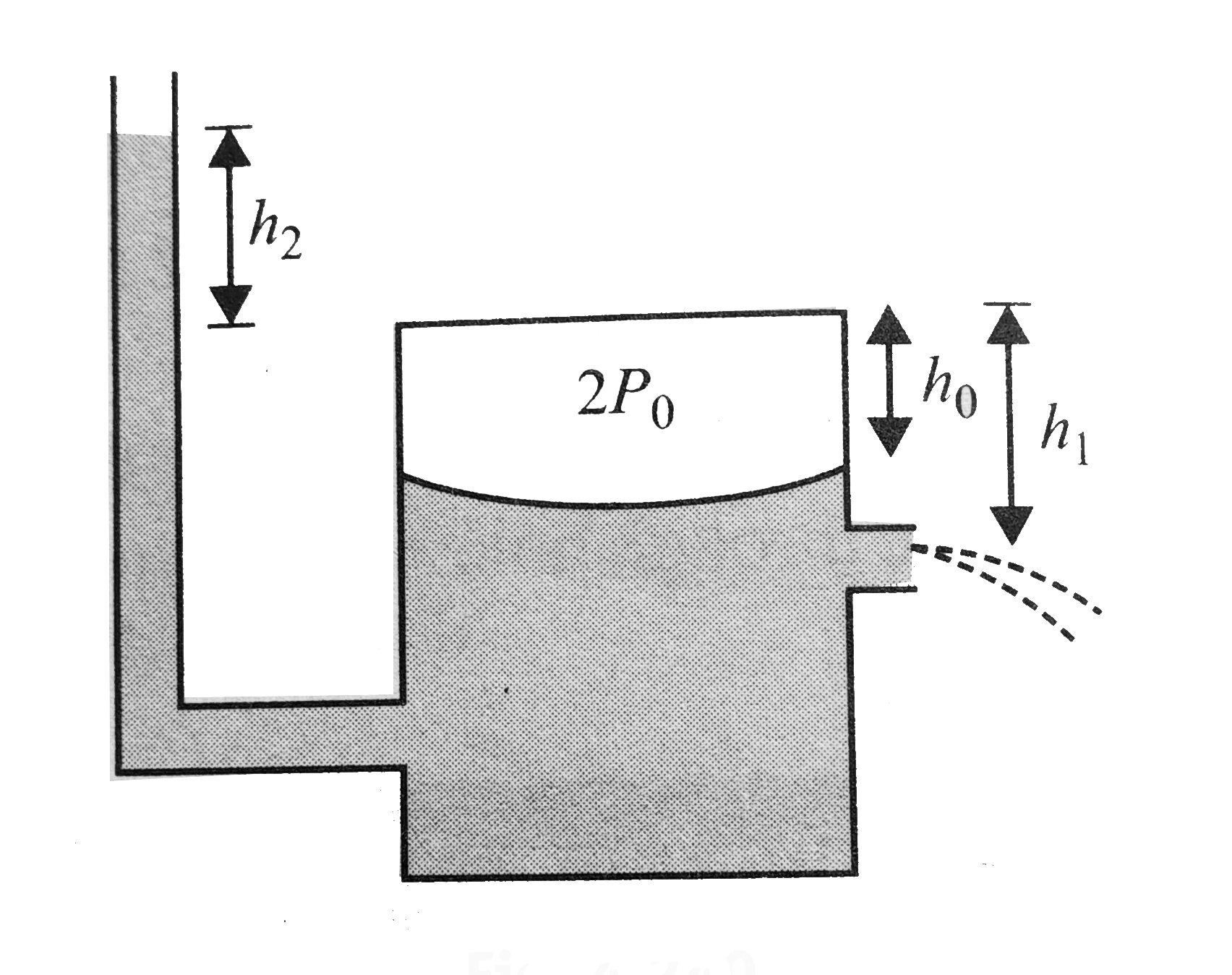
Find the speed with which water comes out of the hole
View SolutionFigure shows a large closed cylindrical tank containing water. Initially, the air trapped above the water surface has a height h0 and pressure 2p0 where rh0 is the atmospheric pressure. There is a hole in the wall of the tank at a depth h1 below the top from which water comes out. A long vertical tube is connected as shown.

Find the height h2 of the water in the long tube above top initially.
View SolutionFigure shows a large closed cylindrical tank containing water, Initially the air trapped above the water surface has a height h0and pressure 2p0 where p0is the atmospheric pressure. There is a hole in the wall of tank at a depth h1below the top from which water comes out. A long vertical tube is connected as shown. (a) Find the height h2 of the water in the long tube above the top initially. (b) Find the speed with which water comes out of hole.(c)Find the height of the water in the long tube above the top when the water stops coming out of the hole.

View SolutionSuppose that water is emptied from a spherical tank of radius 10 cm. If the depth of the water in the tank is 4 cm and is decreasing at the rate of 2 cm/sec, then the radius of the top surface of water is decreasing at the rate of
View SolutionA tank of height H and base area A is half filled with water and there is a small orifice at the bottom and there is a heavy solid cylinder having base area A3 and height of the cylinder is same as that of the tak. The water is flowing out of the orifice. Here cylinder is put into the tank to increase the speed of water flowing out.
After long time, when the height of water inside the tank again becomes equal to H2, the solid cylinder is taken out. then the velocity of liquid flowing out of the orifice (just after removing the cylinder) will be
View SolutionA hollow sphere has a small hole in it. On lowering the sphere in a tank of water, it is observed that water enters into the hollow sphere at a depth of 40 cm below the surface. Surface tension of water is 7×10−2N/m. The diameter of the hole is approximately :
View Solution