If stress-strain relation for volametric change is in the from ΔVV0=KP where P is applied uniform pressure, then K stands for
shear modulus
compressibility
Young's modulus
bulk modulus
The correct Answer is:B
To solve the question regarding the stress-strain relation for volumetric change given by the equation ΔVV0=KP, we need to identify what the constant K represents among the options provided: shear modulus, compressibility, Young's modulus, and bulk modulus.
1. Understanding the Equation:
The equation ΔVV0=KP describes the relationship between the change in volume (ΔV), the original volume (V0), and the applied uniform pressure (P). Here, K is a proportionality constant.
2. Identifying the Options:
- Shear Modulus: This is defined as the ratio of shear stress to shear strain. It is not applicable here since the equation involves volumetric change, not shear.
- Young's Modulus: This is defined as the ratio of tensile stress to tensile strain. Again, this is not relevant to volumetric changes.
- Bulk Modulus: This is defined as the measure of a substance's resistance to uniform compression. It relates to volumetric strain and is defined as B=−PΔVV0.
- Compressibility: This is defined as the reciprocal of bulk modulus, given by β=1B. It is also related to how much a material compresses under pressure.
3. Relating K to Bulk Modulus:
From the definition of bulk modulus, we can rearrange it to find a relationship with compressibility:
B=−PΔVV0⟹ΔVV0=−PB
If we compare this with our original equation ΔVV0=KP, we can see that K must be related to compressibility.
4. Conclusion:
Since K is proportional to the change in volume per unit pressure, and recognizing that compressibility is defined as the change in volume per unit pressure, we conclude that K represents compressibility.
Final Answer:
Thus, K stands for compressibility.
---
The equation ΔVV0=KP describes the relationship between the change in volume (ΔV), the original volume (V0), and the applied uniform pressure (P). Here, K is a proportionality constant.
- Shear Modulus: This is defined as the ratio of shear stress to shear strain. It is not applicable here since the equation involves volumetric change, not shear.
- Young's Modulus: This is defined as the ratio of tensile stress to tensile strain. Again, this is not relevant to volumetric changes.
- Bulk Modulus: This is defined as the measure of a substance's resistance to uniform compression. It relates to volumetric strain and is defined as B=−PΔVV0.
- Compressibility: This is defined as the reciprocal of bulk modulus, given by β=1B. It is also related to how much a material compresses under pressure.
From the definition of bulk modulus, we can rearrange it to find a relationship with compressibility:
B=−PΔVV0⟹ΔVV0=−PB
If we compare this with our original equation ΔVV0=KP, we can see that K must be related to compressibility.
Since K is proportional to the change in volume per unit pressure, and recognizing that compressibility is defined as the change in volume per unit pressure, we conclude that K represents compressibility.
Thus, K stands for compressibility.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - APPLICATIONS OF ELASTIC BEHAVIOUR OF MATERIALS
8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - HOTS
8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - STRESS-STRAIN CURVE
5 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - NCERT Exemplar
5 Videos
- MOTION IN A PLANE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOTION IN A PLANE EXERCISE - Assertion And Reason
15 Videos
Similar Questions
An ideal gas (CpCv=γ)has initial volume V0 is kept in a vessel. It undergoes a change and follows the following relation P=kV2 (where P is pressure, and V is volume) find the change in internal energy of the gas if its final pressure is P0:
View SolutionTwo soap bubble are combined isothermally to form a big bubble of radius R. If ΔV is change in volume, ΔS is change in surface area and P0 is atmospheric pressure then show that 3P0(ΔV)+4T(ΔS)=0
View SolutionPressure P, Volume V and temperature T of a certain material are related by the P=αT2V. Here α is constant. Work done by the material when temparature changes from T0 to 2T0 while pressure remains constant is :
View SolutionA sample of ideal gas undergoes isothermal expansion in a reversible manner from volume V1 to volume V2. The initial pressure is P1 and the final pressure is P2. The same sample is then allowed to undergoes reversible expansion under adiabatic conditions from volume V1→V2. The initial pressure being same but final pressure is P3.
Which relation is correct (γ=CPCV)?
View SolutionA sample of ideal gas undergoes isothermal expansion in a reversible manner from volume V1 to volume V2. The initial pressure is P1 and the final pressure is P2. The same sample is then allowed to undergoes reversible expansion under adiabatic conditions from volume V1→V2. The initial pressure being same but final pressure is P3.
The work of expansion in adiabatic process (wadi) is related to work of expansion in isothermal process (wiso) is
View SolutionThe currect voltage relation of diode is given by I=(e1000V/T−1)mA, where the applied voltage V is in volt and the temperature T is in degree Kelvin. If a student makes an error measuring ±0.01V while measuring the current of 5mA at 300K, what will be error in the value of current in mA?
View SolutionGraphically show the total work done in an expansion when the state of an ideal gas is changed reversibly and isothermally from (pi,Vi) to (pf,Vf). With the help of a pV plot compare the work done in the above case with that carried out against a constant pressure pf.
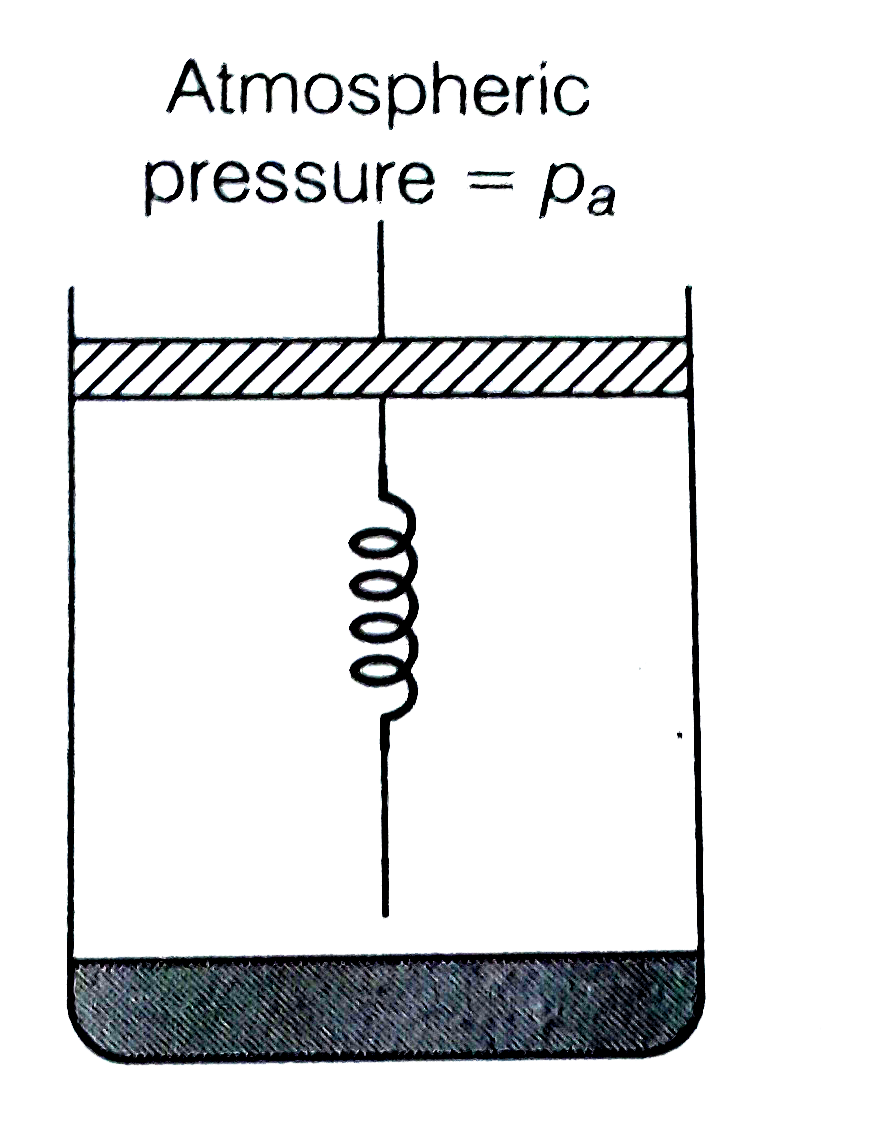
View SolutionConsider one mole of perfect gas on a cylinder of units cross-section with a piston attached (figure). A spring (spring constant K ) is attached ( unstretched length L ) to the piston and to the bottom of the cylinder . Initially the spring is unstretched and the gas is in equilibrium. A certain amount of heat Q is supplied to the gas causing an increase of value from V0 to V1.
(a) What is the initial pressure of the system ?
(b) What is the final pressure of the system?
(c) Using the first law of thermodynamic, write down a relation between Q,pa,V,V0 and K.
View SolutionTwo spherical soap bubble coalesce. If V is the consequent change in volume of the contained air and S the change in total surface area, show that
3PV+4ST=0
where T is the surface tension of soap bubble and P is
Atmospheric pressure
View SolutionVariation of volume with temperature was first studied by French chemist, Jacques Charles, in 1787 and then extended by another French chemist Joseph Gay-Lussac in 1802. For a fixed mass of a gas under isobaric condition, variation of volume V with temperature t°C is given by V = V0[l+αt] where V0 is the volume at 0°C, at constant pressure.
1 or every 1° change in temperature, the volume of the gas changes by............... of the volume at 0∘ C
View Solution
