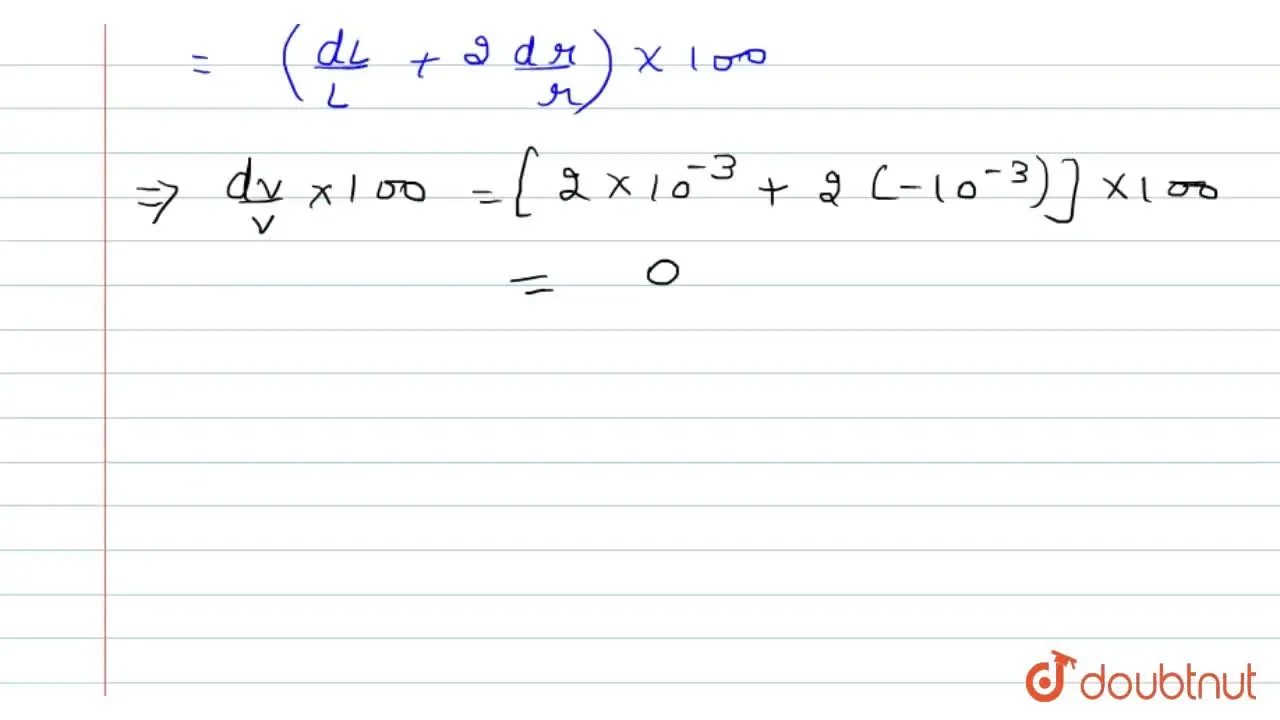A material has Poisson's ratio 0.50. If a uniform rod of it suffers a longitudinal strain of 2×10−3, then the percentage change in volume is
0.6
0.4
0.2
0.4
The correct Answer is:D
As the Poisson's ratio of a material is 0.5, so there is no change in volume.
Related Playlists
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - APPLICATIONS OF ELASTIC BEHAVIOUR OF MATERIALS8videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - HOTS8videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - STRESS-STRAIN CURVE5videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - NCERT Exemplar5videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOTION IN A PLANE EXERCISE - Assertion And Reason15videos
MOTION IN A PLANE
Similar Questions
A material has a Poisson's ratio 0.3. It suffers a uniform longitudinal strain 4.5×10−3, calculate the percentage change in its volume.
View SolutionA wire has Poisson's ratio 0.3. If the change in the length of the wire on stretching it is 1% then percentage change in the volume of the wire will be
View SolutionThe Poisson’s ratio for a metal is 0.25. If lateral strain is 0.0125, the longitudinal strain will be
View SolutionIf a force is applicable to an elastic wire of the material of Poisson's ratio 0.2 there is a decrease of the cross-sectional area by 1 % . The percentage increase in its length is :
View SolutionThe stress along the length of a rod with rectangular cross section) is 1% of the Young's modulus of its material. What is the approximate percentage of change of its volume? (Poisson's ratio of the material of the rod is 0.3)
View SolutionThe Poisson's ratio of a material is 0.4. If a force is applied to a wire of this material, there is a decrease of cross-sectional area by 2%. The percentage increase in its length is
View SolutionThe Poisson's ratio of a material is 0.5. If a force is applied to a wire of this material, there is a decrease in the cross-sectional area by 4%. The percentage increase in the length is
View SolutionA uniform wire (Y=2×1011N/m2) is subjected to a longitudinal tensile stress of 5×107N/m2. If the overall volume change in the wire is 0.02% the fractional decrease in its radius is 5k×10−6 Find the value of k
View SolutionThe Young's modulus of the material of a wire is 2×1010Nm−2 If the elongation strain is 1% then the energy stored in the wire per unit volume is Jm−3 is
View SolutionThe Young's modulus of the material of a wire is 2×1010Nm−2 If the elongation strain is 1% then the energy stored in the wire per unit volume in Jm−3 is
View Solution