If the boundary of system moves by an infinitesimal amount, the work involved is given by dw=−PextdV
for irreversible process w=−PextΔV (where ΔV=Vf−Vi)
for reversible process Pext=Pint±dP≅Pint
so for reversible isothermal process w=−nRTln.VfVi
2mole of an ideal gas undergoes isothermal compression along three different paths :
(i) reversible compression from Pi=2 bar and Vi=8L to Pf=20 bar
(ii) a single stage compression against a constant external pressure of 20 bar, and
(iii) a two stage compression consisting initially of compression against a constant external pressure of 10 bar until Pgas=Pext, followed by compression against a constant pressure of 20 bar until Pgas=Pext
Order of magnitude of work is :
w1>w2>w3
w3>w2>w1
w2>w3>w1
w1=w2=w3
The correct Answer is:C
To solve the problem of work done during isothermal compression of an ideal gas along three different paths, we will calculate the work done for each path step by step.
Step 1: Calculate Work Done for Reversible Compression
For a reversible isothermal process, the work done W can be calculated using the formula:
W=−nRTln(VfVi)
Given:
- n=2 moles
- Pi=2 bar
- Vi=8 L
- Pf=20 bar
First, we need to find the initial and final volumes using the ideal gas law:
PV=nRT
For initial conditions:
Vi=nRTiPi
Assuming isothermal conditions, we can use the same temperature for final conditions. We need to find Vf at Pf=20 bar.
Using the ideal gas law for final conditions:
Vf=nRTfPf
Since Ti=Tf, we can express Vf in terms of Vi:
VfVi=PiPf
Calculating Vf:
Vf8=220⟹Vf=8×220=0.8L
Now, substituting into the work formula:
W=−2RTln(0.88)
Step 2: Calculate Work Done for Single Stage Compression
For a single stage compression against a constant external pressure of Pext=20 bar, the work done is given by:
W=−PextΔV
Where ΔV=Vf−Vi=0.8−8=−7.2L.
Converting L to m3 (1 L = 0.001 m³):
ΔV=−7.2×0.001=−0.0072m3
Now substituting into the work formula:
W=−20×105×(−0.0072)=14400J
Step 3: Calculate Work Done for Two Stage Compression
In the two-stage compression, we first compress against a constant pressure of 10 bar until the gas pressure equals the external pressure, and then compress against 20 bar.
1. First Stage: Compress against 10 bar until Pgas=10 bar.
- Using the ideal gas law, we can find the volume at P=10 bar.
- V=nRT10
- Calculate V using the same T as before.
2. Second Stage: Compress against 20 bar from the volume found in the first stage to Vf.
The total work done will be the sum of the work done in both stages.
Summary of Work Done
1. Reversible Compression: W1 (calculated from Step 1)
2. Single Stage Compression: W2=14400J
3. Two Stage Compression: W3 (calculated from Step 3)
Conclusion
The order of magnitude of work done is:
W2>W3>W1
- n=2 moles
- Pi=2 bar
- Vi=8 L
- Pf=20 bar
- Using the ideal gas law, we can find the volume at P=10 bar.
- V=nRT10
- Calculate V using the same T as before.
2. Single Stage Compression: W2=14400J
3. Two Stage Compression: W3 (calculated from Step 3)
Topper's Solved these Questions
Similar Questions
If the boundary of system moves by an infinitesimal amount, the work involved is given by dw=−PextdV
for irreversible process w=−PextΔV (where ΔV=Vf−Vi)
for reversible process Pext=Pint±dP≅Pint
so for reversible isothermal process w=−nRTln.VfVi
2mole of an ideal gas undergoes isothermal compression along three different plaths :
(i) reversible compression from Pi=2 bar and Vi=8L to Pf=20 bar
(ii) a single stage compression against a constant external pressure of 20 bar, and
(iii) a two stage compression consisting initially of compression against a constant external pressure of 10 bar until Pgas=Pext, followed by compression against a constant pressure of 20 bar until Pgas=Pext
Work done on the gas in single stage compression is :
View SolutionIf the boundary of system moves by an infinitesimal amount, the work involved is given by dw=−PextdV
for irreversible process w=−PextΔV (where ΔV=Vf−Vi)
for reversible process Pext=Pint±dP≅Pint
so for reversible isothermal process w=−nRTln.VfVi
2mole of an ideal gas undergoes isothermal compression along three different plaths :
(i) reversible compression from Pi=2 bar and Vi=8L to Pf=20 bar
(ii) a single stage compression against a constant external pressure of 20 bar, and
(iii) a two stage compression consisting initially of compression against a constant external pressure of 10 bar until Pgas=Pext, followed by compression against a constant pressure of 20 bar until Pgas=Pext
Work done (in bar-L) on the gas in reversible isothermal compression is :
View SolutionIf the boundary of system moves by an infinitesimal amount, the work involved is given by dw=−PextdV
for irreversible process w=−PextΔV (where ΔV=Vf−Vi)
for reversible process Pext=Pint±dP≅Pint
so for reversible isothermal process w=−nRTln.VfVi
2mole of an ideal gas undergoes isothermal compression along three different plaths :
(i) reversible compression from Pi=2 bar and Vi=8L to Pf=20 bar
(ii) a single stage compression against a constant external pressure of 20 bar, and
(iii) a two stage compression consisting initially of compression against a constant external pressure of 10 bar until Pgas=Pext, followed by compression against a constant pressure of 20 bar until Pgas=Pext
Total work done on the gas in two stage compression is :
View SolutionOne mole of a monoatomic ideal gas undergoes the process A→B in the given P-V diagram. What is the specific heat for this process?
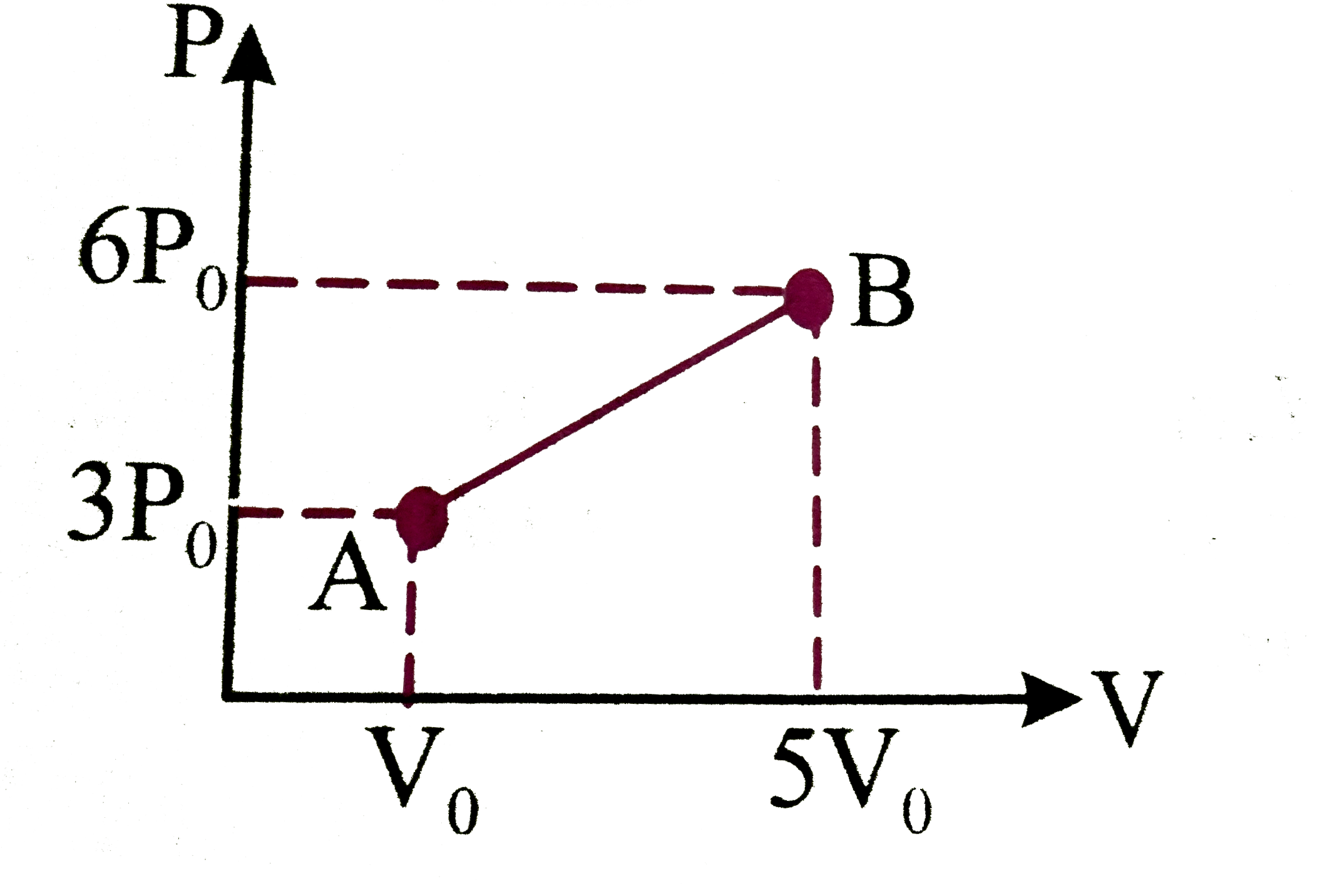
View SolutionA sample of gas is compressed from an initial volume of 2v0 to v0 using three different processes.
First: Using reversible isothermal
Second: Using reversible adiabatic
Third: Using irreversible adiabatic under a constant external pressure then
View SolutionFor a reversible process at T = 300K, the volume is increased from Vi=1L to Vf=10L. Calculate ΔH if the process is isothermal -
View SolutionA gas is expanded from volume V1 to V2 through three different process:
a. Reversible adiabatic
b. Reversible isothermal
c. Irreversible adiabatic (against a constant external pressure Pex)
The correct option is
View SolutionTwo moles of an ideal gas undergo the following process :
(a) a reversible isobaric expansion from (P atm, VL) to (P atm , 2VL).
(b) a reversible isochoric change of state from (P atm , 2V,L) to (P/2 atm, 2VL)
(c) a reversible isothermal compression from (P/2 atm, 2VL) to (P atm, VL).
Sketch with labels each of the processes on the same P−V diagram.
View SolutionA sample of ideal gas undergoes isothermal expansion in a reversible manner from volume V1 to volume V2. The initial pressure is P1 and the final pressure is P2. The same sample is then allowed to undergoes reversible expansion under adiabatic conditions from volume V1→V2. The initial pressure being same but final pressure is P2.
If P3 and P2 are equal, then
View SolutionA sample of ideal gas undergoes isothermal expansion in a reversible manner from volume V1 to volume V2. The initial pressure is P1 and the final pressure is P2. The same sample is then allowed to undergoes reversible expansion under adiabatic conditions from volume V1→V2. The initial pressure being same but final pressure is P2.
Which graphic representation is correct
a.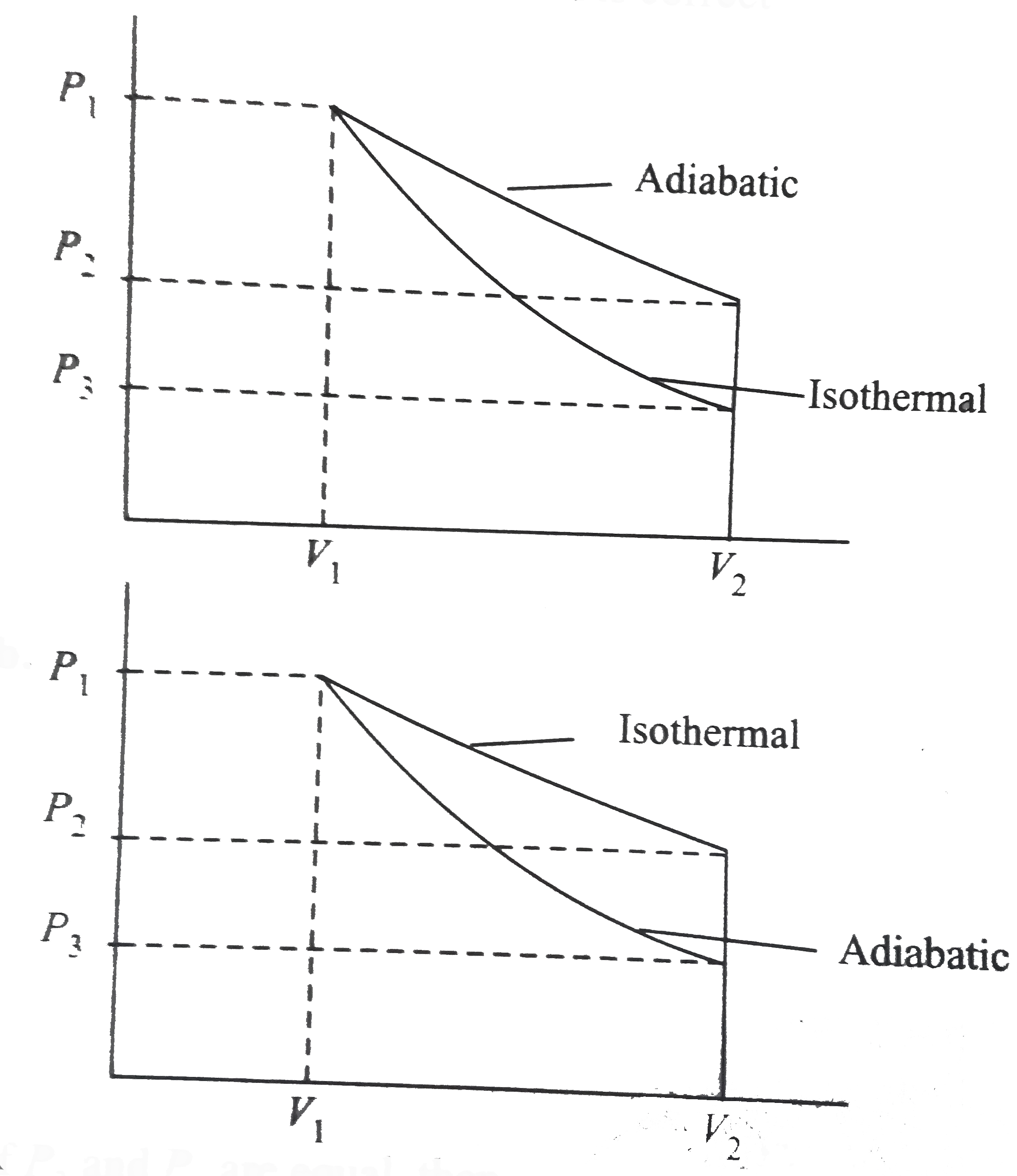
View Solution
