Question

Two moles of an ideal gas undergo the following process $:$
$\left(a\right)$ a reversible isobaric expansion from $(P$ atm, $VL)$ to $(P$ atm , $2VL)$.
$\left(b\right)$ a reversible isochoric change of state from $(P$ atm , $2V,L)$ to $(P/2$ atm, $2VL)$
$\left(c\right)$ a reversible isothermal compression from $(P/2$ atm, $2VL)$ to $(P$ atm, $VL)$.
Sketch with labels each of the processes on the same $P-V$ diagram.

Options

A.
B.
C.
D.

Answer

3

Explanation

To solve the problem, we need to analyze the three processes undergone by the ideal gas and sketch them on a P-V diagram. Let's break down each step:

Step 1: Identify the Processes
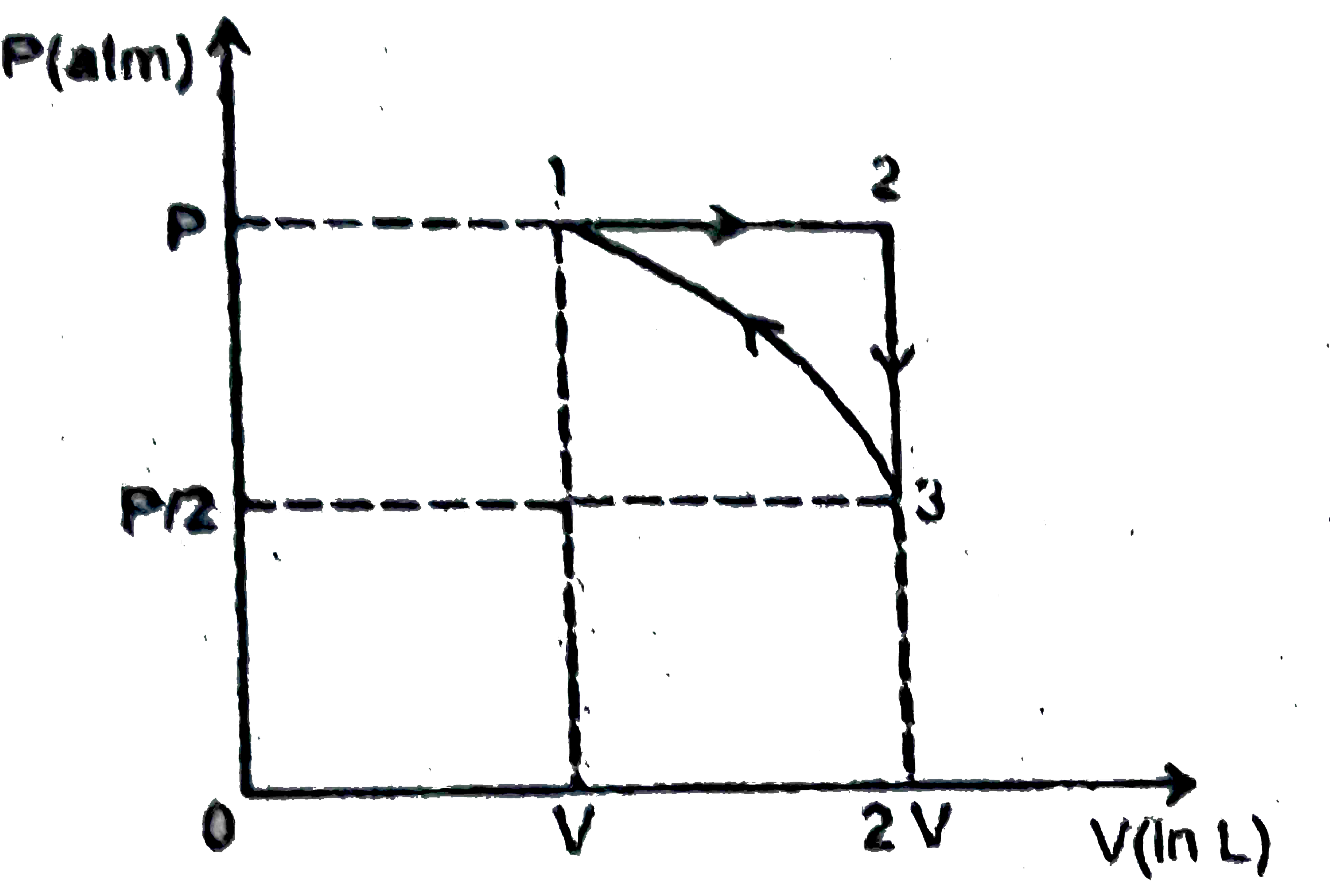
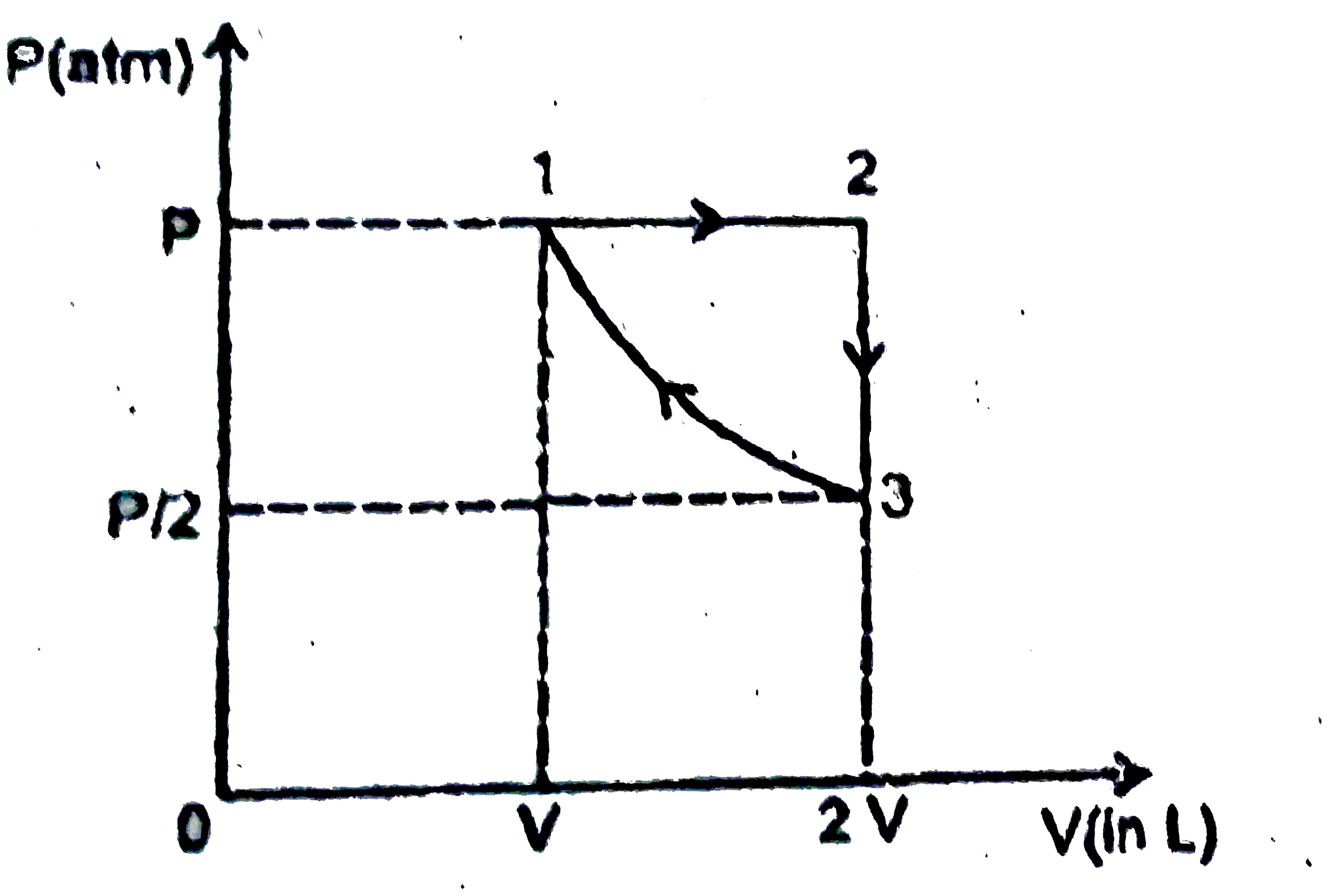
1. Isobaric Expansion: The gas expands at constant pressure from (P atm, V L) to (P atm, 2V L).
2. Isochoric Change: The gas undergoes a change at constant volume from (P atm, 2V L) to (P/2 atm, 2V L).
3. Isothermal Compression: The gas is compressed at constant temperature from (P/2 atm, 2V L) to (P atm, V L).

Step 2: Sketch the P-V Diagram
1. Draw the Axes:
- Draw a vertical line for pressure (P) and a horizontal line for volume (V).

2. Process (a) - Isobaric Expansion:
- Start at point A (P atm, V L).
- Move horizontally to the right to point B (P atm, 2V L) since pressure is constant (isobaric).

3. Process (b) - Isochoric Change:
- From point B (P atm, 2V L), move vertically downwards to point C (P/2 atm, 2V L) since volume is constant (isochoric).

4. Process (c) - Isothermal Compression:
- From point C (P/2 atm, 2V L), draw a curve back to point A (P atm, V L). This curve should be hyperbolic since it represents an isothermal process where pressure and volume are inversely related.

Step 3: Label the Points
- Label point A as (P atm, V L).
- Label point B as (P atm, 2V L).
- Label point C as (P/2 atm, 2V L).

Final Sketch
The final P-V diagram will show:
- A horizontal line from A to B for the isobaric expansion.
- A vertical line from B to C for the isochoric change.
- A hyperbolic curve from C back to A for the isothermal compression.