If the boundary of system moves by an infinitesimal amount, the work involved is given by dw=−PextdV
for irreversible process w=−PextΔV (where ΔV=Vf−Vi)
for reversible process Pext=Pint±dP≅Pint
so for reversible isothermal process w=−nRTln.VfVi
2mole of an ideal gas undergoes isothermal compression along three different plaths :
(i) reversible compression from Pi=2 bar and Vi=8L to Pf=20 bar
(ii) a single stage compression against a constant external pressure of 20 bar, and
(iii) a two stage compression consisting initially of compression against a constant external pressure of 10 bar until Pgas=Pext, followed by compression against a constant pressure of 20 bar until Pgas=Pext
Work done (in bar-L) on the gas in reversible isothermal compression is :
9.212
36.848
18.424
None of these
The correct Answer is:B
To solve the problem of calculating the work done on the gas during reversible isothermal compression, we will follow these steps:
Step 1: Identify the Given Values
- Initial Pressure, Pi=2 bar
- Initial Volume, Vi=8 L
- Final Pressure, Pf=20 bar
- Number of moles, n=2
Step 2: Use the Ideal Gas Law to Find Final Volume
For an ideal gas, the relationship between pressure and volume at constant temperature is given by:
PiVi=PfVf
We can rearrange this to find Vf:
Vf=PiViPf
Substituting the known values:
Vf=2bar×8L20bar=1620=0.8L
Step 3: Calculate the Work Done Using the Formula for Reversible Isothermal Process
The work done on the gas during a reversible isothermal process is given by:
w=−nRTln(VfVi)
However, we need to express RT in terms of the initial conditions. We can use the ideal gas law to find T:
PV=nRT⟹T=PiVinR
Substituting the values:
T=2bar×8L2×R=162R=8R
Step 4: Substitute T Back into the Work Formula
Now we can substitute T into the work equation:
w=−n(8R)Rln(0.88)
This simplifies to:
w=−8ln(0.88)
Calculating 0.88=0.1:
w=−8ln(0.1)
Step 5: Calculate ln(0.1)
Using the properties of logarithms:
ln(0.1)=ln(10−1)=−ln(10)
Thus:
w=−8×(−ln(10))=8ln(10)
Step 6: Substitute the Value of ln(10)
Using ln(10)≈2.303:
w=8×2.303=18.424bar-L
Final Answer
The work done on the gas in reversible isothermal compression is approximately:
18.424bar-L
- Initial Pressure, Pi=2 bar
- Initial Volume, Vi=8 L
- Final Pressure, Pf=20 bar
- Number of moles, n=2
For an ideal gas, the relationship between pressure and volume at constant temperature is given by:
PiVi=PfVf
We can rearrange this to find Vf:
Vf=PiViPf
Substituting the known values:
Vf=2bar×8L20bar=1620=0.8L
The work done on the gas during a reversible isothermal process is given by:
w=−nRTln(VfVi)
However, we need to express RT in terms of the initial conditions. We can use the ideal gas law to find T:
PV=nRT⟹T=PiVinR
Substituting the values:
T=2bar×8L2×R=162R=8R
Now we can substitute T into the work equation:
w=−n(8R)Rln(0.88)
This simplifies to:
w=−8ln(0.88)
Calculating 0.88=0.1:
w=−8ln(0.1)
Using the properties of logarithms:
ln(0.1)=ln(10−1)=−ln(10)
Thus:
w=−8×(−ln(10))=8ln(10)
Using ln(10)≈2.303:
w=8×2.303=18.424bar-L
The work done on the gas in reversible isothermal compression is approximately:
18.424bar-L
Topper's Solved these Questions
Similar Questions
If the boundary of system moves by an infinitesimal amount, the work involved is given by dw=−PextdV
for irreversible process w=−PextΔV (where ΔV=Vf−Vi)
for reversible process Pext=Pint±dP≅Pint
so for reversible isothermal process w=−nRTln.VfVi
2mole of an ideal gas undergoes isothermal compression along three different paths :
(i) reversible compression from Pi=2 bar and Vi=8L to Pf=20 bar
(ii) a single stage compression against a constant external pressure of 20 bar, and
(iii) a two stage compression consisting initially of compression against a constant external pressure of 10 bar until Pgas=Pext, followed by compression against a constant pressure of 20 bar until Pgas=Pext
Order of magnitude of work is :
View SolutionIf the boundary of system moves by an infinitesimal amount, the work involved is given by dw=−PextdV
for irreversible process w=−PextΔV (where ΔV=Vf−Vi)
for reversible process Pext=Pint±dP≅Pint
so for reversible isothermal process w=−nRTln.VfVi
2mole of an ideal gas undergoes isothermal compression along three different plaths :
(i) reversible compression from Pi=2 bar and Vi=8L to Pf=20 bar
(ii) a single stage compression against a constant external pressure of 20 bar, and
(iii) a two stage compression consisting initially of compression against a constant external pressure of 10 bar until Pgas=Pext, followed by compression against a constant pressure of 20 bar until Pgas=Pext
Work done on the gas in single stage compression is :
View SolutionIf the boundary of system moves by an infinitesimal amount, the work involved is given by dw=−PextdV
for irreversible process w=−PextΔV (where ΔV=Vf−Vi)
for reversible process Pext=Pint±dP≅Pint
so for reversible isothermal process w=−nRTln.VfVi
2mole of an ideal gas undergoes isothermal compression along three different plaths :
(i) reversible compression from Pi=2 bar and Vi=8L to Pf=20 bar
(ii) a single stage compression against a constant external pressure of 20 bar, and
(iii) a two stage compression consisting initially of compression against a constant external pressure of 10 bar until Pgas=Pext, followed by compression against a constant pressure of 20 bar until Pgas=Pext
Total work done on the gas in two stage compression is :
View SolutionDuring expansion of a gas into vaccum (Pext=0), Work done is zero if the process is
(A) Reversible (B) Irreversible (C ) Isothermal
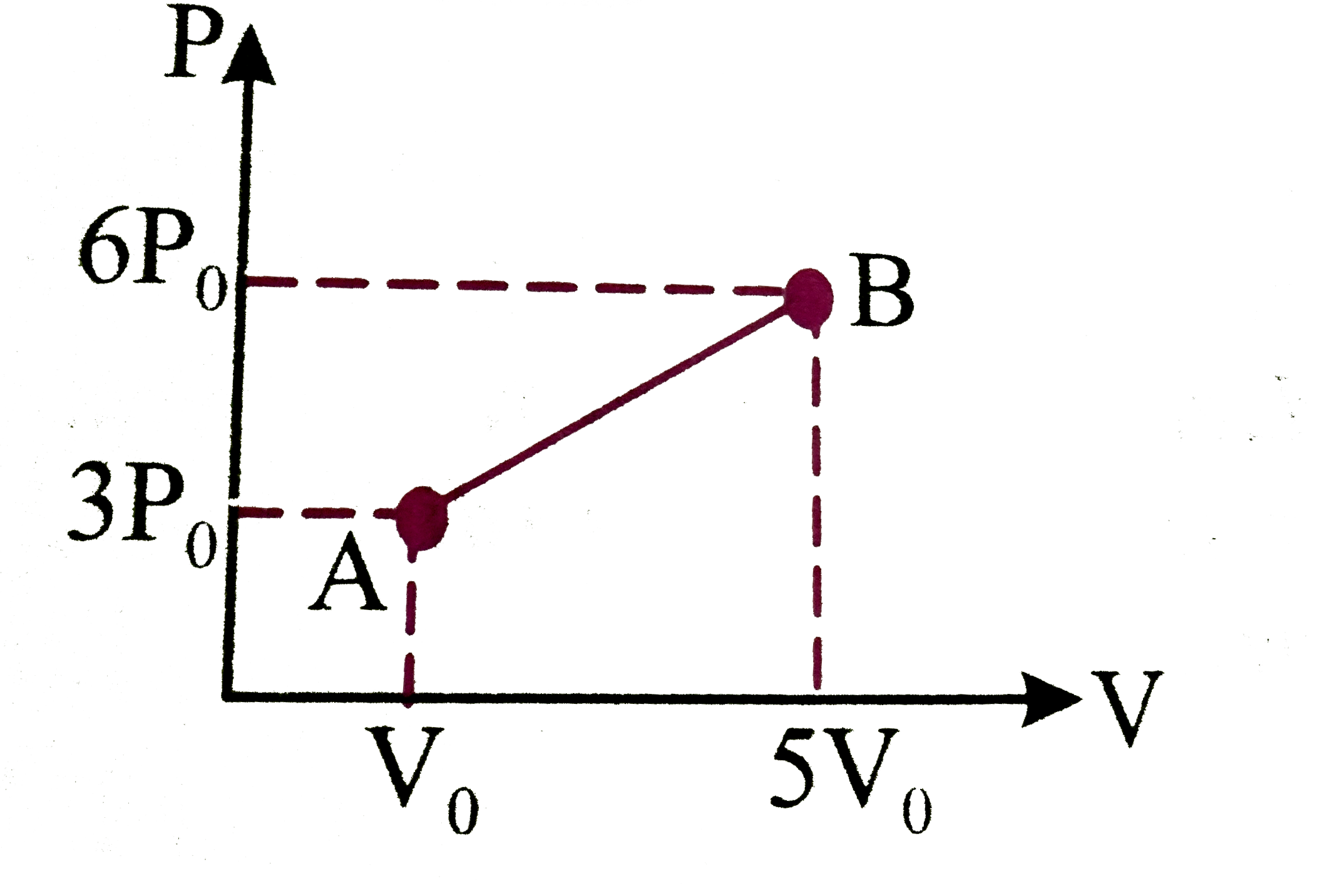
View SolutionOne mole of a monoatomic ideal gas undergoes the process A→B in the given P-V diagram. What is the specific heat for this process?
View SolutionA sample of gas is compressed from an initial volume of 2v0 to v0 using three different processes.
First: Using reversible isothermal
Second: Using reversible adiabatic
Third: Using irreversible adiabatic under a constant external pressure then
View SolutionFor a reversible process at T = 300K, the volume is increased from Vi=1L to Vf=10L. Calculate ΔH if the process is isothermal -
View SolutionWhich of the following P-V curve best represents. As isothermal process?
View SolutionA gas is expanded from volume V1 to V2 through three different process:
a. Reversible adiabatic
b. Reversible isothermal
c. Irreversible adiabatic (against a constant external pressure Pex)
The correct option is
View SolutionTwo moles of an ideal gas undergo the following process :
(a) a reversible isobaric expansion from (P atm, VL) to (P atm , 2VL).
(b) a reversible isochoric change of state from (P atm , 2V,L) to (P/2 atm, 2VL)
(c) a reversible isothermal compression from (P/2 atm, 2VL) to (P atm, VL).
Sketch with labels each of the processes on the same P−V diagram.
View Solution
