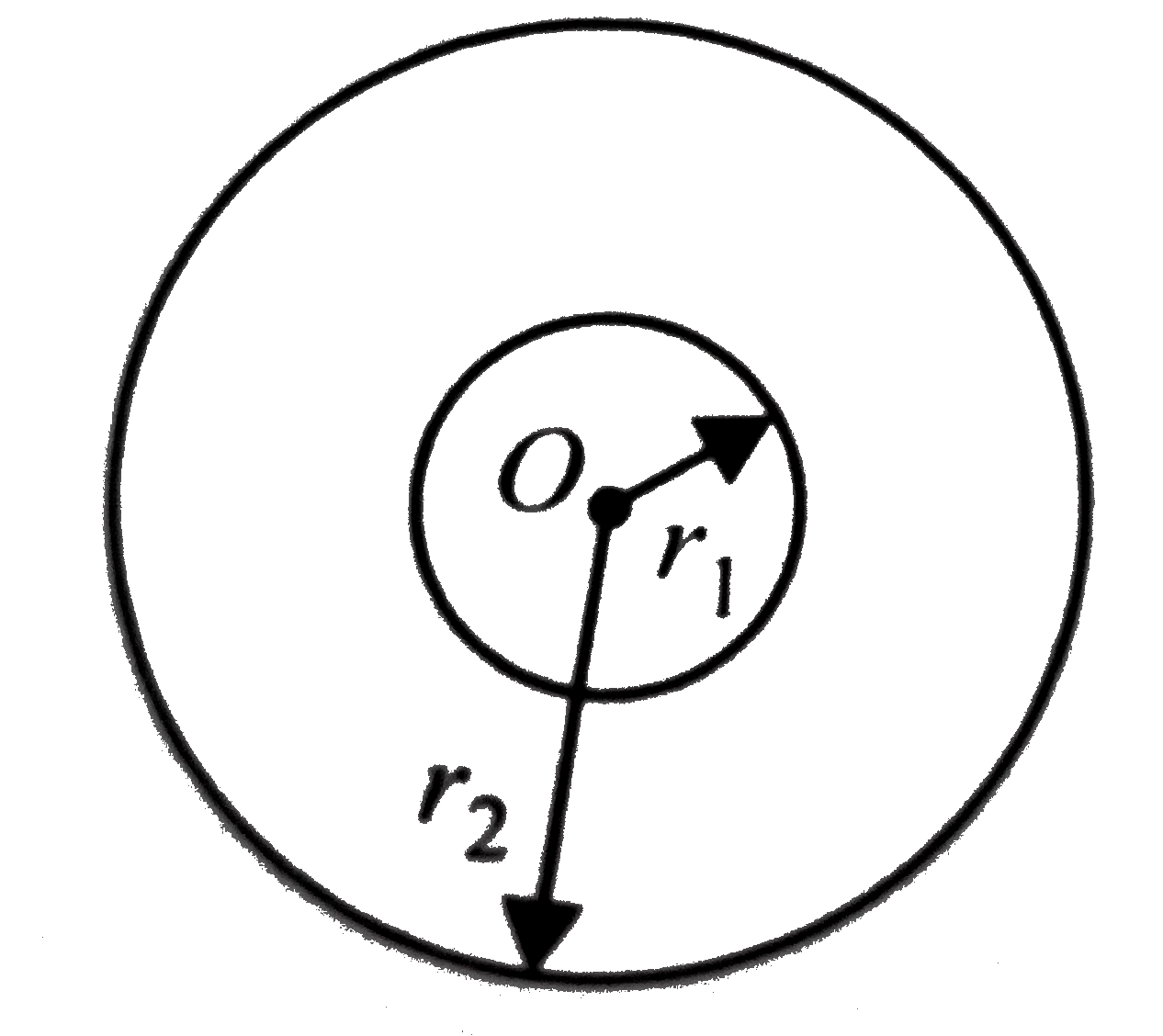
Two thin metallic spherical shells of radii $r_{1}$ and $r_{2}$ $(r_{1} < r_{2})$ are placed with their centres coinciding. A material of thermal conductivity K is filled in the space between the shells. The inner shells is maintained at temperature $θ_{1}$ .and the outer shell at temperature $θ_{2}$ $(θ_{1} < θ_{2})$ . Calculate the rate at which heat flows radially through the material.

Video Solution

Text Solution

Verified by Experts

Let us drow two spherical shells of radii x and $x + d x$ concentric with the given system. Let the temperatures at these shells be theta and $θ + d t h η$ respectively. The amount of heat flowing radially inward through the material between x and $x + d x$ is
$\frac{Δ Q}{Δ t} = \frac{K 4 π x^{2} d θ}{d x}$ . thus, $K 4 π \int_{θ_{1}}^{θ_{2}} d θ = \frac{Δ Q}{Δ t} \int_{r 1}^{R 2} \frac{d x}{x^{2}}$ . or, $K 4 π (θ_{2} - θ_{1}) = \frac{Δ Q}{Δ t} (\frac{1}{R_{1}} - \frac{1}{R_{2}})$ . or, (DeltaQ)/(Deltat)=(4piKr_(1)r_(2)(theta_(2)-theta_(1)))/(r_(2)-r_(1))

Updated on:21/07/2023