A blackbody of surface area 1cm2 is placed inside an enclosure. The enclosure has a constant temperature 27(∘)C and the blackbody is maintained at 327∘C by heating it electrically. What electric power is needed to maintain the temperature? σ=6.0×10−8Wm−2K−2 .
To find the electric power needed to maintain the temperature of a blackbody in an enclosure, we can follow these steps:
Step 1: Convert temperatures from Celsius to Kelvin
- The temperature of the blackbody (T1) is given as 327°C. To convert to Kelvin:
T1=327+273=600K
- The temperature of the enclosure (T2) is given as 27°C. To convert to Kelvin:
T2=27+273=300K
Step 2: Convert the surface area from cm² to m²
- The surface area (A) is given as 1 cm². To convert to m²:
A=1cm2=1×10−4m2
Step 3: Use the Stefan-Boltzmann Law to calculate emitted and absorbed power
- The power emitted by the blackbody (P_emitted) is given by:
Pemitted=σAT14
- The power absorbed by the enclosure (P_absorbed) is given by:
Pabsorbed=σAT24
Step 4: Calculate the net rate of energy loss
- The net rate of energy loss (P_net) is the difference between the emitted and absorbed power:
Pnet=Pemitted−Pabsorbed=σA(T14−T24)
Step 5: Substitute the known values into the equation
- Given σ=6.0×10−8W/m2K2, A=1×10−4m2, T1=600K, and T2=300K:
Pnet=6.0×10−8×1×10−4×(6004−3004)
Step 6: Calculate T14 and T24
- Calculate T14:
T14=6004=1.296×1011
- Calculate T24:
T24=3004=8.1×109
Step 7: Compute the difference T14−T24
- Calculate the difference:
T14−T24=1.296×1011−8.1×109≈1.288×1011
Step 8: Substitute back to find the power needed
- Now substitute back into the equation:
Pnet=6.0×10−8×1×10−4×1.288×1011
Pnet≈0.73W
Conclusion
The electric power needed to maintain the temperature of the blackbody is approximately 0.73 watts.
---
- The temperature of the blackbody (T1) is given as 327°C. To convert to Kelvin:
T1=327+273=600K
- The temperature of the enclosure (T2) is given as 27°C. To convert to Kelvin:
T2=27+273=300K
- The surface area (A) is given as 1 cm². To convert to m²:
A=1cm2=1×10−4m2
- The power emitted by the blackbody (P_emitted) is given by:
Pemitted=σAT14
- The power absorbed by the enclosure (P_absorbed) is given by:
Pabsorbed=σAT24
- The net rate of energy loss (P_net) is the difference between the emitted and absorbed power:
Pnet=Pemitted−Pabsorbed=σA(T14−T24)
- Given σ=6.0×10−8W/m2K2, A=1×10−4m2, T1=600K, and T2=300K:
Pnet=6.0×10−8×1×10−4×(6004−3004)
- Calculate T14:
T14=6004=1.296×1011
- Calculate T24:
T24=3004=8.1×109
- Calculate the difference:
T14−T24=1.296×1011−8.1×109≈1.288×1011
- Now substitute back into the equation:
Pnet=6.0×10−8×1×10−4×1.288×1011
Pnet≈0.73W
The electric power needed to maintain the temperature of the blackbody is approximately 0.73 watts.
Topper's Solved these Questions
- HEAT TRANSFERBOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - OBJECTIVE I
10 Videos
- HEAT TRANSFERBOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - OBJECTIVE II
6 Videos
- HEAT TRANSFERBOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - QUESTIONS FOR SHORT ANSWER
11 Videos
- HEAT AND TEMPERATUREBOOK - HC VERMA ENGLISHCHAPTER - HEAT AND TEMPERATUREEXERCISE - Objective 2
6 Videos
- INTRODUCTION TO PHYSICSBOOK - HC VERMA ENGLISHCHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Question for short Answer
4 Videos
Similar Questions
A blackbody of surface area 10cm2 is heated to 127∘C and is suspended in a room at temperature 27∘C calculate the initial rate of loss of heat from the body to the room.
View SolutionA spherical tungsten pieces of radius 1.0cm is suspended in an evacuated chamber maintained at 300K. The pieces is maintained at 1000K by heating it electrically. Find the rate at which the electrical energy must be supplied. The emissivity of tungsten is 0.30 and the Stefan constant σ is 6.0×10−sWm−2K−4 .
View SolutionA thin brass rectangular sheet of sides 10 cm and 5 cm is heated in a furnace to 500∘ C and taken out. How much electric power is needed to maintain the sheet at this temperature ? Its emissivity is 0.25.
View SolutionAn electric heater emits 1000W of thermal radiation. The coil has a surface area of 0.02m2 . Assuming that the coil radiates like a blackbody, Find its temperature. σ=6.0×10−8Wm−2K−2 .
View SolutionA black body of mass 34.38 g and surface area 19.2cm2 is at an intial temperature of 400 K. It is allowed to cool inside an evacuated enclosure kept at constant temperature 300 K. The rate of cooling is 0.04∘C/s. The sepcific heat of the body Jkg−1K−1 is
(Stefan's constant, σ=5.73×10−8Wm−2K−4)
View SolutionA copper sphere is suspended in an evacuated chamber maintained at 300K. The sphere is maintained at a constant temperature of 500K by heating it electrically. A total of 210W of electric power is needed to do it. When the surface of the copper sphere is completely blackened, 700W is needed to maintain the same temperature of the sphere. Calculate the emissivity of copper.
View SolutionA copper sphere is suspended in an evacuated chamber maintained at 300K. The sphere is maitained at a constant temperature of 500K by heating it electrically. A total of 210W is electric power is needed to do it. When the surface of the copper sphere is completely blackned, 700W is needed to maintain the same temperature of the sphere. Calculate the emissivity of copper.
View SolutionA metal ball of surface area 200cm2 and temperature 527∘C is surrounded by a vessel at 27∘C . If the emissivity of the metal is 0.4, then the rate of loss of heat from the ball is (σ=5.67×10−8J/m2−s−k4)
View SolutionFigure shows a steel rod joined to a brass rod. Each of the rods has length of 31cm and area of cross-section 0.20cm2. The junction is maintained at a constant temperature 50∘C and the two ends are maintained at 100∘C. The amount of heat taken out from the cold junction in 10 minutes after the steady state is reached in n×102J. Find 'n' the thermal conductivities are Ksteel=46W/m−∘C and Kbrass=109W/m−∘C.
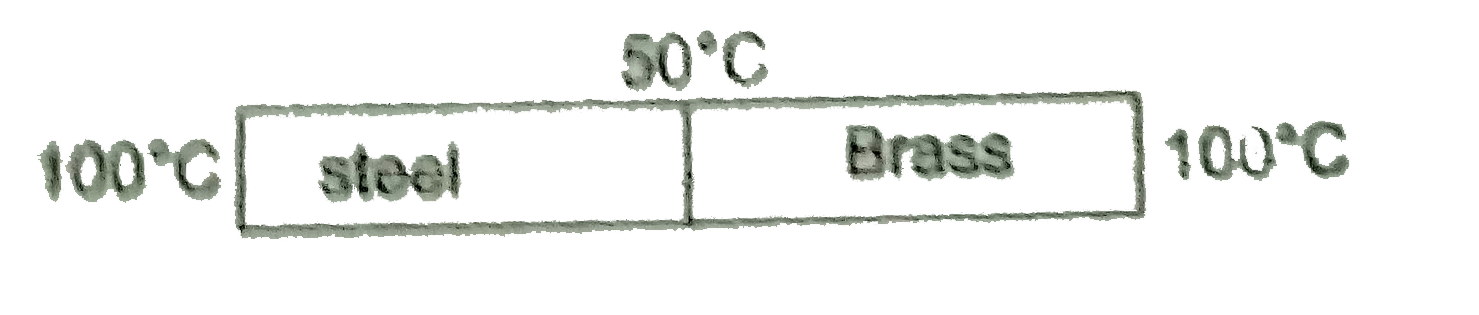
View SolutionCalculate the amount of heat radiated per second by a body of surface area 12cm2 kept in thermal equilibrium in a room at temperature 20∘C The emissivity of the surface =0.80 and σ=6.0×10−sWm−2K−4 .
View Solution
