A wave pulse is travelling on a string with a speed v towards the positive x-axis. The shape of the string at t=0 is given by y(x)=Asin(x/a), where Aanda are constants.
(a) What are the dimensions of Aanda?
(b) Write the equation of the wave for a general time t, if the wave speed is v.
The correct Answer is:A
At t=t0,{t(x,t0)}=Asin(xa)
For a wave travelling in the positive x-direction the general equationn is given by
y=Asin(xa−tT)
Putting t=-t and comparing with equation 1 we get
→g(x,0)=Asin{(xa)+(t0t)}
→g(x,t)=Asin[{(xa)+t0T}−(tT)]
As T=a/v<br>a=wave≤n>hv=speedofthewave<br>rarr y=A sin[(x/y)+t_0/((a/v))-t/((a/v))<br>rarr y=Asin(x+v(t_0-t))/a
Topper's Solved these Questions
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer
7 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Objective -2
9 Videos
- THE FORCESBOOK - HC VERMA ENGLISHCHAPTER - THE FORCESEXERCISE - Questions for short Answer
9 Videos
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Question for Short Answer
16 Videos
Similar Questions
A wave pulse is travelling on a string with a speed v towards the positive X-axis. The shape of the string at t = 0 is given by g(x)=Asin(xa), where A and a are constants. (a) What are the dimensions of A and a ? (b) Write the equation of the wave for a general time 1, if the wave speed is v.
View SolutionThe equation of a wave travelling along the positive x-axis, as shown in figure at t = 0 is given by
View SolutionA wave propagates in a string in the positive x-direction with velocity v. The shape of the string at t=t0 is given by
f(x,t0)=Asin(x2a2). Then the wave equation at any instant t is given by
View Solutionthe equation of a wave travelling along the positive x - axis ,as shown in figure at t = 0 is given by
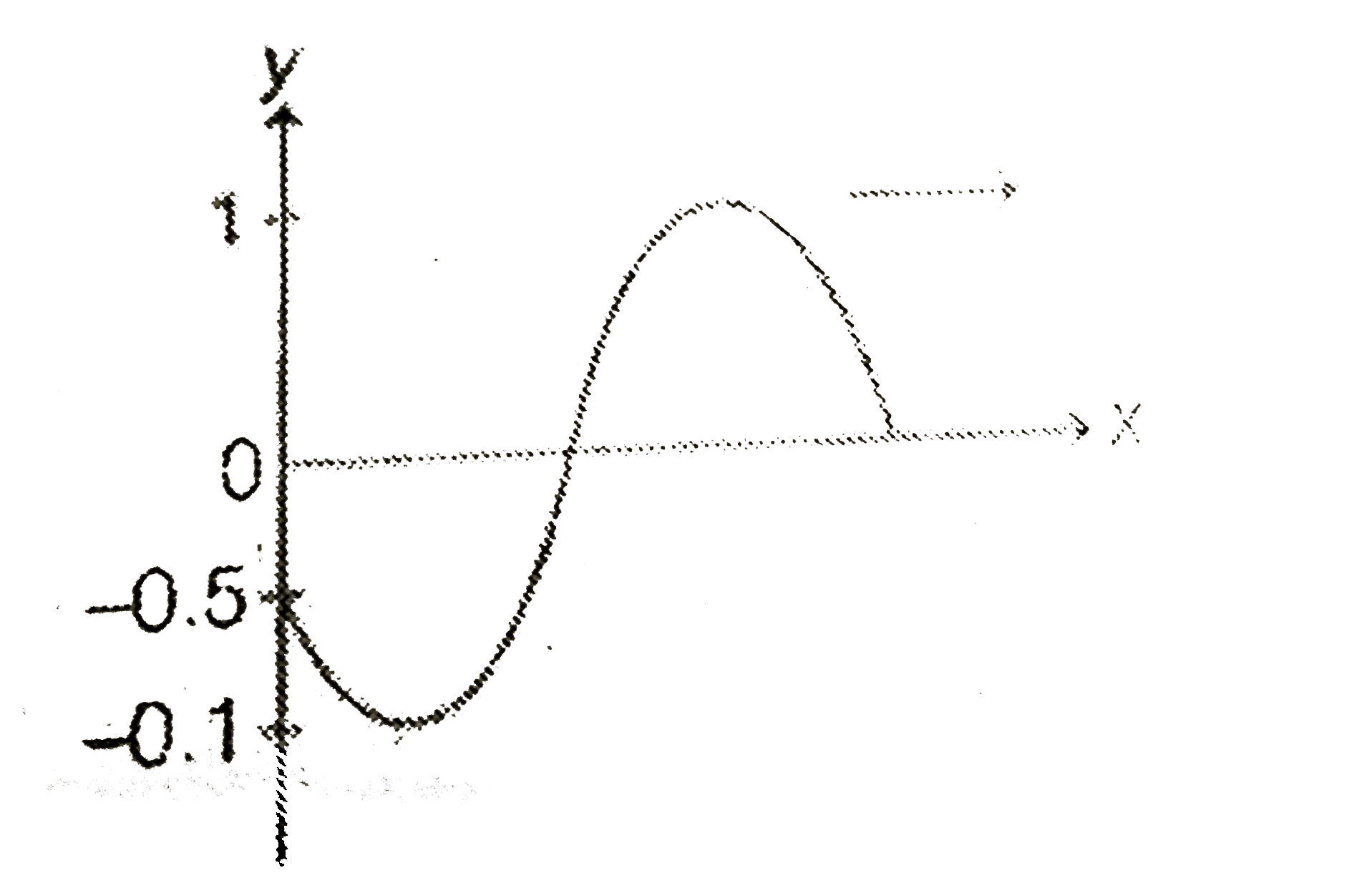
View SolutionThe equation of a wave travelling on a string stretched along the X-axis is given by
y=Ae(−xa+tT)2
(a) Write the dimensions of A, a and T.
(b) Find the wave speed.
(c) In which direction is the wave travelling?
(d) Where is the maximum of the pulse located at t=T and at t=2T?
View SolutionA pulse is propagating on a long stretched string along its length taken as positive x-axis. Shape of the string at t = 0 is given by y=√a2−x2when |x|≤a=0 when |x|≥a . What is the general equation of pulse after some time 't' , if it is travelling along positive x-direction with speed V?
View SolutionThe equation of a wave is given by
y=asin(100t−x10) where x and y are in metre an t in second, the velocity of wave is
View SolutionWhen a wave transverses in a medium, the displacement of a particle located at distance x at time t is given by y=asin(bt−cx) where a, b and c are constants of the wave. The dimension of b/c are same as that of:
View SolutionA wave pulse is travelling positive x direction with a speed of 4.5 m/s. At time t= 0, the wave pulse is given as y=6x2−3. Here x and y are in metre. Then which function represents the equation of pulse just after 2 second?
View SolutionA wave pulse on a string on a string has the dimension shown in figure. The wave speed is v=1 cm /s . If point O is a free end. The shape of wave at time t = 3s si

View Solution
