A scooter weighing 150 g together with its rider moving at 36 km/hr is to take a turn of radius 30 m. What horizontal force on the scooter is needed to make the turn possible?
To solve the problem of determining the horizontal force needed for a scooter weighing 150 kg (including the rider) to make a turn of radius 30 m while moving at a speed of 36 km/h, we can follow these steps:
Step 1: Convert the speed from km/h to m/s
The speed of the scooter is given as 36 km/h. To convert this to meters per second (m/s), we use the conversion factor:
1 km/h=518 m/s
Thus,
Speed=36×518=10 m/s
Step 2: Identify the formula for centripetal force
The horizontal force required to maintain circular motion (centripetal force) can be calculated using the formula:
F=mv2r
where:
- F is the centripetal force,
- m is the mass of the scooter and rider,
- v is the velocity,
- r is the radius of the turn.
Step 3: Substitute the known values into the formula
Given:
- Mass m=150 kg
- Velocity v=10 m/s
- Radius r=30 m
Substituting these values into the formula:
F=150×(10)230
Step 4: Calculate the centripetal force
Calculating the values:
F=150×10030
F=1500030=500 N
Conclusion
The horizontal force required for the scooter to make the turn is 500 N.
---
The speed of the scooter is given as 36 km/h. To convert this to meters per second (m/s), we use the conversion factor:
1 km/h=518 m/s
Thus,
Speed=36×518=10 m/s
The horizontal force required to maintain circular motion (centripetal force) can be calculated using the formula:
F=mv2r
where:
- F is the centripetal force,
- m is the mass of the scooter and rider,
- v is the velocity,
- r is the radius of the turn.
Given:
- Mass m=150 kg
- Velocity v=10 m/s
- Radius r=30 m
F=150×(10)230
Calculating the values:
F=150×10030
F=1500030=500 N
The horizontal force required for the scooter to make the turn is 500 N.
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Question for short Answer
10 Videos
- CIRCULAR MOTIONBOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Objective -2
6 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective -2
11 Videos
- FLUID MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - All Questions
90 Videos
Similar Questions
A scooter weighing 150 kg together with its rider moving at 36 km/hr is to take a turn of radius 30 m. What horizontal force on the scooter is needed to make the turn possible?If the horizontal force needed for the turn in the previous problem is to be supplied by the normal force by the road what should be the proper angle of banking?
View SolutionA track consists of two circular pars ABC and CDE of equal radius 100 m and joined smoothly as shown in figure.Each part subtends a right angle at its centre. A cycle weighing 100 kg together with rider travels at a constant speed of 18 km/h on the track. A. Find the normal contact force by the road on the cycle when it is at B and at D. b.Find the force of friction exerted by the track on the tyres when the cycle is at B,C and D. c. Find the normal force between the road and the cycle just before and just after the cycle crosses C. d. What should be the minimum friction coefficient between the road and the tyre, which will ensure that the cyclist can move with constant speed? Take g=10ms2

View Solution
Knowledge Check
A 4m long ladder weighing 25kg rests with its upper end against a smooth wall and lower end on rough ground.What should be the minimum coefficient of friction between the ground and the ladder for it to be inclined at 60∘ with the horizontal without slipping? (Takeg=10m/s2)
Aa. 0.19Bb. 0.29Cc. 0.39Dd. 0.49
The centripetal force required by a 1000 kg car that takes a turn of radius 50 m at a speed of 36 kmph is
View SolutionA 500 kg car takes a round turn of radius 50 m with a velocity of 36 km/hr . The centripetal force is
View SolutionA car moving at a speed of 36 km/hr is taking a turn on a circular road of radius 50 m. A small wooden plate is kept on the seat with its plane perpendicular to the radius of the circular road figure. A small bock of mass 100 g is kept on the seat which rests against the plate. The friction coefficient between the block and the plate is μ=0.58. a. Find the normal contact force exerted by the plate on the block. b. The plate is slowly turned so that the angle between the normal to the plate and the radius of the road slowly increases Find the angle at which the block will just start sliding on the plate.

View SolutionA track consists of two circular pars ABC andCDE of equal rdius 100 m and joined smoothly as shown in figure.Each part sutends a right ngle at its centre. A cycle weighing 100 kg together with rider travels at a constant speed of 18 km/h on the track. A. Findteh nromal contct force by tehroad on the cycle whenit is at B and at D. b.Findteh force of friction exerted by the track on the tyres when the cycle is at B,C and D. c. Find the normal force between teh road and teh cycle just before and just after the cycle crosses C. d. What should be the minimum friction coefficient between the road and the tyre, which will ensure that teh cyclist can move with constant speed? Take g=10ms2

View SolutionAn aeroplane travelling at a speed of 500 km/hr tilts at an angle of 30° as it makes a turn. What is the radius of the curve ?
View SolutionA4m long ladder weighing 25kg rests with its upper and against a smooth wall and lower and on rought ground.What should be the minimum coefficient of friction between the ground and ladder for it to be inclined at 60∘ with the horizontal without slipping? (Takeg=10m/s2)
View SolutionA bead of mass 12kg starts from rest from A to move in a vertical place along a smooth fixed quarter ring of radius 5m, under the action of a constant horizontal force f=5N as shown. The speed of bead as it reaches the point (B) is [Take g=10ms−2]
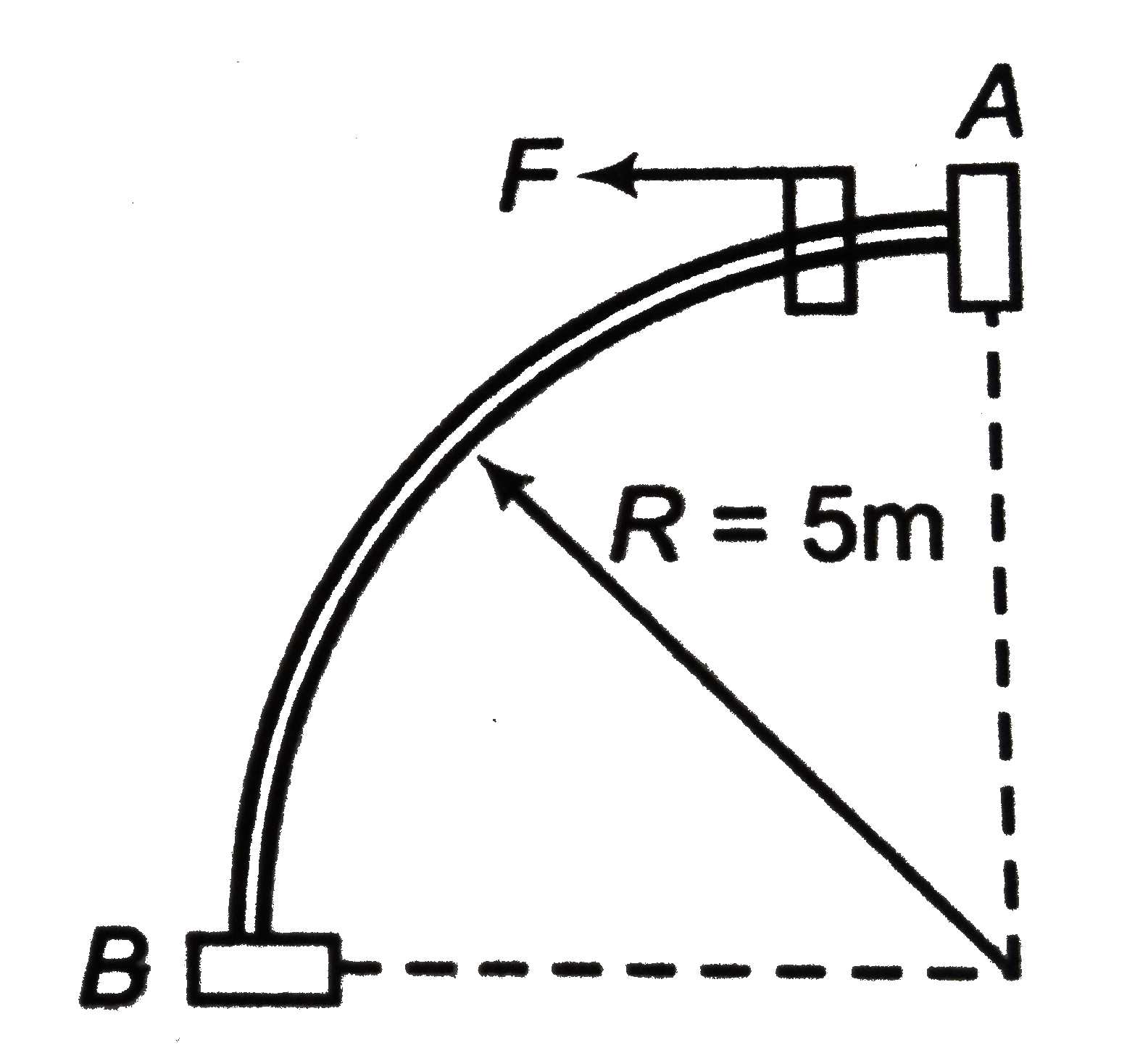
View Solution
