A block is projected along a rough horizontal road with a speed of 10 m/s. If the coefficient of kinetic friction is 0.10, how far will it travel before coming to rest?
To solve the problem of how far a block will travel before coming to rest when projected along a rough horizontal road, we can follow these steps:
Step 1: Identify the given values
- Initial speed (u) = 10 m/s
- Coefficient of kinetic friction (μ) = 0.10
- Acceleration due to gravity (g) = 9.81 m/s² (standard value)
Step 2: Calculate the frictional force
The frictional force (f) acting on the block can be calculated using the formula:
f=μ⋅m⋅g
However, since we are interested in the acceleration (deceleration in this case) caused by friction, we can express it as:
a=μ⋅g
Step 3: Substitute the values
Now, substituting the values into the equation for acceleration:
a=0.10⋅9.81
a=0.981m/s2
Step 4: Apply the third equation of motion
We can use the third equation of motion, which relates initial velocity, final velocity, acceleration, and distance:
v2=u2+2as
Where:
- v = final velocity = 0 m/s (since the block comes to rest)
- u = initial velocity = 10 m/s
- a = -0.981 m/s² (negative because it is deceleration)
- s = distance traveled before coming to rest
Substituting the values into the equation:
0=(10)2+2(−0.981)s
0=100−1.962s
Step 5: Solve for s
Rearranging the equation to solve for s:
1.962s=100
s=1001.962
s≈50.96m
Conclusion
The block will travel approximately 50.96 meters before coming to rest.
---
- Initial speed (u) = 10 m/s
- Coefficient of kinetic friction (μ) = 0.10
- Acceleration due to gravity (g) = 9.81 m/s² (standard value)
The frictional force (f) acting on the block can be calculated using the formula:
f=μ⋅m⋅g
However, since we are interested in the acceleration (deceleration in this case) caused by friction, we can express it as:
a=μ⋅g
Now, substituting the values into the equation for acceleration:
a=0.10⋅9.81
a=0.981m/s2
We can use the third equation of motion, which relates initial velocity, final velocity, acceleration, and distance:
v2=u2+2as
Where:
- v = final velocity = 0 m/s (since the block comes to rest)
- u = initial velocity = 10 m/s
- a = -0.981 m/s² (negative because it is deceleration)
- s = distance traveled before coming to rest
0=(10)2+2(−0.981)s
0=100−1.962s
Rearranging the equation to solve for s:
1.962s=100
s=1001.962
s≈50.96m
The block will travel approximately 50.96 meters before coming to rest.
Topper's Solved these Questions
- FRICTIONBOOK - HC VERMA ENGLISHCHAPTER - FRICTIONEXERCISE - Questions for short Answer
11 Videos
- FRICTIONBOOK - HC VERMA ENGLISHCHAPTER - FRICTIONEXERCISE - Objective -2
5 Videos
- FLUID MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - All Questions
90 Videos
- GRAVITATIONBOOK - HC VERMA ENGLISHCHAPTER - GRAVITATIONEXERCISE - Question for short Answers
18 Videos
Similar Questions
A block of 10kg is pulled at a constant speed on a rough horizontal plane by a force of 20N. Calculate the coefficient of friction ?
View SolutionA block of mass 20kg is pushed with a horizontal force of 90N. If the coefficient of static & kinetic friction are 0.4 & 0.3, the frictional force acting on the block is: (g=10ms−2)
View SolutionA 250 g block slides on aeroug horizontal table. Find the work donen by the frictinal force in bringing the block to rest if it is initially moving at a speed of 40 cm/s. If the friction coefficient between te table and the block is 0.1 how far does the block move before coming to rest?
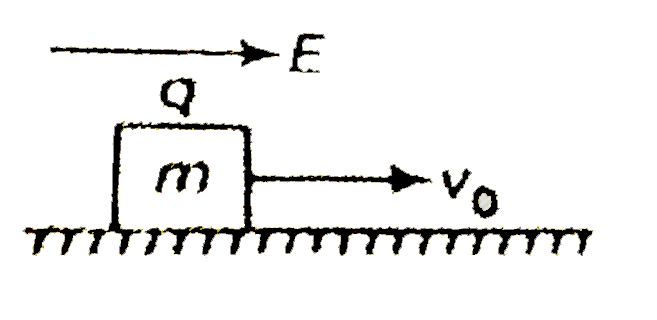
View SolutionIf a charged particle is projected on a rough horizontal surface with speed v0. Find the value of dynamic coefficient of friction, if the kinetic energy of system is constant.
View SolutionA block of mass m starts at rest at height on a frictionless inclined plane. The block slides down the plane, travels across a rough horizontal surface with coefficient of kinetic friction μ and compresses a spring with force constant k a distance x before momentarilly coming to rest. Then the spring extends and the block travels back across the rough surface, sliding up the plane. The block travels a total distance d on rough horizontal surface. The correct experssion for the maximum height h' that the block reaches on its return is :

View SolutionA block of mass m starts at rest at height on a frictionless inclined plane. The block slides down the plane, travels across a rough horizontal surface with coefficient of kinetic friction μ and compresses a spring with force constant k a distance x before momentarilly coming to rest. Then the spring extends and the block travels back across the rough surface, sliding up the plane. The block travels a total distance d on rough horizontal surface. The correct experssion for the maximum height h' that the block reaches on its return is :

View SolutionChoose the correct option:
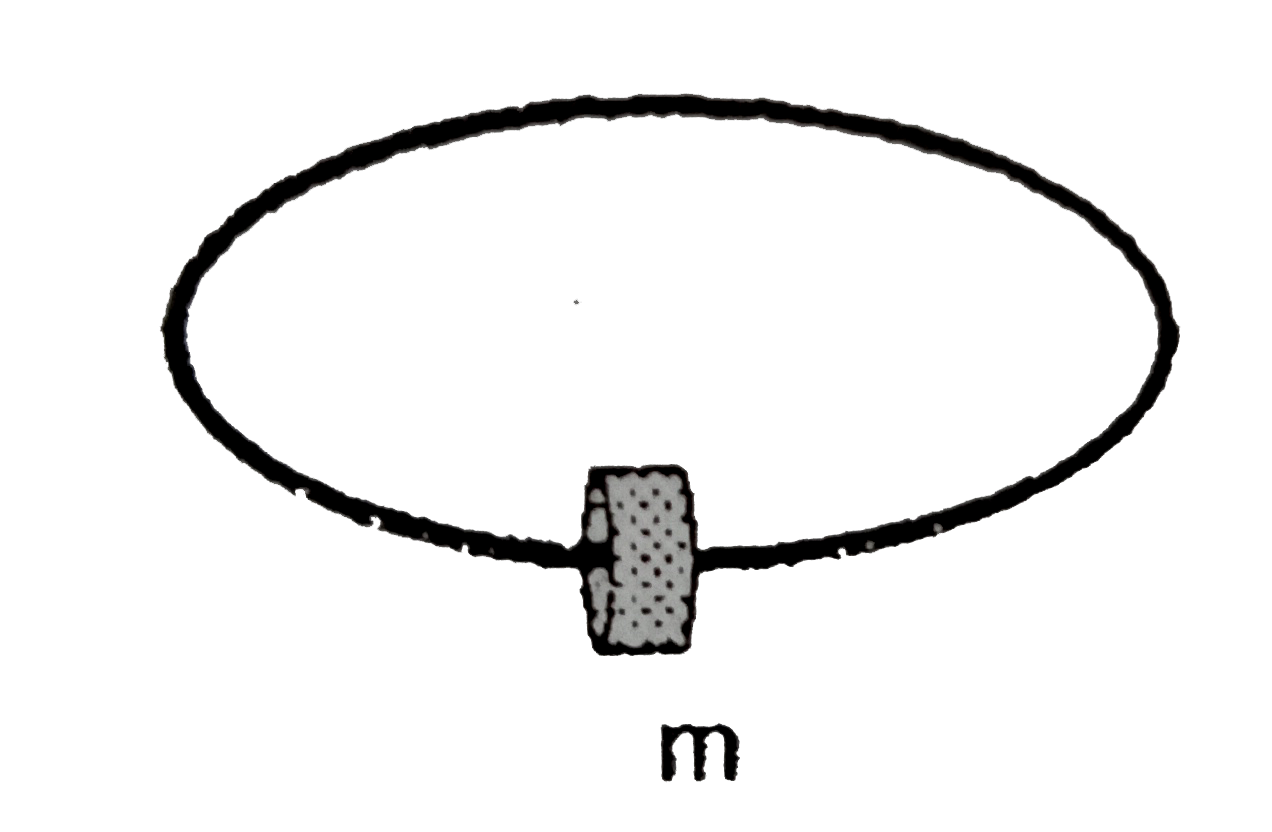
A small collar of mass m is given an initial velocity of magnitude v0 on the horizontal circular track fabricated from a slender rod. If the coefficient of kinetic friction is μK, determine the distance traveled before the collar comes to rest. (Recognize that the friction force depends on the net normal force).
View SolutionA vehicle of mass m is moving on a rough horizontal road with kinetic energy 'E'. If the co-efficient of friction between the tyres and the road be μ. Then the stopping distance is,
View SolutionA block of mass m=5kg is resting on a rough horizontal surface for which the coefficient of friction is 0.2 . When a force F=40N is applied, the acceleration of the block will be (g=10m/s2) .

View SolutionA block of mass 5kg is lying on a rough horizontal surface. The coefficient of static and kinetic friction are 0.3 and 0.1 and g=10ms−2 The frictional force on the block is
View Solution

