Oxygen is filled in a closed metal jar of volume at a pressure of and temperature 400K.The jar has a small leak in it. The atmospheric pressure is Pa and the atmospheric temperature is 300K. Find the mass of the gas that leaks out by time the pressure and the temperature inside the jar equlise with the surrounding.
To solve the problem, we will use the Ideal Gas Law, which states that:
PV=nRT
Where:
- P = Pressure
- V = Volume
- n = Number of moles
- R = Universal gas constant (8.314 J/(mol·K))
- T = Temperature in Kelvin
Step 1: Calculate the initial mass of oxygen in the jar (m1)
Given:
- Initial pressure inside the jar, P1=1.5×105Pa
- Volume of the jar, V1=1.0×10−3m3
- Initial temperature inside the jar, T1=400K
- Molar mass of oxygen, M=32g/mol
Using the Ideal Gas Law, we can express the number of moles n as:
n=P1V1RT1
Now, substituting the values:
n=(1.5×105)×(1.0×10−3)(8.314)×(400)
Calculating n:
n=1503325.6≈0.045mol
Now, we can calculate the mass m1:
m1=n×M=0.045mol×32g/mol≈1.44g
Step 2: Calculate the final mass of oxygen in the jar (m2)
Now, we need to find the mass of oxygen when the pressure and temperature inside the jar equalize with the surroundings.
Given:
- Atmospheric pressure, P2=1.0×105Pa
- Atmospheric temperature, T2=300K
Using the Ideal Gas Law again:
n=P2V1RT2
Substituting the values:
n=(1.0×105)×(1.0×10−3)(8.314)×(300)
Calculating n:
n=1002494.2≈0.040mol
Now, we can calculate the mass m2:
m2=n×M=0.040mol×32g/mol≈1.28g
Step 3: Calculate the mass of gas that leaked out
The mass of gas that leaked out Δm is given by:
Δm=m1−m2
Substituting the values:
Δm=1.44g−1.28g=0.16g
Final Answer
The mass of the gas that leaks out is 0.16 grams.
---
- P = Pressure
- V = Volume
- n = Number of moles
- R = Universal gas constant (8.314 J/(mol·K))
- T = Temperature in Kelvin
- Initial pressure inside the jar, P1=1.5×105Pa
- Volume of the jar, V1=1.0×10−3m3
- Initial temperature inside the jar, T1=400K
- Molar mass of oxygen, M=32g/mol
- Atmospheric pressure, P2=1.0×105Pa
- Atmospheric temperature, T2=300K
Topper's Solved these Questions
Similar Questions
A steel tank contains 300 g of ammonia gas (NH3) at a pressure of 1.35×106 Pa and a temperature of 77∘C . Later the temperature is 22∘C and the pressure is 8.7×105 Pa .
View SolutionThe manometer has a water column difference of 50 cm . If the atmospheric pressure is 105 Pa, find the pressure of the gas in the container .
View Solution
Knowledge Check
A vessel has 6gm of oxygen at pressure P and temperature 400 K.A small hole is made in it so that oxygen leaks out. How much oxygen leaks out if the final pressure is P2 and temperauture 300 K ?
AA. 5 gBB. 4 gCC. 2 gDD. 3 g
A compressed cylinder of gas contains 1.50×103 g of N2 gas at a pressure of 2.0×107 Pa and a temperature of 17.1∘C. What volume of gas has been released into the atmosphere if the final pressure in the cylinder is 1.80×105 Pa ? Assume ideal behaviour and that the gas temperature is unchanged.
View SolutionA glass container encloses a gas a pressure of 8×105 pa and 300 K temperature. The container walls can bear a maximum pressure of 106 Pa. If the temperature of container is gradually increased, find the temperature at which the container will break.
View SolutionA glass container encloses gas at a pressure 4×105 Pa and 300 K temperature. The container walls can bear a maximum pressure of 8×105 Pa. If the temperature of container is gradually increased find temperature at which container will break.
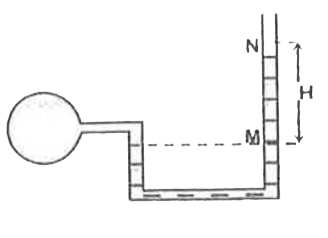
View SolutionA U - tube filled with mercury is connected to bulb containing a gas as shown . If atmospheric pressure is 1.013×105 Pa and H=0.05 m , then
View SolutionA gas at 0∘C and 1 atmospheric pressure occupies 2.5 litres. What change in temperature would be necessary if the pressure is to be adjusted to 1.5 atmospheres and the gas has been transferred to a 2.0 litre container?
View SolutionWhat is the pressure on a swimmer 10m below the surface of lake? g=10ms−2, atmospheric pressure = 1.01×105Pa
View SolutionAt sea level, the atmospheric pressure is 1.04×105 Pa. Assuming g=10m s−2 and density of air to be uniform and equal to 1.3kg m−3, find the height of the atmosphere.
View Solution
