An air bubble of radius 2.0mm is formed at the bottom of a 3.3m deep river. Calculate the radius of the bubble as it comes to the surface. Atmospheric pressure =1.0×105Pa and desnity of water=1000kg m^(-3).
To solve the problem of finding the radius of an air bubble as it rises from the bottom of a river to the surface, we will use the principles of pressure and Boyle's law. Here is a step-by-step solution:
Step 1: Understand the Problem
We have an air bubble formed at a depth of 3.3 m in water with an initial radius of 2.0 mm. We need to find the radius of the bubble when it reaches the surface.
Step 2: Convert Units
Convert the radius from millimeters to meters:
Ra=2.0mm=2.0×10−3m
Step 3: Calculate the Pressure at the Bottom (Point A)
The pressure at the bottom of the river (Point A) can be calculated using the formula:
PA=PB+ρgh
Where:
- PB = atmospheric pressure = 1.0×105Pa
- ρ = density of water = 1000kg/m3
- g = acceleration due to gravity = 10m/s2
- h = depth of the river = 3.3m
Substituting the values:
PA=1.0×105+(1000)(10)(3.3)
PA=1.0×105+33000=1.32×105Pa
Step 4: Apply Boyle's Law
According to Boyle's law, for a gas at constant temperature:
PAVA=PBVB
Where:
- VA = volume of the bubble at Point A
- VB = volume of the bubble at Point B
The volume of a sphere is given by:
V=43πr3
Thus:
VA=43πR3a
VB=43πR3b
Step 5: Set Up the Equation
Substituting the volumes into Boyle's law:
PA(43πR3a)=PB(43πR3b)
The 43π cancels out:
PAR3a=PBR3b
Step 6: Solve for Rb
Rearranging the equation gives:
R3b=PAPBR3a
Taking the cube root:
Rb=(PAPB)1/3Ra
Step 7: Substitute the Values
Substituting the known values:
Rb=(1.32×1051.0×105)1/3×(2.0×10−3)
Rb=(1.32)1/3×(2.0×10−3)
Calculating (1.32)1/3≈1.1:
Rb≈1.1×(2.0×10−3)=2.2×10−3m
Final Answer
The radius of the bubble as it comes to the surface is approximately:
Rb≈2.2mm
---
We have an air bubble formed at a depth of 3.3 m in water with an initial radius of 2.0 mm. We need to find the radius of the bubble when it reaches the surface.
Convert the radius from millimeters to meters:
Ra=2.0mm=2.0×10−3m
The pressure at the bottom of the river (Point A) can be calculated using the formula:
PA=PB+ρgh
Where:
- PB = atmospheric pressure = 1.0×105Pa
- ρ = density of water = 1000kg/m3
- g = acceleration due to gravity = 10m/s2
- h = depth of the river = 3.3m
PA=1.0×105+(1000)(10)(3.3)
PA=1.0×105+33000=1.32×105Pa
According to Boyle's law, for a gas at constant temperature:
PAVA=PBVB
Where:
- VA = volume of the bubble at Point A
- VB = volume of the bubble at Point B
V=43πr3
Thus:
VA=43πR3a
VB=43πR3b
Substituting the volumes into Boyle's law:
PA(43πR3a)=PB(43πR3b)
The 43π cancels out:
PAR3a=PBR3b
Rearranging the equation gives:
R3b=PAPBR3a
Taking the cube root:
Rb=(PAPB)1/3Ra
Substituting the known values:
Rb=(1.32×1051.0×105)1/3×(2.0×10−3)
Rb=(1.32)1/3×(2.0×10−3)
Rb≈1.1×(2.0×10−3)=2.2×10−3m
The radius of the bubble as it comes to the surface is approximately:
Rb≈2.2mm
Topper's Solved these Questions
Similar Questions
An air bubble rises from the bottom of a lake of depth 10.34m to its surface. Compare the pressure on bubble at the bottom to that on surface. (Atmospheric pressure =0.76mofHg, density of Hg=13.6×103Kgm−3 and density of water =103kgm−3).
View SolutionAn air bubble of radius 1 mm is located at a depth of 20 cm below water level. The excess pressure inside the bubble above the atmospheric pressure is [Given, the surface tension of water is 0.075 Nm−1 and density is 1000 kg m−3]
View Solution
Knowledge Check
A cylindrical vessel of height 500mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200mm. Find the fall in height(in mm) of water level due to opening of the orifice.
[Take atmospheric pressure =1.0×105N/m2, density of water=1000kg//m^3andg=10m//s^2. Neglect any effect of surface tension.]A6B4C2D8
The lower end of a capillary tube of diameter 2.0 mm is dipped 8.00cm below the surface of water in a beaker. What is the pressure required in the tube in order to blow a hemispherical bubble at its end in water? The surface tension of water at temperature of the experiments is 7.30×10−2Nm−1. 1 atmospheric pressure =1.01×105Pa, density of water =1000kg/m3,g=9.80ms−2. also calculate the excess pressure.
View SolutionA narrow capillary tube is dipped 10cm below water surface and a liquid bubble of radius 2mm formed at the lower end by blowing air through the tube.
a. Calculate the excess pressure due to surface tension.
b. What is the pressure required in the tube in order to blow a hemispherical bubble at its end in water?
The surface tension of water at temperature of the experiment is 7.30×10−2N/m. 1 atmospheric pressure =105Pa, density of water =1000kg/m3
View SolutionWhat is the pressure on a swimmer 10m below the surface of lake? g=10ms−2, atmospheric pressure = 1.01×105Pa
View SolutionIf the lower end of a capillary tube of radius 2.5 mm is dipped 8 cm below the surface of water in a beaker, then calculate the pressure within a bubble blown at its end in water, in excess of atompheric pressure. [Surface tension of water 72×10−3 N/m]
View SolutionAn air bubble rises the bottom of a lake to the top and increases thereby its volume 15 times . If the atmospheric pressure is 75 cm of hg and density of lake water is 1.02×103kg/m3 then find the depth of lake ( Neglect surface tension).
View SolutionThe U-tube acts as a water siphon. The bend in the tube is 1m above the water surface. The tube outlet is 7m below the water surface. The water issues from the bottom of the siphon as a free jet at atmospheric pressure. Determine the speed of the free jet and the minimum absolute pressure of the water in the bend. Given atmospheric pressure =1.01×105N/m2,g=9.8m/s2 and density of water =103kg/m3
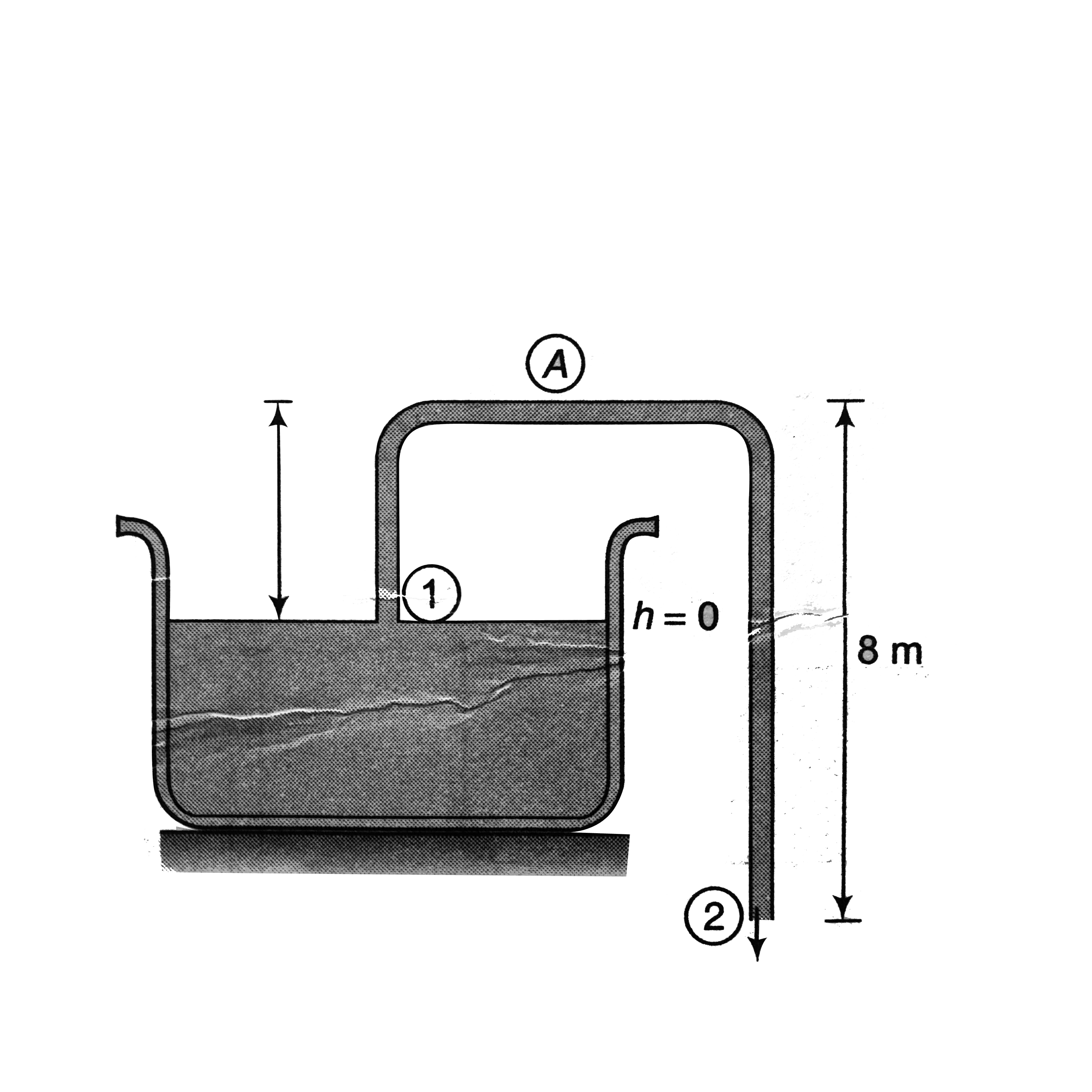 .
.
View SolutionAn air bubble of 20cm3 volume is at the bottom of a lake 40m deep where the temperature is 4∘C. The bubble rises to the surface which is at a temperature of 20∘C. Take the temperature to be the same as that of the surrounding water and find its volume just before it reaches the surface.
View Solution
