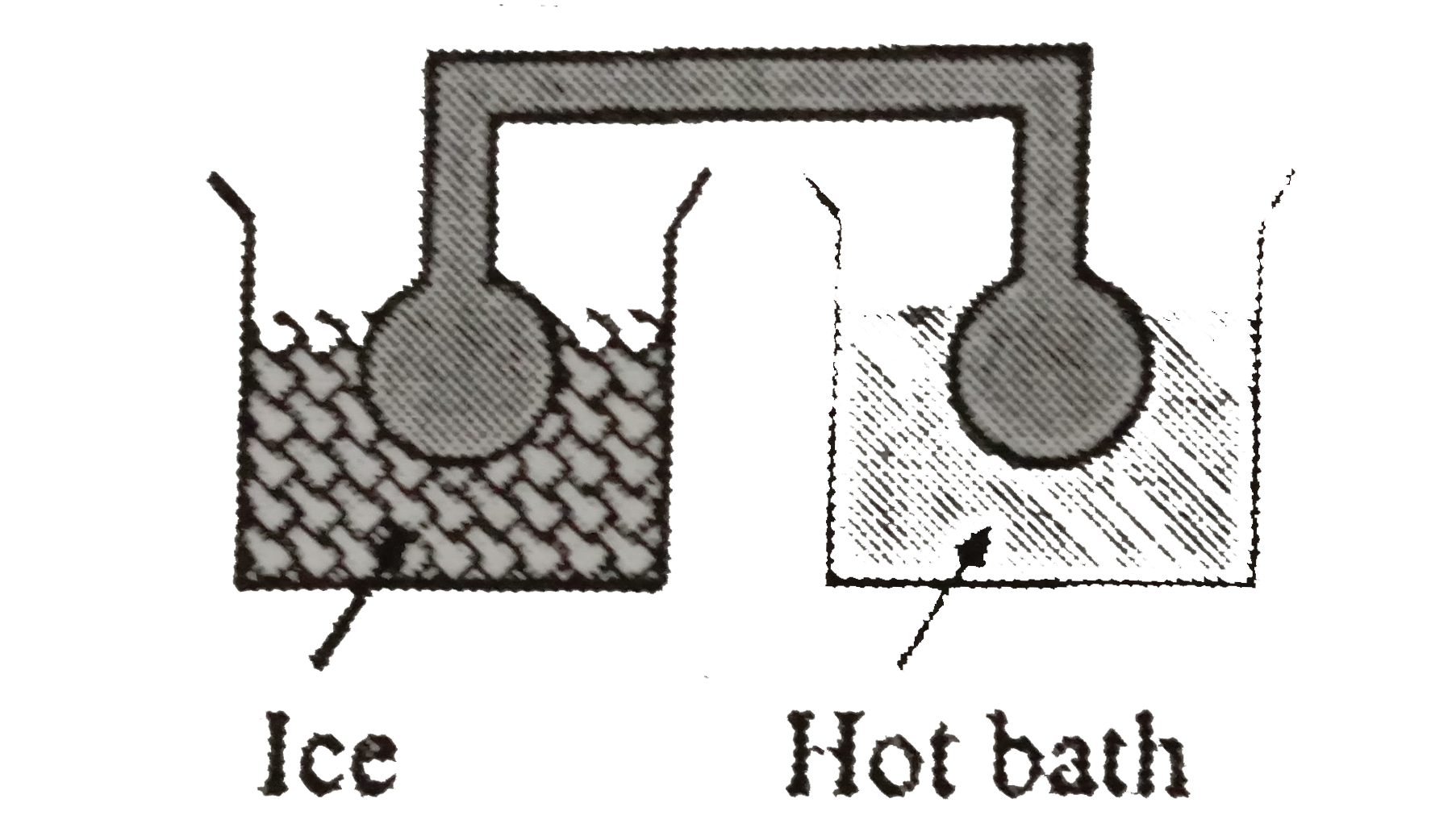
two glass bulbs of equal volume are connected by a narrow tube and are filled with a gas at 0(∘)C at a pressure of 76cm of mercury. One of the bulbs is then placed in melting ice and the other is placed in a water bath maintained at 62∘C.What is the new value of the pressure inside the bulbs? The volume of the connecting tube is neigligible.
To solve the problem, we will use the ideal gas law and the concept of moles of gas in the two bulbs. We will follow these steps:
Step 1: Write down the given values.
- Initial pressure, P1=76cm of Hg
- Initial temperature, T1=0∘C=273K
- Temperature of the first bulb (in melting ice), T2=0∘C=273K
- Temperature of the second bulb (in water bath), T3=62∘C=335K
Step 2: Use the ideal gas law for both bulbs.
The ideal gas law states that:
P1Vn1T1=P2Vn2T2
Where:
- P1 and P2 are the pressures in the first and second bulbs.
- n1 and n2 are the number of moles in the first and second bulbs.
- T1 and T2 are the temperatures in Kelvin.
Since the volume V is the same for both bulbs, we can cancel it out.
Step 3: Set up the equation for the second bulb.
For the second bulb, we can write:
P1n1T1=P2n2T3
Rearranging gives:
P2=P1n2T3n1T1
Step 4: Express n2 in terms of n1.
From the first bulb, we can express the number of moles n2 in terms of n1:
n2=n1−n3
Where n3 is the number of moles in the first bulb after some gas has moved to the second bulb.
Step 5: Substitute n2 into the equation.
Substituting n2 into the equation for P2:
P2=P1(n1−n3)T3n1T1
Step 6: Use the ideal gas law for the first bulb after gas has moved.
For the first bulb:
P1n1=P2n3
Rearranging gives:
n3=P2n1P1
Step 7: Substitute n3 back into the equation for P2.
Substituting n3 into the equation for P2:
P2=P1(n1−P2n1P1)T3n1T1
This simplifies to:
P2=P1n1T3n1T1+P2T3
Step 8: Solve for P2.
Rearranging gives:
P2(T1+T3)=P1T3
Thus:
P2=P1T3T1+T3
Step 9: Substitute the known values.
Substituting the known values:
P2=76cm of Hg×335K273K+335K
Calculating gives:
P2=76×335608≈39.5cm of Hg
Step 10: Final answer.
The new value of the pressure inside the bulbs is approximately 39.5cm of Hg.
---
- Initial pressure, P1=76cm of Hg
- Initial temperature, T1=0∘C=273K
- Temperature of the first bulb (in melting ice), T2=0∘C=273K
- Temperature of the second bulb (in water bath), T3=62∘C=335K
The ideal gas law states that:
P1Vn1T1=P2Vn2T2
Where:
- P1 and P2 are the pressures in the first and second bulbs.
- n1 and n2 are the number of moles in the first and second bulbs.
- T1 and T2 are the temperatures in Kelvin.
For the second bulb, we can write:
P1n1T1=P2n2T3
Rearranging gives:
P2=P1n2T3n1T1
From the first bulb, we can express the number of moles n2 in terms of n1:
n2=n1−n3
Where n3 is the number of moles in the first bulb after some gas has moved to the second bulb.
Substituting n2 into the equation for P2:
P2=P1(n1−n3)T3n1T1
For the first bulb:
P1n1=P2n3
Rearranging gives:
n3=P2n1P1
Substituting n3 into the equation for P2:
P2=P1(n1−P2n1P1)T3n1T1
This simplifies to:
P2=P1n1T3n1T1+P2T3
Rearranging gives:
P2(T1+T3)=P1T3
Thus:
P2=P1T3T1+T3
Substituting the known values:
P2=76cm of Hg×335K273K+335K
Calculating gives:
P2=76×335608≈39.5cm of Hg
The new value of the pressure inside the bulbs is approximately 39.5cm of Hg.
Topper's Solved these Questions
Similar Questions
Two glass bulbs of same volume containing a gas at NTP are connected by a very narrow tube. The pressure is 88.46cm of mercury when one bulb is kept in ice and the other in hot water. Calculate the temperature of the hot water.
View SolutionIn the given figure a glass tube lies horizontally with the middle 20cm containing mercury. The two ends of the tube contains air at 27∘C and at a pressure 76cm of mercuy. Now the air column on one side in maintained at 0∘C and the other side is maintained at 127∘C. Find the new length of the air column on the cooler side. Neglect the changes in the volume of mercury and of the glass.

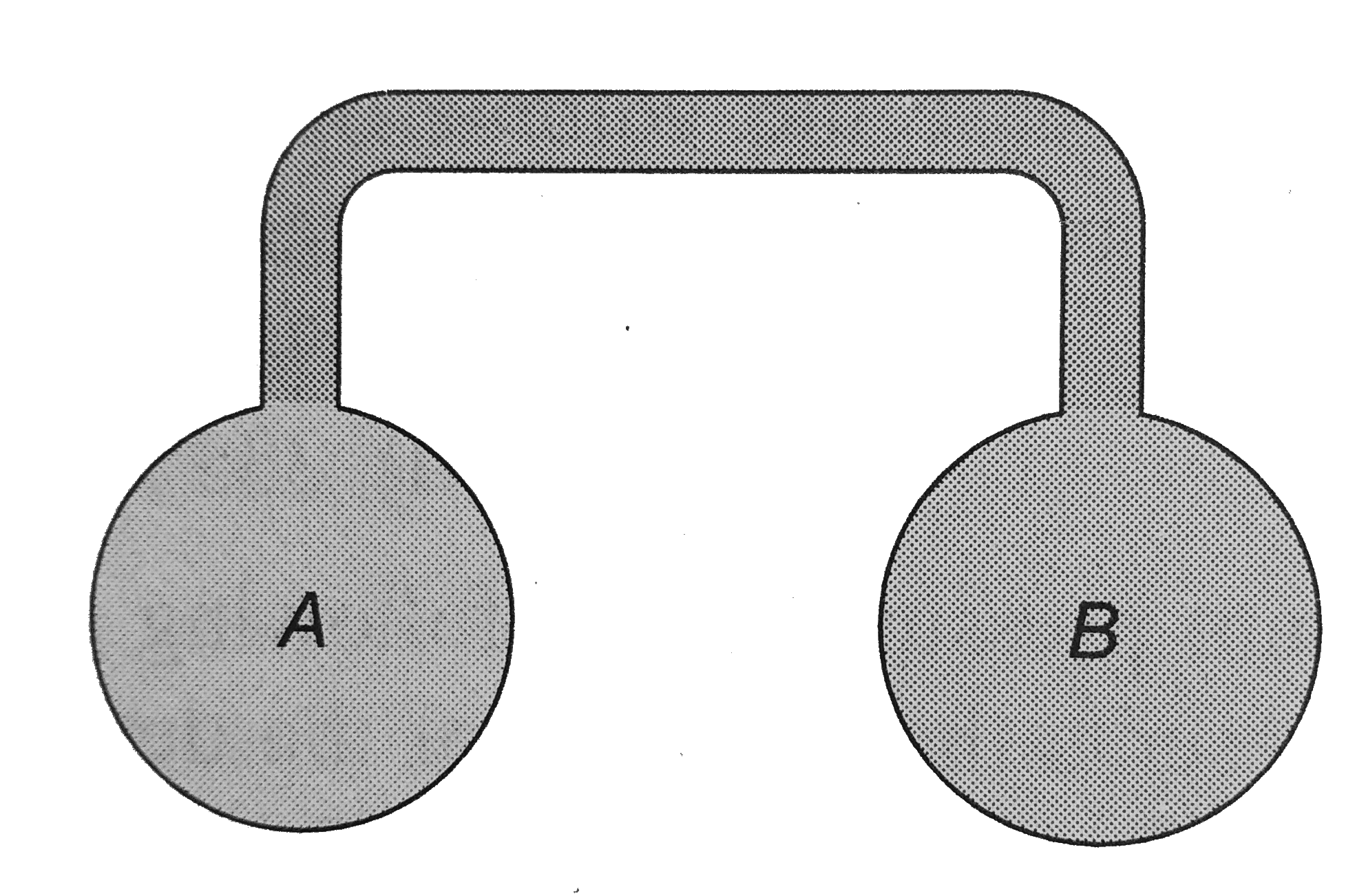
View SolutionTwo spherical vessel of equal volume are connected by a n arrow tube. The apparatus contains an ideal gas at one atmosphere and 300K. Now if one vessel is immersed in a bath of constant temperature 600K and the other in a bath of constant temperature 300K. then the common pressure will be
View Solution120 cm3 of a gas is taken at 27.3 K. The temperature is then raised to 0∘C What is the new volume of the gas ? The pressure is kept constant.
View SolutionA glass tube sealed at both ends is 100cm long, It lies horizontally with the middle 10cm containing mercury. The two ends of the tube contain air at 27∘Cand at a pressure 76cm of mercury. The air column on one side is maintained at 0∘Cand the other side is maintained at 127∘C. Calculate the length of the air column on the cooler side. neglect the changes in the volume of mercury and of the glass.
View SolutionTwo flasks of equal volumes have been joined by a narrow tube of negligible volume. Initially both the flasks are 300 K and totally 9 mole of gas is present. One of the flasks is then placed in a thermostat at 600K. How many moles of gas is present in hot bulb.
View SolutionTwo identical glass bulbs are interconnected by a thin glass tube. A gas is filled in these bulbs at N.T.P. If one bulb is placed in ice and another bulb is placed in hot bath, then the pressure of the gas becomes 1.5 times. The temperature of hot bath will be
View SolutionTwo identical glass spheres filled with air are connected by a thin horizontal glass tube the glass tube contains a pellet of mercury at its mid-point Air in one sphere is at 0∘C and the other is at 20∘C if temperature of both the vessels are increased by 10∘C then neglecting the expansions of the bulbs and the tube
View SolutionThe pressure of the gas in a constant volume gas thermometer is 80cm of mercury in melting ice at 1atm. When the bulb is placed in a liquid, the pressure becomes 160cm of mercury. Find the temperature of the liquid.
View SolutionThe pressure of the gas in a constant volume gas thermometer is 80cm of mercury in melting ice at 1atm. When the bulb is placed in a liquid, the pressure becomes 160cm of mercury. Find the temperature of the liquid.
View Solution
