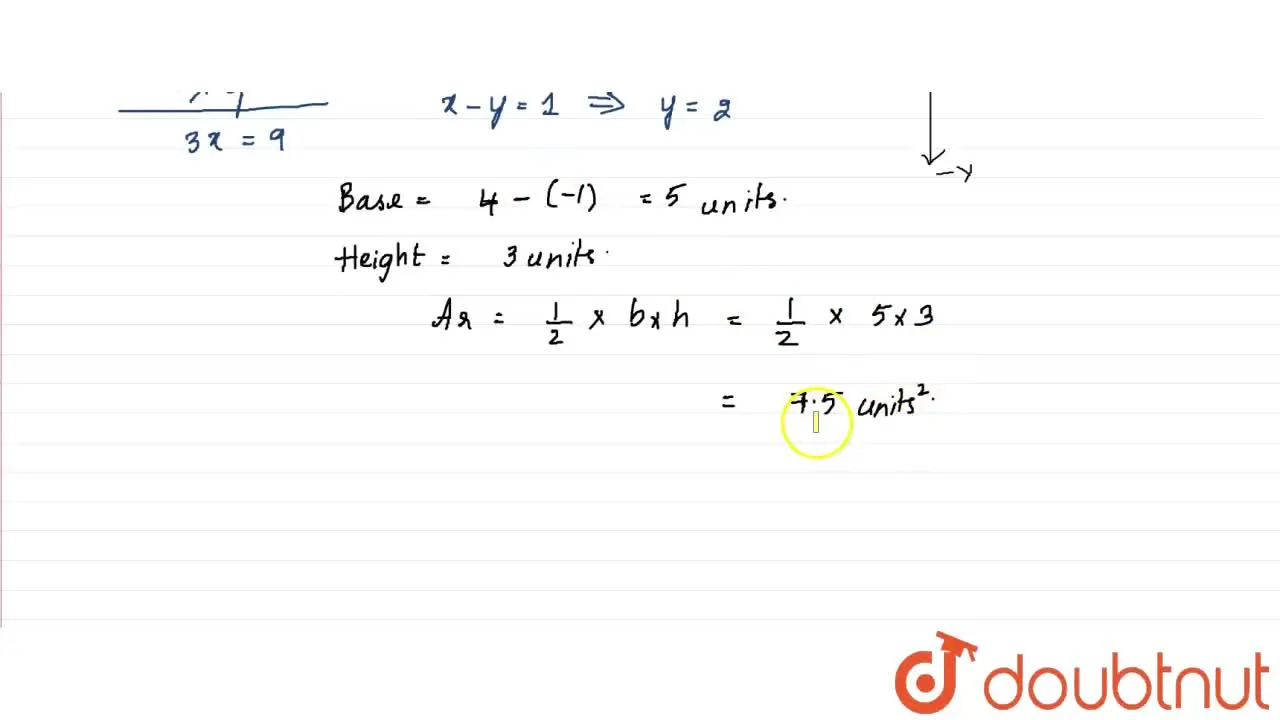
Draw the graphs of the equations x−y=1 and 2x+y=8 . Shade the area bounded by these two lines and y-axis. Also, determine this area.
Step by step video & image solution for Draw the graphs of the equations x-y=1\ a n d\ 2x+y=8 . Shade the area bounded by these two lines and y-axis. Also, determine this area. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Related Playlists
Similar Questions
Solve the following system of linear equations graphically: x+y−11=0, x−y−1=0 . Shade the region bounded by these lines and y-axis. Also, find the area of the region bounded by these lines and y-axis.
View SolutionDraw the graph of the pair of equations 2x+y=4 and 2x-y=4. Write the vertices of the triangle formed by these lines and the y-axis, find the area of this triangle?
View SolutionDraw the graph of the equation 2x+y=6 . Shade the region bounded by the graph and the coordinate axes. Also, find the area of the shaded region.
View SolutionDraw the graphs of 2x+y=6 and 2x−y+2=0 . Shade the region bounded by these lines and x-axis. Find the area of the shaded region.
View SolutionDraw the graphs of the equations x=3, x=5 and 2x-y-4=0. Also find the area of the quadrilateral formed by the lines and the X-axis.
View SolutionThe area bounded by the curve y=4−x2 and X-axis is
View SolutionDraw the graphs of 2x+y=6andx−y+2=0. Shade the region boun-ded by these lines and x=axis. Find the area of the shaded region.
View SolutionFind the area bounded by the curve |x|+y=1 and axis of x.
View SolutionThe area bounded by the curve x=y2+4y with y-axis is
View SolutionFind the area bounded by the lines y=4x+5,y=5−xand4y=x+5.
View Solution