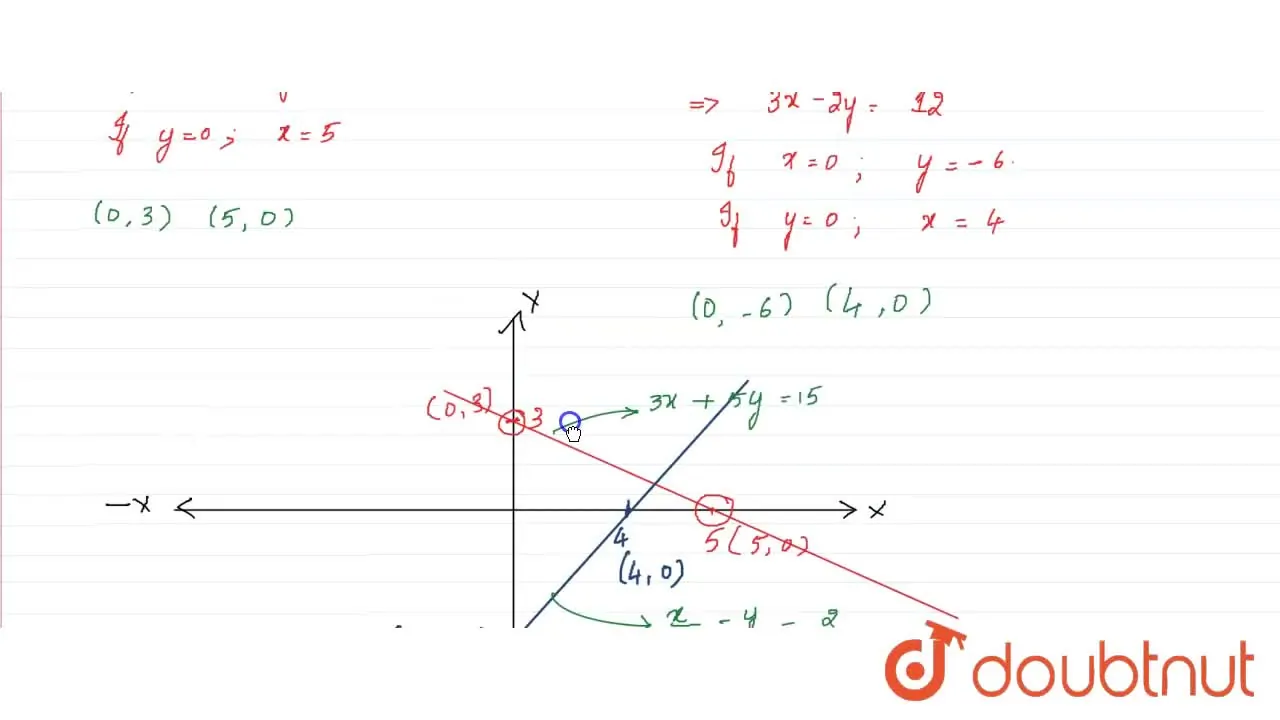
Draw the graph of each of the following linear equations in two variables: 3x+5y=15 (ii) x2−y3=2
Step by step video & image solution for Draw the graph of each of the following linear equations in two variables: 3x+5y=15 (ii) x/2-y/3=2 by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Related Playlists
Similar Questions
Draw the graph of each of the following linear equations in two variables: −x+y=6 (ii) y=2x
View SolutionDraw the graph of each of the following linear equations in two variables: x−23=y−3 (ii) 2y=−x+1
View SolutionDraw the graph of each of the following linear equations in two variables:
(i) x+y=4 (ii) x−y=2
(iii) y=3x (iv) 3=2x+yView SolutionDraw the graph of each of the following linear equations in two variables:(i) x+y=4 (ii) x−y=2 (iii) y=3x (iv) 3=2x+y
View SolutionDraw the graphs of each of the following linear equation in Cartesian plane: y=1
View SolutionWrite each of the following equation in two variables:
(i) 2x=3
(ii) y=3View SolutionWrite each of the following equation in two variables:
(i)5x=72
(ii) y=32xView SolutionDraw the graphs of each of the following linear equations: x−2=0,x+5=0,2x+4=3x+1
View SolutionWrite each of the following as an equation in two variables:(i) x=−5 (ii) y=2 (iii) 2x=3 (iv) 5y=2
View SolutionWrite each of the following as an equation in two variables x and y
(i)x=−3
(ii) y=4View Solution