Using the relation 2(1−cosx)<x2 ,x=0 or prove that sin(tanx)≥x,∀ϵ[0,π4]
Give that 2(1−cosx)<x2,x≠0
To prove sin (tanx)≥x,x∈[0,π/4], let us consider
f(x)=sin(tanx )-x
f(x) =cos (tanx ) sec2x−1
=cos(tanx)−cos2xcost(2)x
sin2x1−(1)2cos2x(cost(2))x
=sin2xcos2x2cos4x>0∀x∈[0,[π/4]
Therefore f(x)>0 .Thus f(x) is an increasing function
so ,for x in [0,π/4]we have
x≥0 or f(x)≥f(0)
sin(tanx)−x≥sin(tan0)−0
sin(tanx)−x≥0
sin(tanx)≥x
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSEXERCISE - Concept Application Exercise 6.110videos
MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSEXERCISE - Concept Application Exercise 6.210videos
MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSEXERCISE - Matrix Match Type14videos
MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - METHODS OF DIFFERENTIATIONEXERCISE - Single Correct Answer Type46videos
METHODS OF DIFFERENTIATION
- BOOK - CENGAGE ENGLISHCHAPTER - MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSEXERCISE - Comprehension Type6videos
MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONS
Similar Questions
The number of solutions of the equation secx1−cosx=11−cosx in [0, 2π] is equal to 3 (b) 2 (c) 1 (d) 0
View SolutionSolutions of the equations (2cosx−1)(3cosx+4)=0 is [0,2π] is:
View SolutionIntegrate the functions1−cosx1+cosx
View SolutionIntegrate the functionscosx1+cosx
View SolutionUsing constraint method find the relation between of a1 and a2.
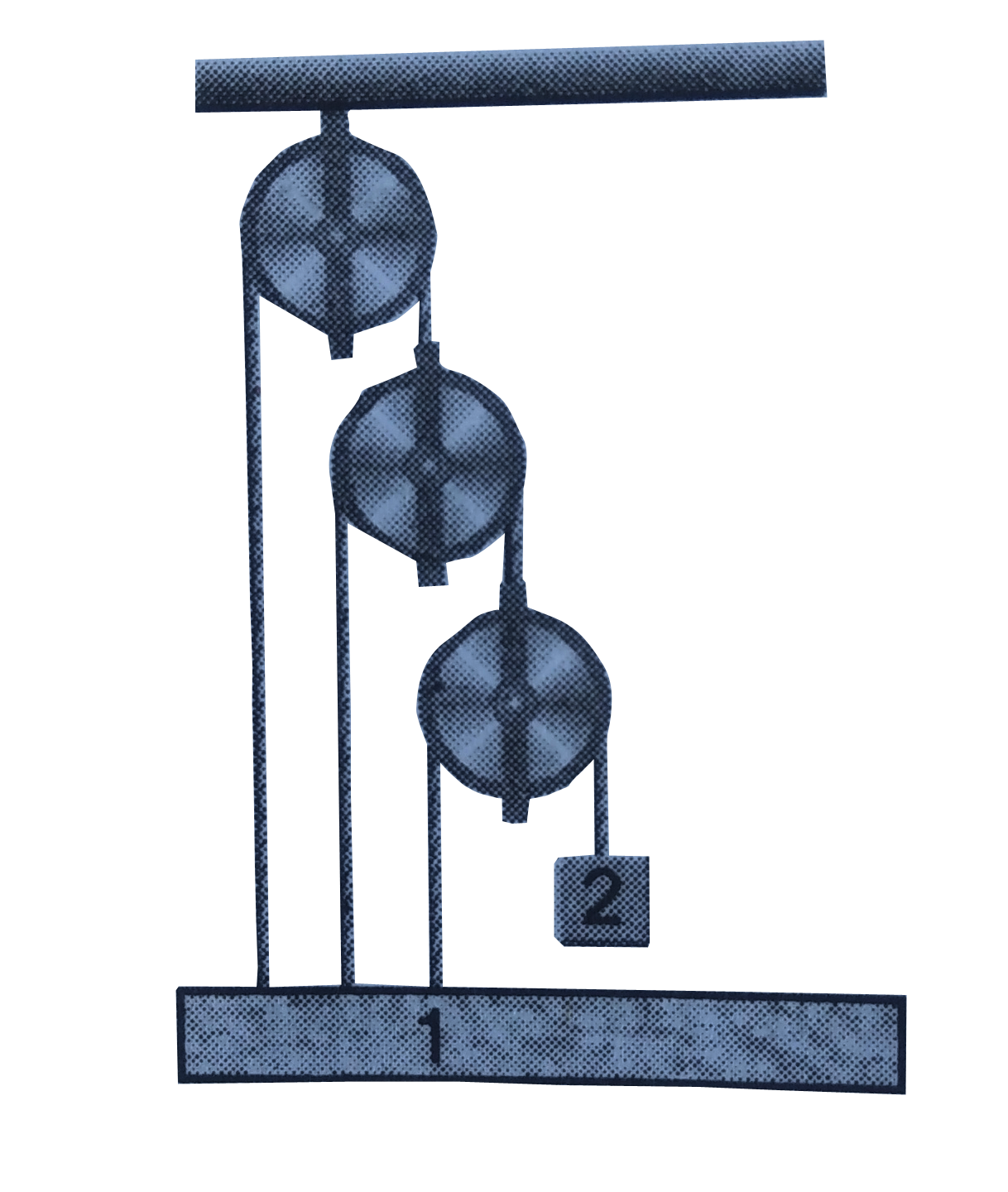 View Solution
View SolutionUsing constraint method find the relation between accelerations of 1 and 2.
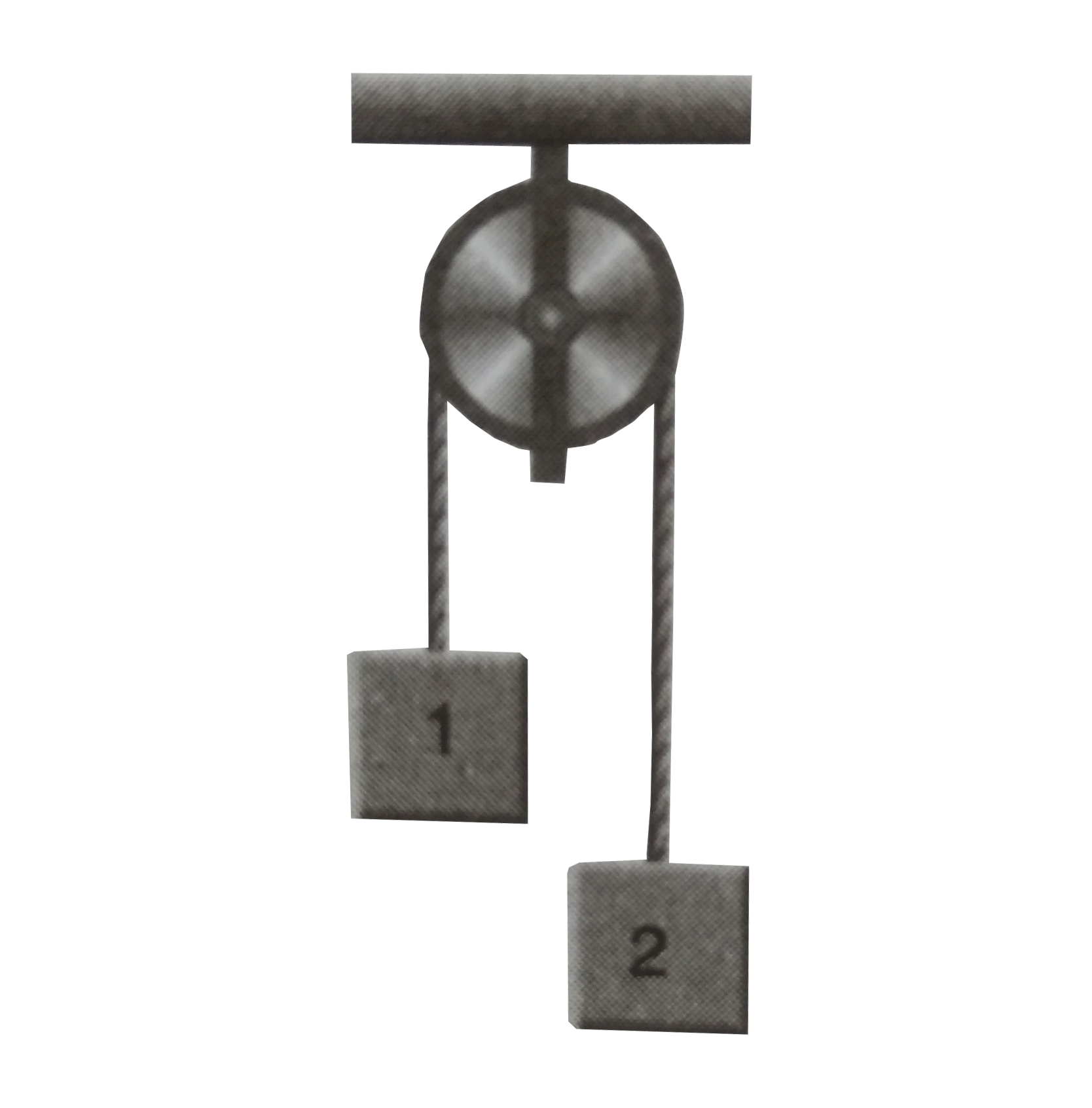
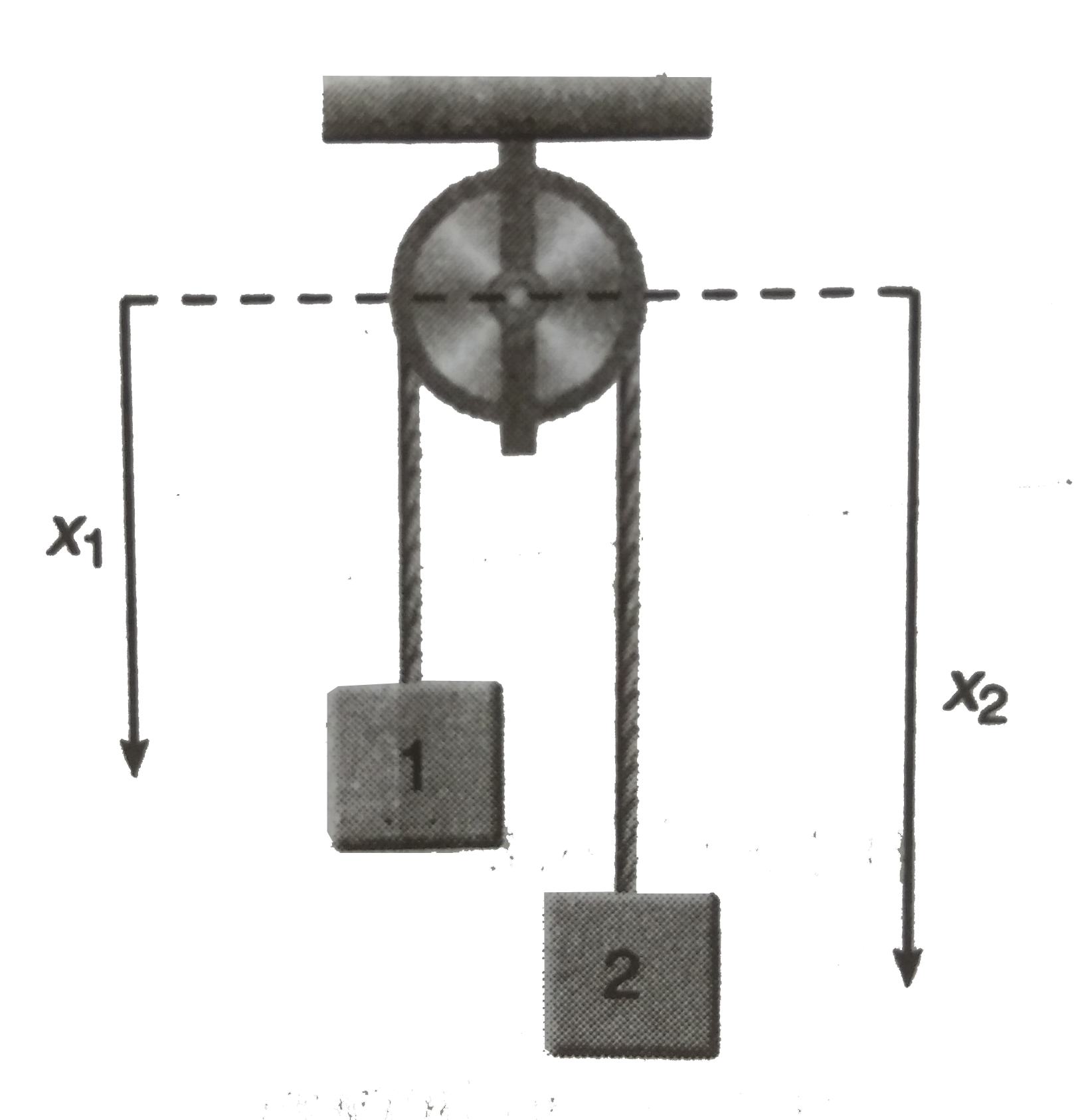 View Solution
View SolutionUsing contraint equation. Find the relation between a1anda2.
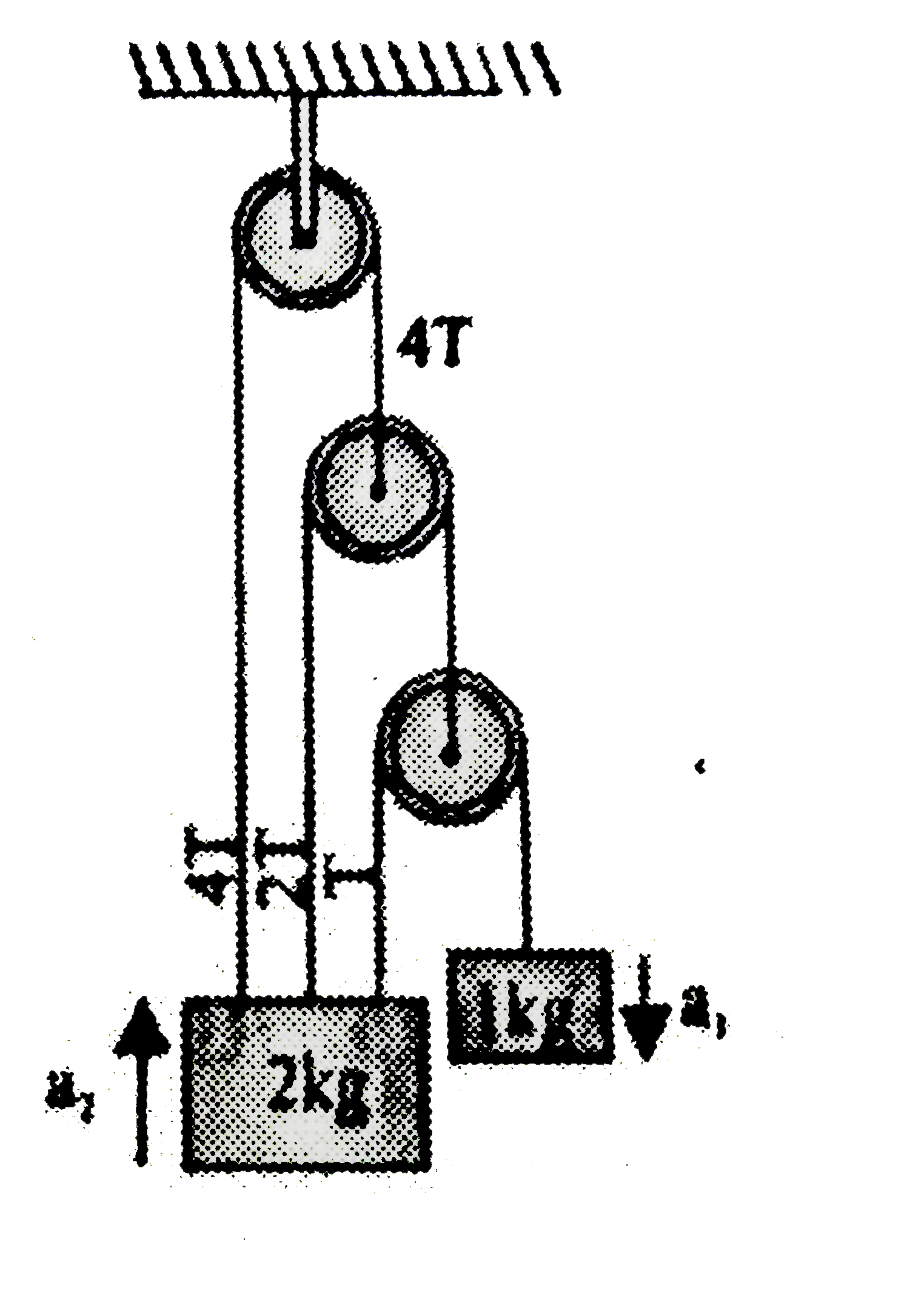 View Solution
View SolutionFind the solution of cosx=12.
View SolutionIf x1,x2,...,xn are real numbers, then the largest value of the expression
sinx1cosx2+sinx2cosx3+...+sinxncosx1, isView Solutionsolve equation 2tan−1(cosx)=tan−1(2cosecx)
View Solution
