Using constraint method find the relation between accelerations of 1 and 2.
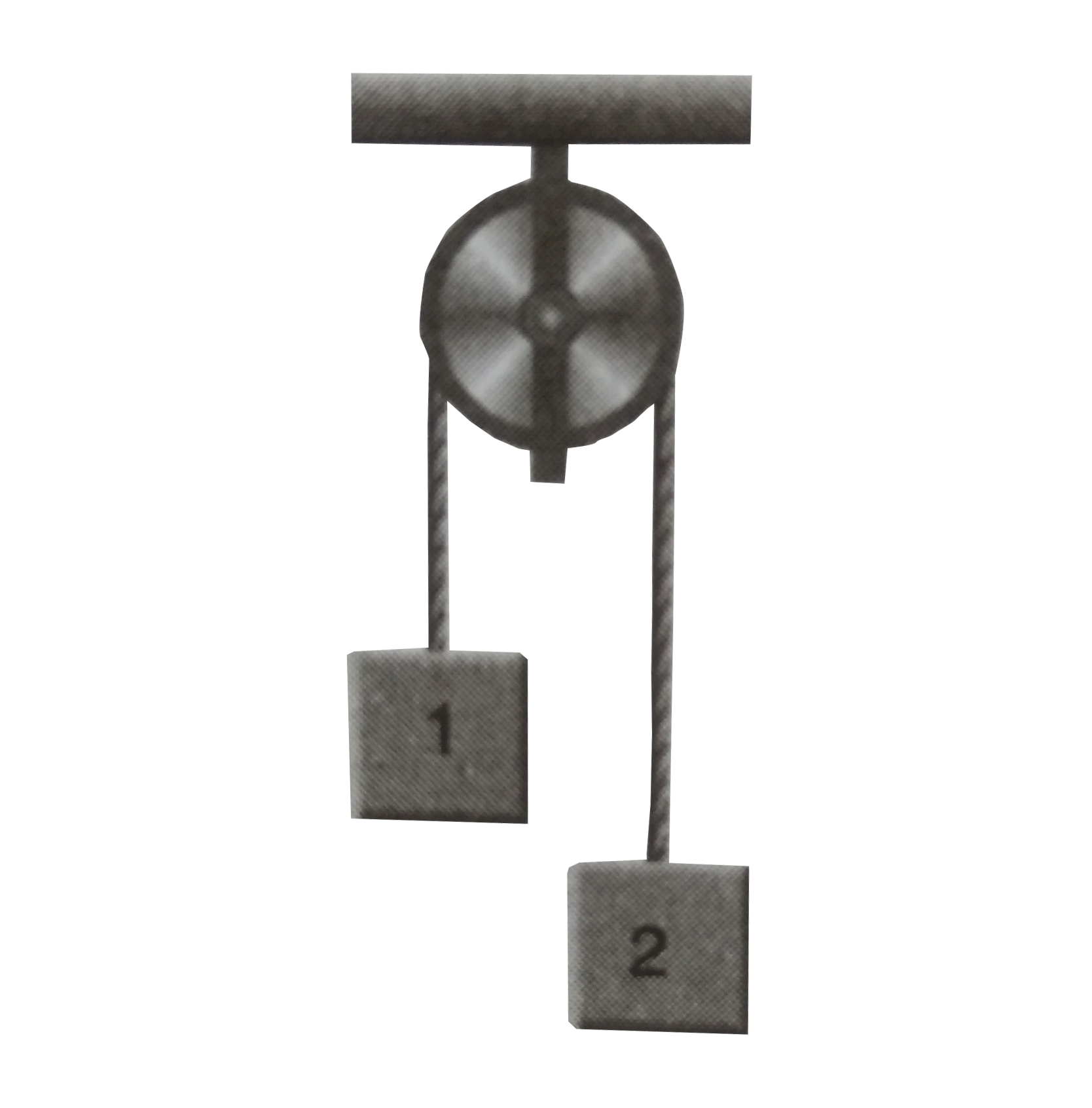
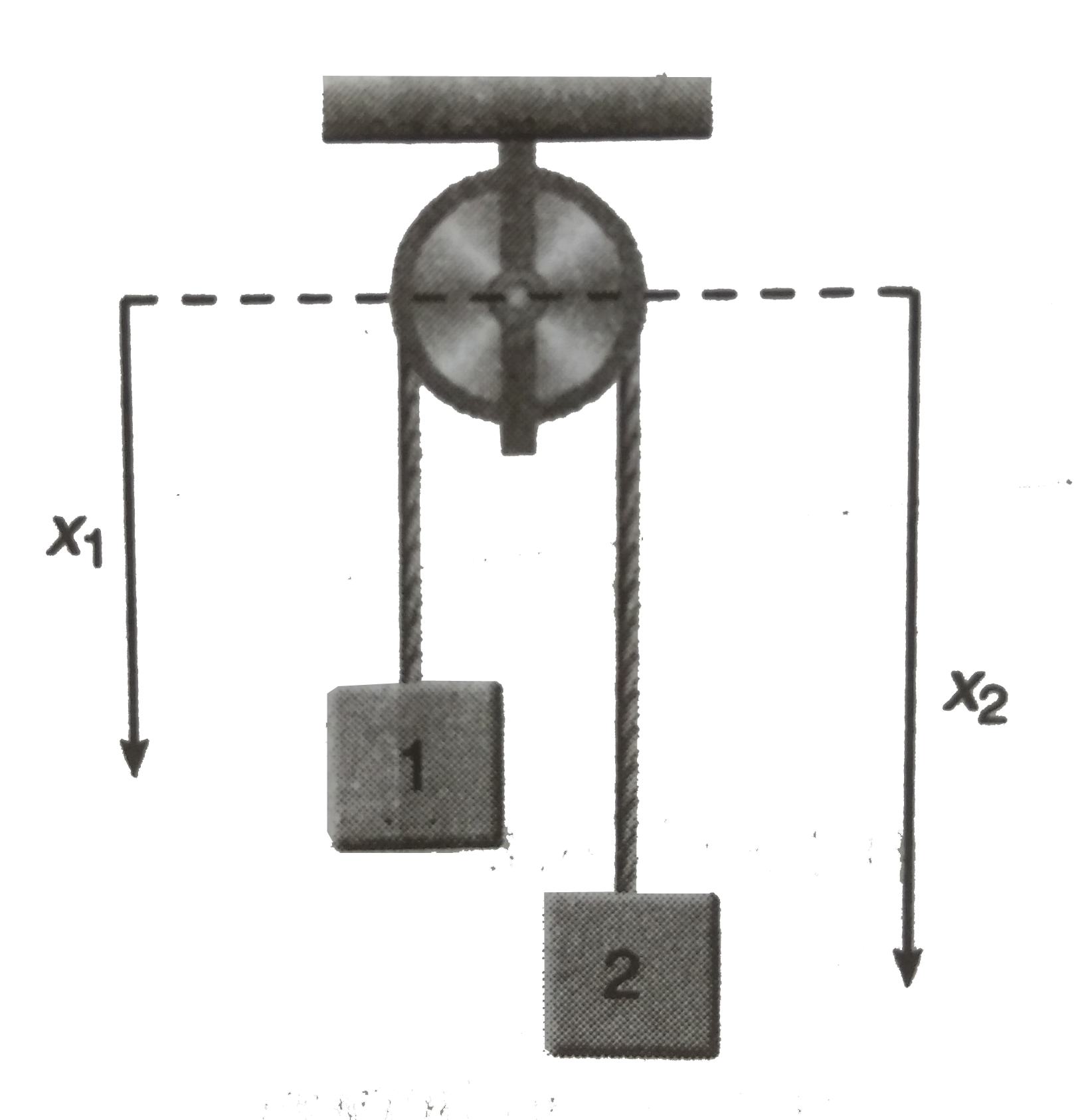
At any instant of time Let x1 and x2 be the displancements of 1 and 2 from line (shown dotted). Here x1 and x2 are variables but,
or x1+x2=1 (length of string)
Differentiating with respect to time, we have
v1+v2=0 or v1=−v2
Again differentiating with respect to time, we get
a1+a2=0 or a1=−a2
This is the required relation between a1 and a2, i.e. accelrations of 1 and 2 are equal but in opposite directions.
Topper's Solved these Questions
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Solved Examples
Explore 15 Videos
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Miscellaneous Examples
Explore 9 Videos
- KINEMATICS 1Book:DC PANDEY ENGLISHChapter:KINEMATICS 1Exercise:INTEGER_TYPE
Explore 15 Videos
- LAWS OF THERMODYNAMICSBook:DC PANDEY ENGLISHChapter:LAWS OF THERMODYNAMICSExercise:Level 2 Subjective
Explore 18 Videos
Similar Questions
Find the relation between acceleration a1 and a2
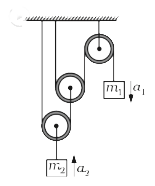
View SolutionUsing constraint method find the relation between of a1 and a2.
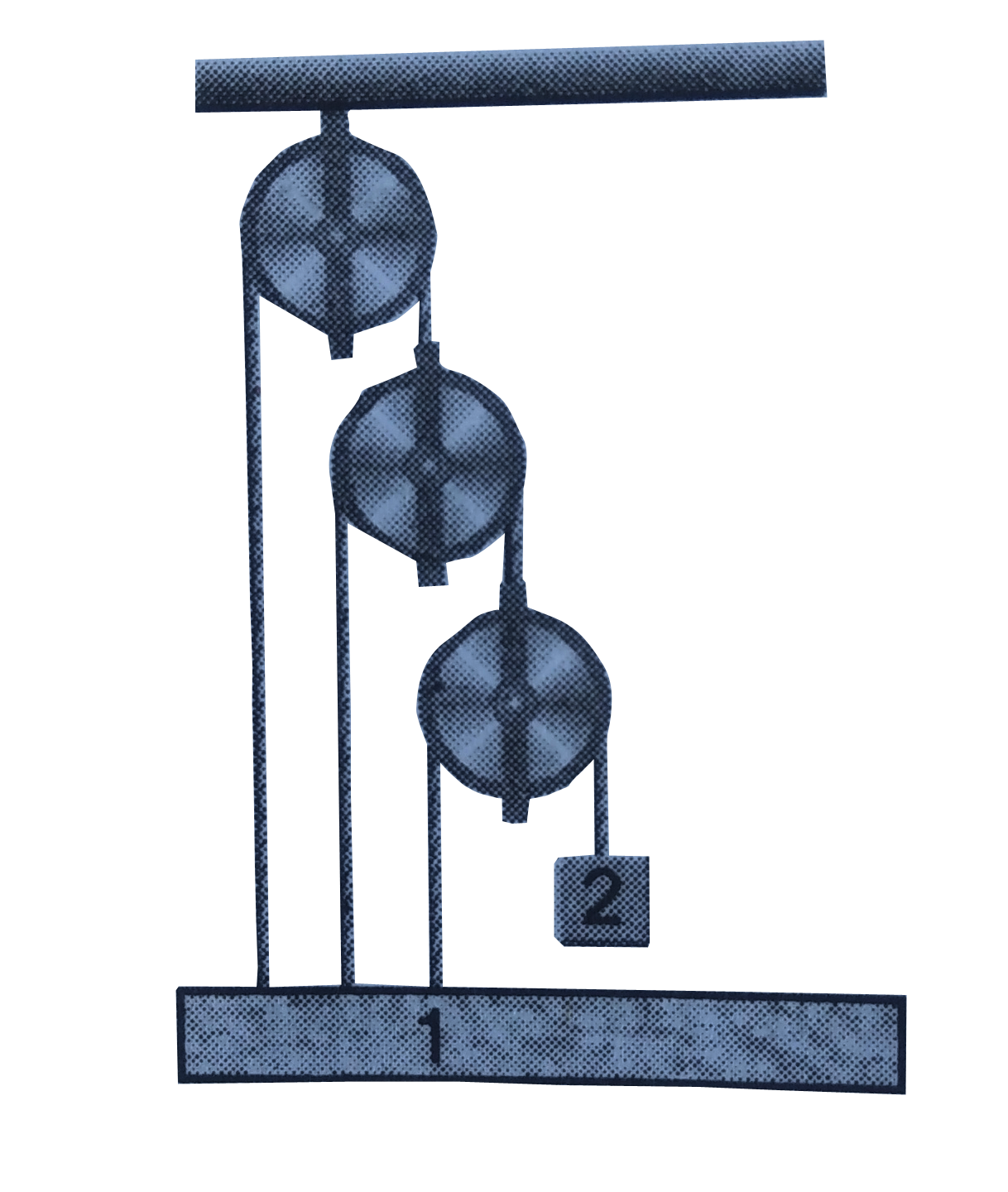
View SolutionFind the relation between acceleration of blocks a1,a2 and a3.

View SolutionUsing contraint equation. Find the relation between a1anda2.
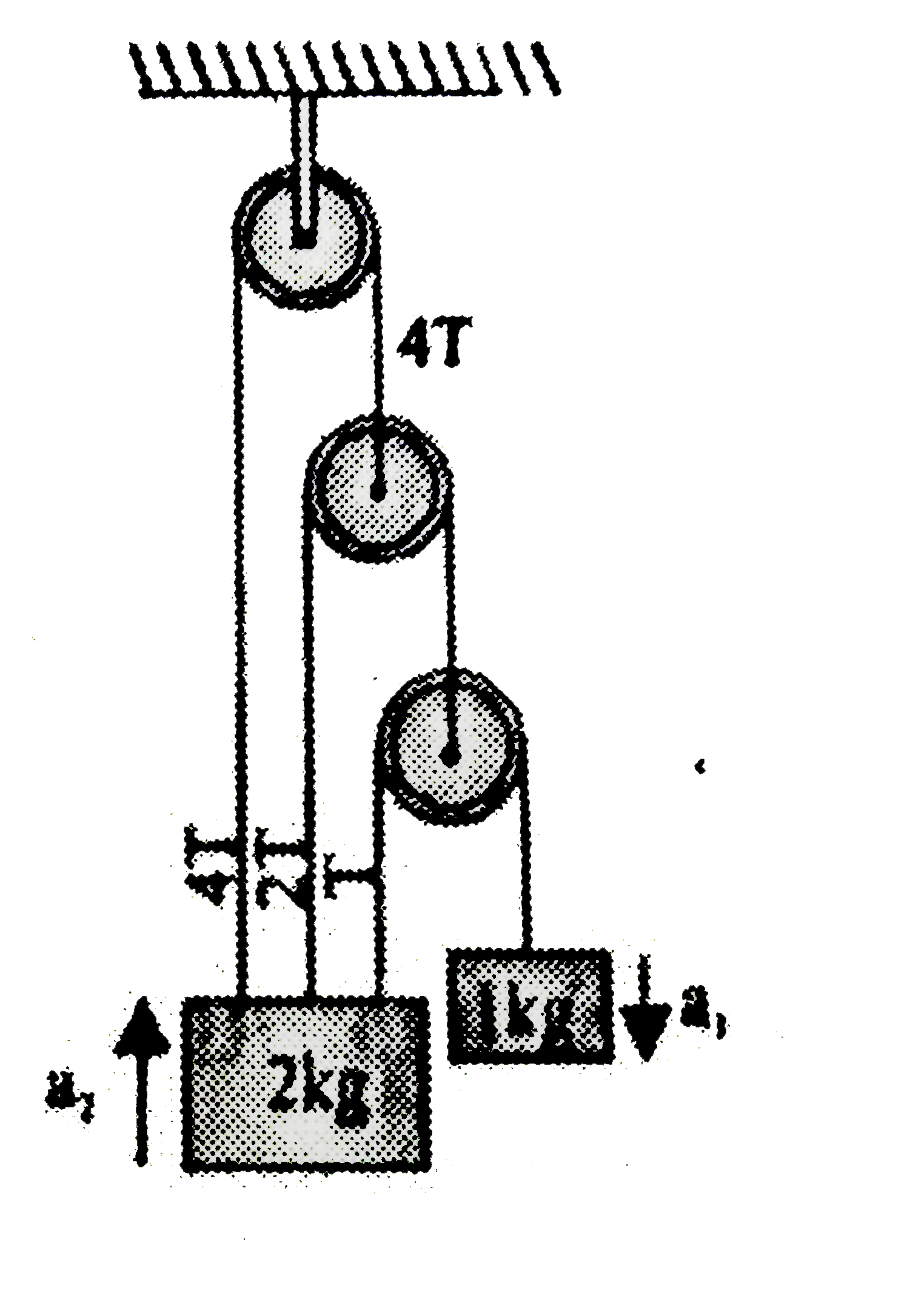
View SolutionIn the given diagram , find the relation between acceleration of blocks m1andm2 . (m2 remains horizontal ).
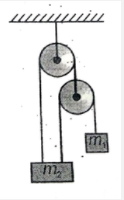
View SolutionIn the pulley-block arrangement shown in figure , find the relation between acceleration of blocks A and B

View SolutionIn the pulley-block arrangement shown in figure , find the relation between acceleration of blocks A and B

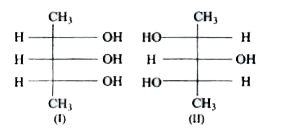
View SolutionFind out relation between I and II :
View SolutionFind the relation in the accelerations of the three masses shown in fig.
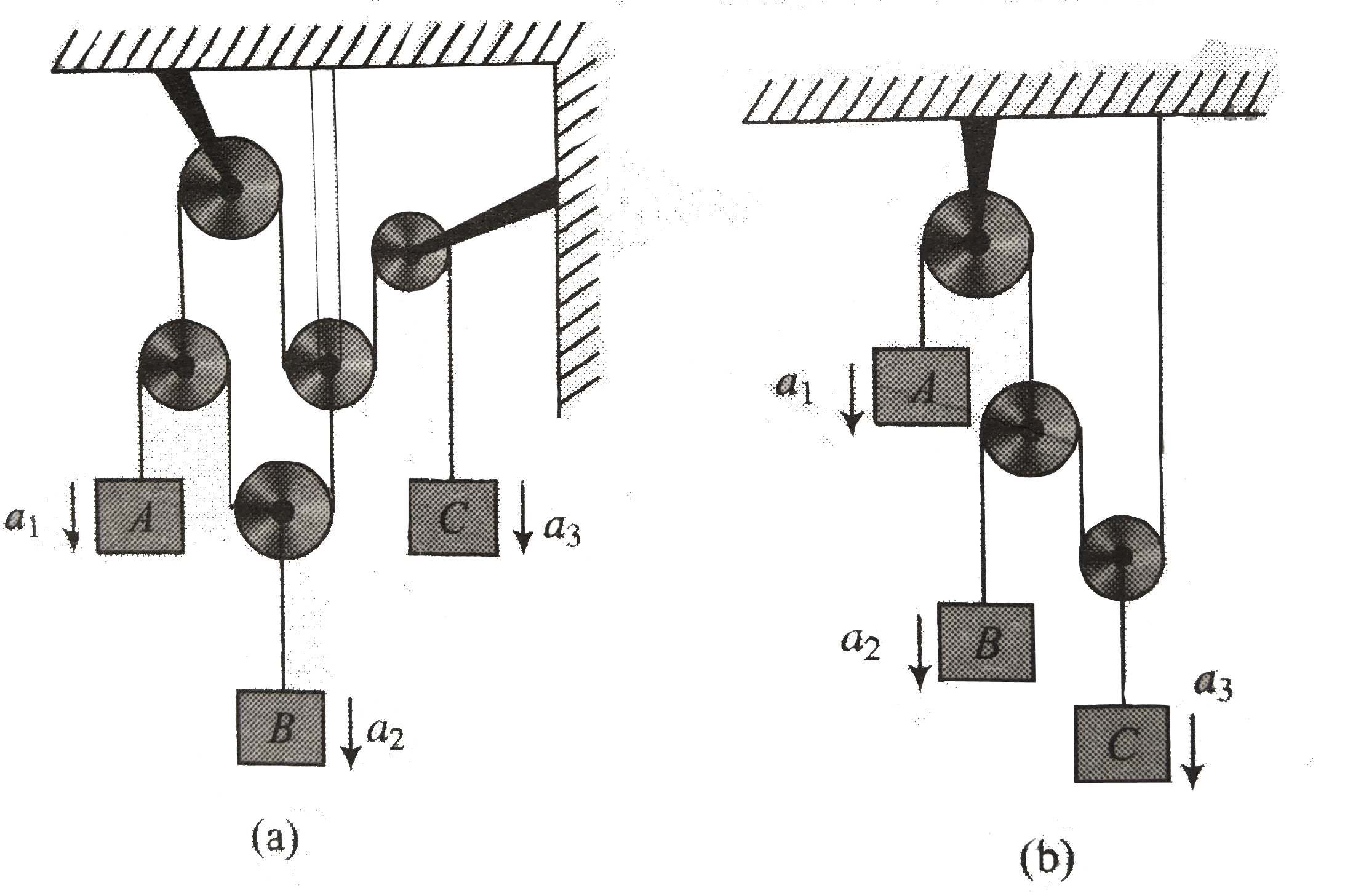
View SolutionIn the figure shown the relation between the accelerations of A B & C is (here a = accelerations of A relative to C)

View Solution

