Answer the following:
(a) Derive an expression for the potential energy of an electric dipole in a uniform electric field. Explain conditions for stable and unstable equilibrium.
(b) Is the electrostatic potential necessarily zero at a point where the electric field is zero ? Give an example to support your answer.
(a) Let an electric, dipole of dipole moment →P be placed in an electric field →E making an angle θ with the direction of electric field intensity →E.
The torque acting on the dipole is given by :
→τ=→P×→E
τ=PEsinθ
work done to rotate the dipole through an angle dthη
dω=τdθ=PEsinθdθ
work done to rotate the dipole from θ1 to θ2
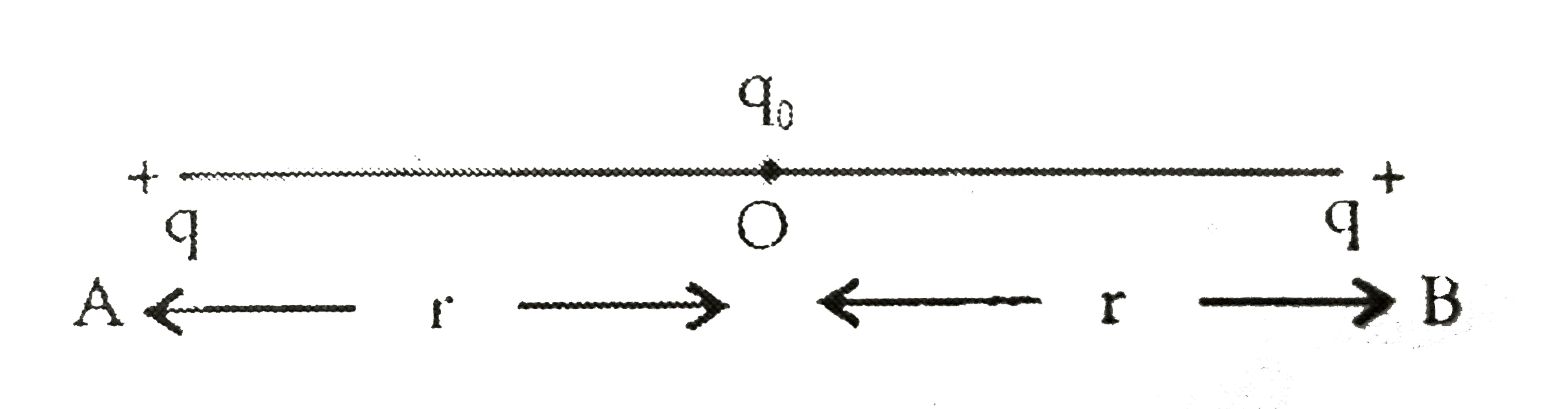
W=int_(theta_(1))^(theta_(2))PE sin theta d theta=PE[-cos theta]_(theta_(1))^(theta_(2))=-PE(cos theta_(2)-cos theta_(1))<br>"If "theta_(1)=90^(@) and theta_(2)=theta" "U=-PE cos theta =vecP.vecE<br>forstab≤equilibriumtheta=0^(@) rArr tau = 0 rArr U=-PE<br>forunstab≤equilibriumtheta=180^(@)rArr tau =0 rArr U = + PE.<br>(b)N⊙<br>ForExamp≤:Letwehavetwopositivechar≥sofsamemagnitudeareplacedatp∮AandBrespectively.E≤ctricfieldatmntOiszerobutthee≤ctrostaticpotentialis<br>V=(1)/(4pi in_(0))(q)/(r)xx2<br>V=(1)/(4pi in_(0))(2q)/(r).
Topper's Solved these Questions
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SECTION - E
5 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SECTION - C
10 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SECTION - C
15 Videos
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D
6 Videos
Similar Questions
Which orientation of an electric dipole in a uniform electric field would correspond to stable equilibrium ?
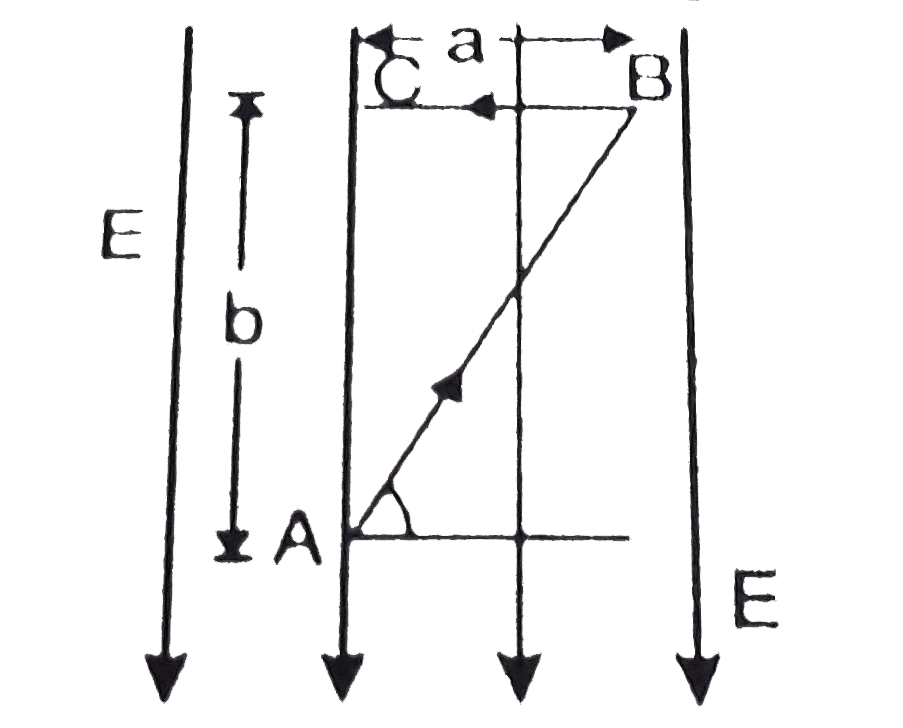
View SolutionThe potential difference points a and B in the given uniform electric field is :
View SolutionDerive the expression for the electric potential at any point along the axial line of an electric dipole.
View SolutionDerive an expression for potential energy of a system of two charges in the absence of the external electrical field.
View SolutionAt what angle of electric dipole moment with uniform electric field, potential energy is maximum ?
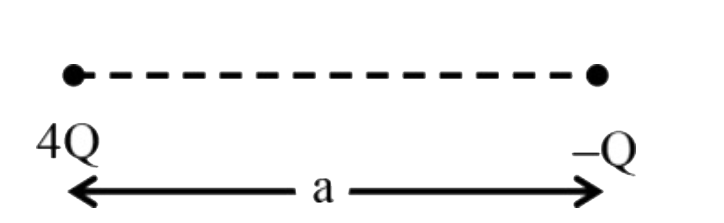
View SolutionThe position of the point where the net electric field will be zero is
View SolutionIn an electric dipole, at which point is the electric potential zero?
View Solution(i) Derive the expression for electric field at a point on the equatorial line of an electric dipole.
(ii) Depiet the orientation of the dipole in (i) stable, (ii) unstable equilibrium in a uniform electric field.
View SolutionA,B and C are three points in a unifrom electric field. The electric potential is

View SolutionAt what angle between dipole moment and electric field, electric dipole remains in stable equilibrium in uniform electric field ?
View Solution
