State the Lorenz's force and express it in vector form. Which pair of vectors are always perpendicular to each other? Derive the expression for the force acting on a current carrying conductor of length L in a uniform magnetic field 'B'.
Step-by-Step Solution
Step 1: State the Lorentz Force
The Lorentz force is the force experienced by a charged particle moving in an electromagnetic field. It is given by the equation:
F=q(E+v×B)
where:
- F is the Lorentz force,
- q is the charge of the particle,
- E is the electric field,
- v is the velocity of the charged particle,
- B is the magnetic field.
Step 2: Express the Lorentz Force in Vector Form
The vector form of the Lorentz force can be simplified to:
F=qv×B
This indicates that the force is perpendicular to both the velocity vector v and the magnetic field vector B.
Step 3: Identify Perpendicular Vectors
From the Lorentz force equation, we can conclude that:
- The magnetic force F is always perpendicular to both the velocity vector v and the magnetic field vector B.
- Therefore, the pair of vectors that are always perpendicular to each other are F (the magnetic force) and v (the velocity of the charged particle), as well as F and B (the magnetic field).
Step 4: Derive the Expression for Force on a Current-Carrying Conductor
Consider a straight conductor of length L carrying a current I placed in a uniform magnetic field B. The force acting on the conductor can be derived as follows:
1. Identify the Charge Carriers: In a current-carrying conductor, the moving charge carriers (usually electrons) experience a magnetic force. The current I can be expressed in terms of the charge density n (number of charge carriers per unit volume), the cross-sectional area A, and the drift velocity vd:
I=n⋅A⋅e⋅vd
where e is the charge of an electron.
2. Force on a Single Charge Carrier: The force on a single charge carrier (electron) moving with drift velocity vd in a magnetic field B is given by:
F=q(vd×B)
For an electron, q=−e:
F=−e(vd×B)
3. Total Force on the Conductor: For a small segment of the conductor of length dL, the total force can be expressed as:
dF=n⋅A⋅e⋅vd⋅dL⋅Bsin(θ)
where θ is the angle between vd and B.
4. Integrate Over Length L: The total force F acting on the entire length L of the conductor is:
F=I⋅L⋅Bsin(θ)
If L is in the direction of the current and B is perpendicular to L, then:
F=I⋅L⋅B
Final Expression
Thus, the force acting on a current-carrying conductor of length L in a uniform magnetic field B is given by:
F=IL×B
The Lorentz force is the force experienced by a charged particle moving in an electromagnetic field. It is given by the equation:
F=q(E+v×B)
where:
- F is the Lorentz force,
- q is the charge of the particle,
- E is the electric field,
- v is the velocity of the charged particle,
- B is the magnetic field.
The vector form of the Lorentz force can be simplified to:
F=qv×B
This indicates that the force is perpendicular to both the velocity vector v and the magnetic field vector B.
From the Lorentz force equation, we can conclude that:
- The magnetic force F is always perpendicular to both the velocity vector v and the magnetic field vector B.
- Therefore, the pair of vectors that are always perpendicular to each other are F (the magnetic force) and v (the velocity of the charged particle), as well as F and B (the magnetic field).
Consider a straight conductor of length L carrying a current I placed in a uniform magnetic field B. The force acting on the conductor can be derived as follows:
I=n⋅A⋅e⋅vd
where e is the charge of an electron.
F=q(vd×B)
For an electron, q=−e:
F=−e(vd×B)
dF=n⋅A⋅e⋅vd⋅dL⋅Bsin(θ)
where θ is the angle between vd and B.
F=I⋅L⋅Bsin(θ)
If L is in the direction of the current and B is perpendicular to L, then:
F=I⋅L⋅B
Thus, the force acting on a current-carrying conductor of length L in a uniform magnetic field B is given by:
F=IL×B
Topper's Solved these Questions
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:PHYSICS (Theory) DELHI BOARD -2016 [SET -I][COMPTT.]
Explore 11 Videos
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:C.B.S.E.CLASS-XII PHYSICS (THEORY) [SET-I] (SECTION-A)
Explore 5 Videos
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:SECTION-B
Explore 52 Videos
- SAMPLE PAPER 2019Book:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:SAMPLE PAPER 2019Exercise:SECTION D
Explore 6 Videos
Similar Questions
Knowledge Check
The magnetic force →F on a current carrying conductor of length I in an external magnetic field →B is given by
AI×→B→lB→l×→BICI(→l×→B)Dl2→l×→B
When is the force experienced by a current-carrying conductor placed in a magnetic field is largest?
View SolutionCalculate the force on a current carrying wire in a uniform magnetic field as shown in Fig. 1.59.
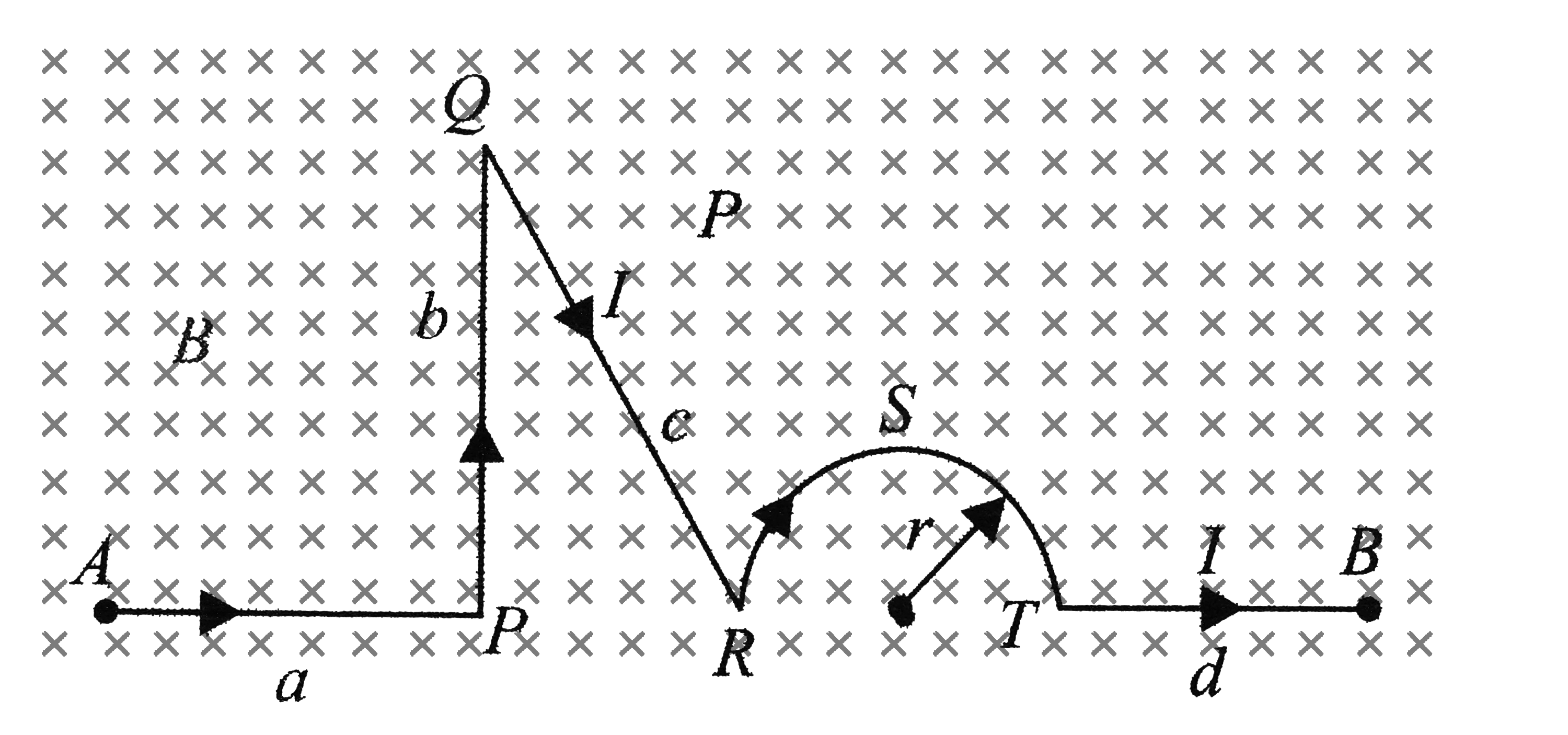
View Solutionthe force on current carrying wire placed in uniform magnetic field B as shown in figure is

View SolutionDerive an expression for the force between two long parallel current carrying conductors. Use this expression to define SI unit of current.
View SolutionCan you derive an expression for the force applied on crosswire by the field B?
View SolutionNo net force acts on a rectangular coil carrying a steady current when suspended freely in a uniform magnetic field.
View SolutionNo net force acts on a rectangular coil carrying a steady current when suspended freely in a uniform magnetic field.
View Solution