An ammeter of resistance 0⋅80Ω can measure current upto 1⋅0A.
(a) What must be the value of shunt resistance to enable the ammeter to measure current upto 5⋅0A?
(b) What is the combined resistance of the ammeter and the shunt?
To solve the problem step by step, we will break it down into two parts as given in the question.
Given:
- Resistance of the ammeter Rg=0.80Ω
- Maximum current the ammeter can measure Ig=1.0A
- Total current to be measured I=5.0A
Part (a): Finding the value of shunt resistance Rs
1. Understanding the Current Distribution:
The total current I splits between the ammeter and the shunt. The current through the ammeter Ig is 1.0 A, and the remaining current through the shunt Is is:
Is=I−Ig=5.0A−1.0A=4.0A
2. Using the Current Division Rule:
The current through the ammeter and the shunt can be expressed using the formula:
IgIs=RsRg
Rearranging gives us:
Ig⋅Rs=Is⋅Rg
3. Substituting the Known Values:
Substitute Ig=1.0A, Is=4.0A, and Rg=0.80Ω:
1.0⋅Rs=4.0⋅0.80
Rs=4.0⋅0.80=3.2Ω
4. Calculating Shunt Resistance:
Since we need to find the shunt resistance that allows the ammeter to measure up to 5.0 A, we can use the formula derived from the current division:
Rs=Rg⋅IgI−Ig
Substituting the values:
Rs=0.80⋅1.05.0−1.0=0.804.0=0.20Ω
Part (b): Finding the combined resistance Rp
1. Using the Formula for Combined Resistance:
The combined resistance Rp of the ammeter and shunt can be calculated using the formula:
Rp=Rs⋅RgRs+Rg
2. Substituting Known Values:
Substitute Rs=0.20Ω and Rg=0.80Ω:
Rp=0.20⋅0.800.20+0.80=0.161.0=0.16Ω
Final Answers:
- (a) The value of shunt resistance Rs=0.20Ω
- (b) The combined resistance Rp=0.16Ω
- Resistance of the ammeter Rg=0.80Ω
- Maximum current the ammeter can measure Ig=1.0A
- Total current to be measured I=5.0A
The total current I splits between the ammeter and the shunt. The current through the ammeter Ig is 1.0 A, and the remaining current through the shunt Is is:
Is=I−Ig=5.0A−1.0A=4.0A
The current through the ammeter and the shunt can be expressed using the formula:
IgIs=RsRg
Rearranging gives us:
Ig⋅Rs=Is⋅Rg
Substitute Ig=1.0A, Is=4.0A, and Rg=0.80Ω:
1.0⋅Rs=4.0⋅0.80
Rs=4.0⋅0.80=3.2Ω
Since we need to find the shunt resistance that allows the ammeter to measure up to 5.0 A, we can use the formula derived from the current division:
Rs=Rg⋅IgI−Ig
Substituting the values:
Rs=0.80⋅1.05.0−1.0=0.804.0=0.20Ω
The combined resistance Rp of the ammeter and shunt can be calculated using the formula:
Rp=Rs⋅RgRs+Rg
Substitute Rs=0.20Ω and Rg=0.80Ω:
Rp=0.20⋅0.800.20+0.80=0.161.0=0.16Ω
- (a) The value of shunt resistance Rs=0.20Ω
- (b) The combined resistance Rp=0.16Ω
Topper's Solved these Questions
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET - II (Delhi Board)
7 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET - III (Delhi Board)
6 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - PHYSICS (Theory) [SET - III]
9 Videos
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D
6 Videos
Similar Questions
An ammeter of resistance 1 Ohm can measure current upto 1.0 A. (i) What must be the value of the shunt resistance to enable the ammeter to measure upto 5.0 A? (ii) What is the combined resistance of the ammeter and the shunt?
View SolutionAn ammeter of resistance 0.6Ω can measure current upto 1.0 A. Calculate (i) The shunt resistance required to enable the ammeter to measure current upto 5.0 A (ii) The combined resistance of the ammeter and the shunt.
View Solution
Knowledge Check
A galvanometer of resistance 70Ω, is converted to an ammeter by a shunt resistance r3=0.03Ω. The value of its resistance will become
A0.025ΩB0.022ΩC0.035ΩD0.030Ω
A galvanometer of resistance 100Ω gives full scale deflection for 10mA current. What should be the value of shunt, so that it can measure a current of 100mA?
View SolutionA galvanometer of resistance 100Ω gives full deffection for a current 10−5 the value of shunt required to convert it into ammeter of range 1 ampere is:-
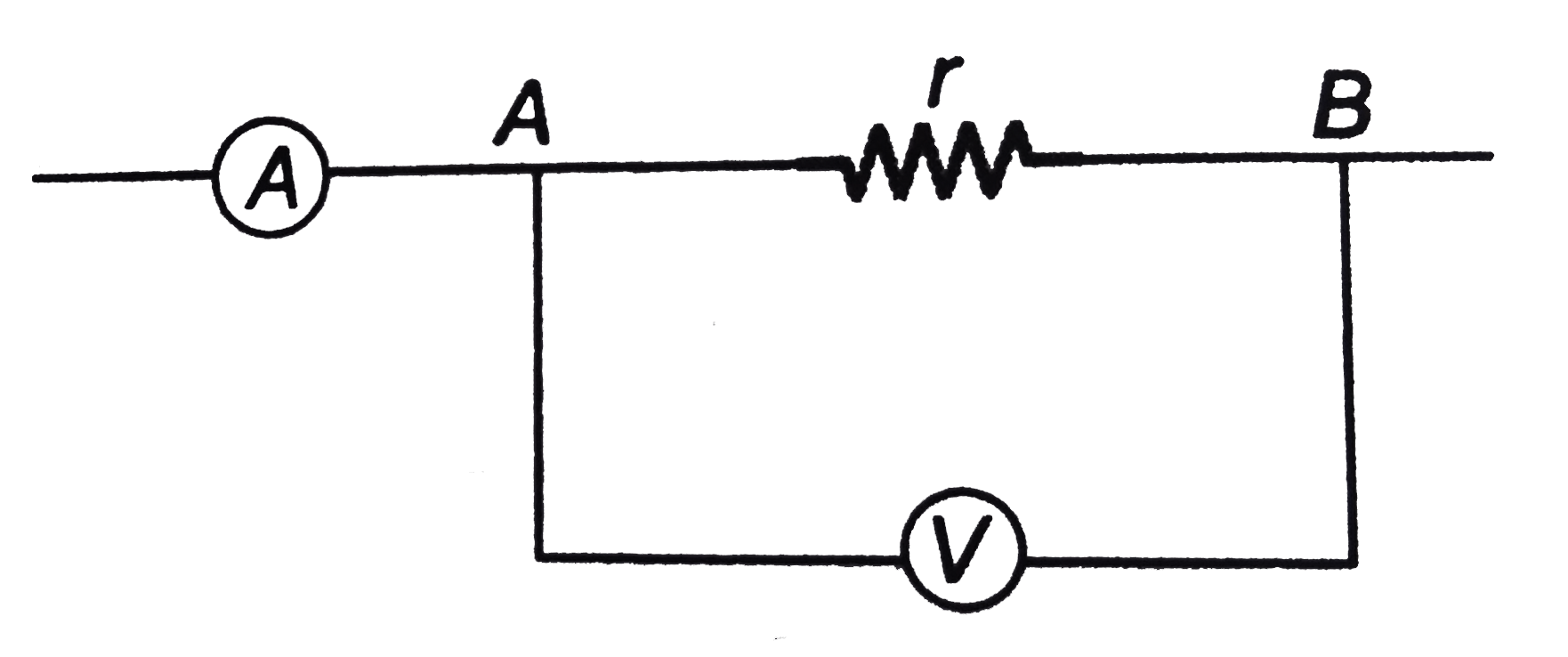
View SolutionDetermine the resistance r if an ammeter shows a current of I=5A and a voltmeter 100V. The internal resistance of the voltmeter is R=2,500Ω
View SolutionA galvanometer of resistance 22.8Ω measures 1 A. How much shunt should be used , so that it can be used to measure 20 A ?
View SolutionThe resistance of an ammeter is 13Ω and its scale is graduated for a current upto 100A. After an additional shunt has been connected to this ammeter it becomes possible to measure currents upto 750A by this meter. The value of shunt resistance is
View SolutionA DC ammeter has resistance 0.1Ω and its current ranges 0−100A. If the range is to be extended to 0−500, then the following shunt resistance will be required
View SolutionA galvanometer having a coil resistance of 60Ω shows full scale defection when a current of 1.0A passes thoguth it. It can vbe convered into an ammeter to read currents up to 5.0A by
View Solution
