A glass lens of refractive index 1.45 when immersed in a transparent liquid becomes invisible. Under what condition does it happen ?
To determine the condition under which a glass lens of refractive index 1.45 becomes invisible when immersed in a transparent liquid, we can follow these steps:
Step 1: Understand the Concept of Refraction
Refraction occurs when light passes from one medium to another and changes speed, which causes the light to bend. The refractive index (n) of a medium is a measure of how much the speed of light is reduced inside that medium compared to the speed of light in a vacuum.
Step 2: Define the Condition for Invisibility
A lens becomes invisible when there is no refraction occurring at the boundary between the lens and the surrounding medium. This happens when the refractive indices of the two materials are equal.
Step 3: Set Up the Equation
Let:
- nglass=1.45 (the refractive index of the glass lens)
- nliquid (the refractive index of the transparent liquid)
For the lens to become invisible, we need:
nglass=nliquid
Step 4: Conclusion
Thus, the condition for the glass lens to become invisible when immersed in a transparent liquid is that the refractive index of the liquid must equal the refractive index of the glass lens. Therefore:
nliquid=1.45
Final Answer
The glass lens becomes invisible in a transparent liquid when the refractive index of the liquid is equal to 1.45.
---
Refraction occurs when light passes from one medium to another and changes speed, which causes the light to bend. The refractive index (n) of a medium is a measure of how much the speed of light is reduced inside that medium compared to the speed of light in a vacuum.
A lens becomes invisible when there is no refraction occurring at the boundary between the lens and the surrounding medium. This happens when the refractive indices of the two materials are equal.
Let:
- nglass=1.45 (the refractive index of the glass lens)
- nliquid (the refractive index of the transparent liquid)
nglass=nliquid
Thus, the condition for the glass lens to become invisible when immersed in a transparent liquid is that the refractive index of the liquid must equal the refractive index of the glass lens. Therefore:
nliquid=1.45
The glass lens becomes invisible in a transparent liquid when the refractive index of the liquid is equal to 1.45.
Topper's Solved these Questions
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:[Set- II]
Explore 4 Videos
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:[Set-III]
Explore 3 Videos
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:SET-III, DELHI BOARD
Explore 4 Videos
- SAMPLE PAPER 2019Book:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:SAMPLE PAPER 2019Exercise:SECTION D
Explore 6 Videos
Similar Questions
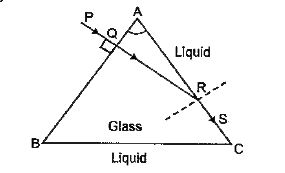
A narrow beam of monochromatic light, PQ, is incident normally on one face of an equiangular glass prism of refractive index 1.45. When the prism is immersed in a certain liquid, the ray makes a grazing emergence along the other face (figure). Find the refractive index of this liquid .
View SolutionStatement-1 : A glass convex lens (refractive index, μ1=1.5), when immersed in a liquid of refractive index μ2=2, functions as a diverging lens.
Statement-2 : The focal length of a lens is given by 1f=(μ−1)(1R1−1R2), where μ is the relative refractive index of the material of the lens with respect to the surrounding medium.
View SolutionA glass lens of refractive index 1.5 is placed in a trough of liquid. What must be the refractive index of the liquid in order to mark the lens disappear?
View SolutionA narrow beam of monochromatic light, pe, is incident normally on one face of an equiangular glass prism of refractive inder 1-45. When the prism is immersed in a certain liquid, the ray makes a grazing emergence along the other face (See below figure). Find the refractive index of this liquid.
View SolutionA double convex lens of refractive index m1 is immersed in a liquid of refractive index m2. This lens will act as:
View SolutionA converging lens has a focal length of 20cm in air. It is made of a material of refractive index 1.6. If it is immersed in a liquid of refractive index 1.3, what will be its new foacl length ?
View SolutionWhen a lens of refractive index n1, then the lens looks to be dissapeared only, if
View SolutionA converging lens of refractive index 1.5 is kept in a liquid medium having the same refractive index. What would be the focal length of the lens in the medium ?
View SolutionTwo identical equi- concave lenses made of glass of refractive index 1.5 placed in contact has power P. When a liquid of refractive index μ is filled in the gap between the concave lenses,the power becomes (P3).The value of μ is R2.Find K.
View SolutionA glass prism of refractive index 1.5 is immersed in water (refractive index 4/3) . A light beam normally on the face AB is totally reflected to reach on the face BC if

View Solution