In the given figure l||m and M is the mid-point of a line segment AB .Show that M is also the mid- point of any line segment CD, having its end points on l and m, respectively.
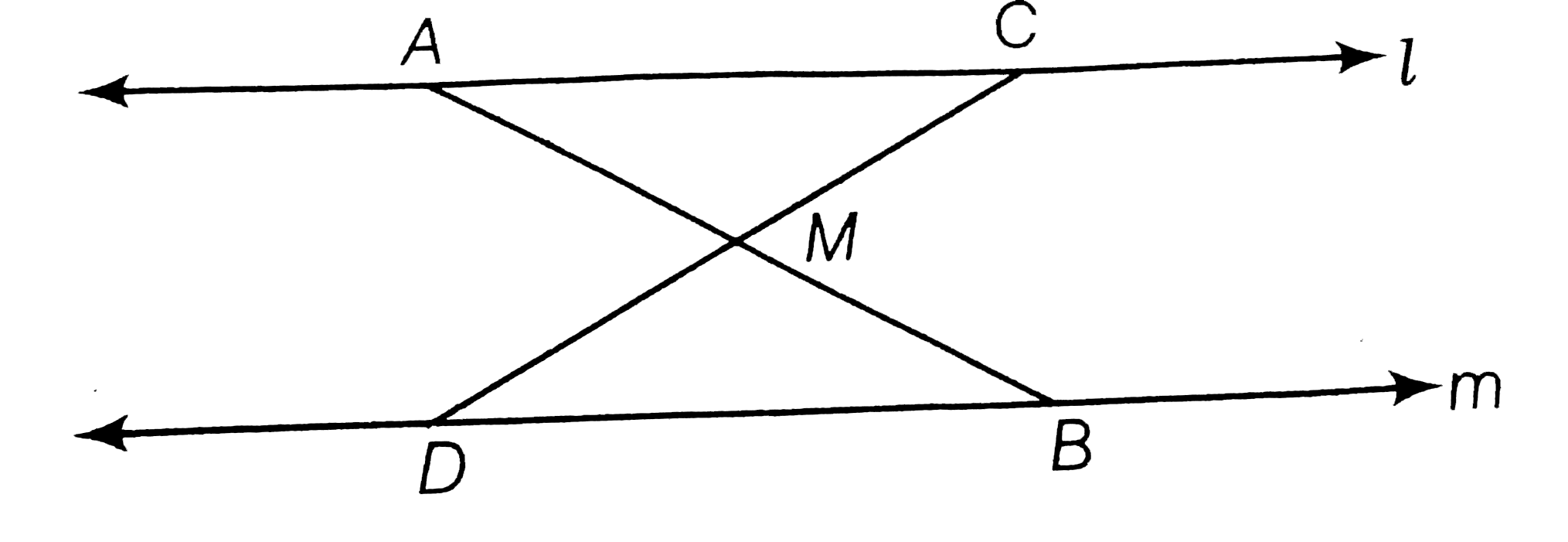
Given in the figure,l|| m and mid -Point of a line segment AB i.e AM =BM
Pr∞fbrecause l||m[given]
∠BAC=∠ABD [altermate interior angles]
∠AMC=∠BMD [vertically opposite angles]
InΔAMCandΔBMD ∠BAC=∠ABD [Proved above ]
AM=BM [given]
and ∠AMC=∠BMD [proved above ]
∵ ΔAMC≅ΔBMD [byASA≅ruencere–]
⇒ MC=MD [byCPCT]
Topper's Solved these Questions
- TRIANGLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIANGLESEXERCISE - LONG ANSWER TYPE QUESTIONS
21 Videos
- TRIANGLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIANGLESEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
12 Videos
- STATISTICS AND PROBABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - LONG ANSWER TYPE QUESTIONS
12 Videos
- VOLUME AND SURFACE AREABOOK - NCERT EXEMPLAR ENGLISHCHAPTER - VOLUME AND SURFACE AREAEXERCISE - LONG ANSWER TYPE QUESTIONS
8 Videos
Similar Questions
In Figure, lIIm and M is the mid-point of the line segment AB. Prove that M is also the mid-point of any line segment CD having its end-points on l and m respectively.
View SolutionIn Figure, l∣∣m and M is the mid-point of the line segment AB . Prove that M is also the mid-pooint of any line segment CD having its end-points on l and m respectively.
View Solution
Knowledge Check
A chord of a circle is a line segment with its end points....
Aon the circleBinterior of the circleCexterior of the circleDNone of these
The mid point of the line segment joining the points (−5,7)and(−1,3) is
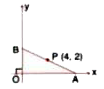
View SolutionIn the given figure, P (4, 2) is mid-point of line segment AB. Find the co-ordinates of A and B.
View SolutionShow that the mid-point of the line segment joining the points (5,7) and (3,9) is also the mid-point of the line segment joining the points (8,6) and (0,10) .
View SolutionFind the mid point of the line segment joining (3,6) and (5,-2).
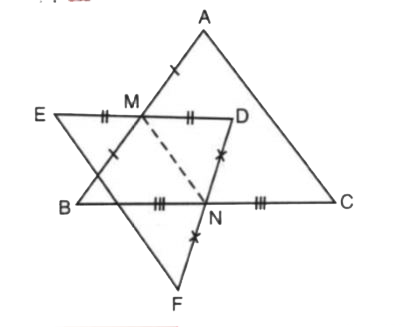
View SolutionIn the given figure, M is mid-point of AB and DE, whereas N is mid-point of BC and DF. Show that : EF = AC.
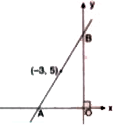
View SolutionThe mid - point of line segment AB (shown in the diagram) is (-3, 5), Find the co-ordinates of A and B.
 .
.
View SolutionThe slope of a line which passes through the origin the mid-point of the line segment joining the points (0,−4) and (8,0) is
View Solution
