ABC is an isosceles triangle with AB =AC and BD,CE are its two medians. Show that BD=CE .
To solve the problem, we will follow these steps:
Step 1: Understand the Triangle
We are given an isosceles triangle ABC where AB = AC. Points D and E are the midpoints of sides AC and AB, respectively. We need to prove that the lengths of the medians BD and CE are equal.
Step 2: Establish the Midpoints
Since D and E are midpoints:
- AE = EB (since E is the midpoint of AB)
- AD = DC (since D is the midpoint of AC)
Step 3: Express Lengths
From the properties of midpoints, we can express:
- AB = AE + EB = 2AE (because AE = EB)
- AC = AD + DC = 2AD (because AD = DC)
Step 4: Set Up the Equation
Since AB = AC, we can write:
2AE=2AD
Dividing both sides by 2 gives us:
AE=AD
This is our first equation.
Step 5: Analyze the Triangles
Now, we will consider triangles ABD and ACE:
- Angle A is common in both triangles (∠A = ∠A).
- We have established that AE = AD.
- Also, we know that AB = AC (given).
Step 6: Apply the SAS Congruence Rule
By the Side-Angle-Side (SAS) congruence criterion:
- Triangle ABD is congruent to triangle ACE.
Step 7: Conclude with CPCTC
By the Corresponding Parts of Congruent Triangles are Congruent (CPCTC) theorem:
- Therefore, BD = CE.
Final Conclusion
We have shown that the lengths of the medians BD and CE are equal, as required.
---
We are given an isosceles triangle ABC where AB = AC. Points D and E are the midpoints of sides AC and AB, respectively. We need to prove that the lengths of the medians BD and CE are equal.
Since D and E are midpoints:
- AE = EB (since E is the midpoint of AB)
- AD = DC (since D is the midpoint of AC)
From the properties of midpoints, we can express:
- AB = AE + EB = 2AE (because AE = EB)
- AC = AD + DC = 2AD (because AD = DC)
Since AB = AC, we can write:
2AE=2AD
Dividing both sides by 2 gives us:
AE=AD
This is our first equation.
Now, we will consider triangles ABD and ACE:
- Angle A is common in both triangles (∠A = ∠A).
- We have established that AE = AD.
- Also, we know that AB = AC (given).
By the Side-Angle-Side (SAS) congruence criterion:
- Triangle ABD is congruent to triangle ACE.
By the Corresponding Parts of Congruent Triangles are Congruent (CPCTC) theorem:
- Therefore, BD = CE.
We have shown that the lengths of the medians BD and CE are equal, as required.
Topper's Solved these Questions
- TRIANGLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIANGLESEXERCISE - LONG ANSWER TYPE QUESTIONS
21 Videos
- TRIANGLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIANGLESEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
12 Videos
- STATISTICS AND PROBABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - LONG ANSWER TYPE QUESTIONS
12 Videos
- VOLUME AND SURFACE AREABOOK - NCERT EXEMPLAR ENGLISHCHAPTER - VOLUME AND SURFACE AREAEXERCISE - LONG ANSWER TYPE QUESTIONS
8 Videos
Similar Questions
ABC is an isosceles triangle with AB = AC = 2a and BC = a . If AD⊥BC, find the length of AD .
View SolutionABC is an isosceles triangle with AB = AC =13 cm and BC = 10 cm. Calculate the length of the perpendicular from A to BC.
View SolutionIn an isosceles triangle ABC, AB = AC and D is a point on BC produced. Prove that :
AD2=AC2+BD.CD
View Solution△ABC is an isosceles triangle with AC = BC. If AB2=2AC2 . Prove that △ABC is a right triangle.
View SolutionABC is a triangle in which AB=ACandD is a point on AC such that BC2=AC×CD.Prove that BD=BC.
View SolutionABC is an isosceles triangle in which altitudes BE and CF are drawn to equal sides AC and AB respectively (see Fig. 7.31). Show that these altitudes are equal.
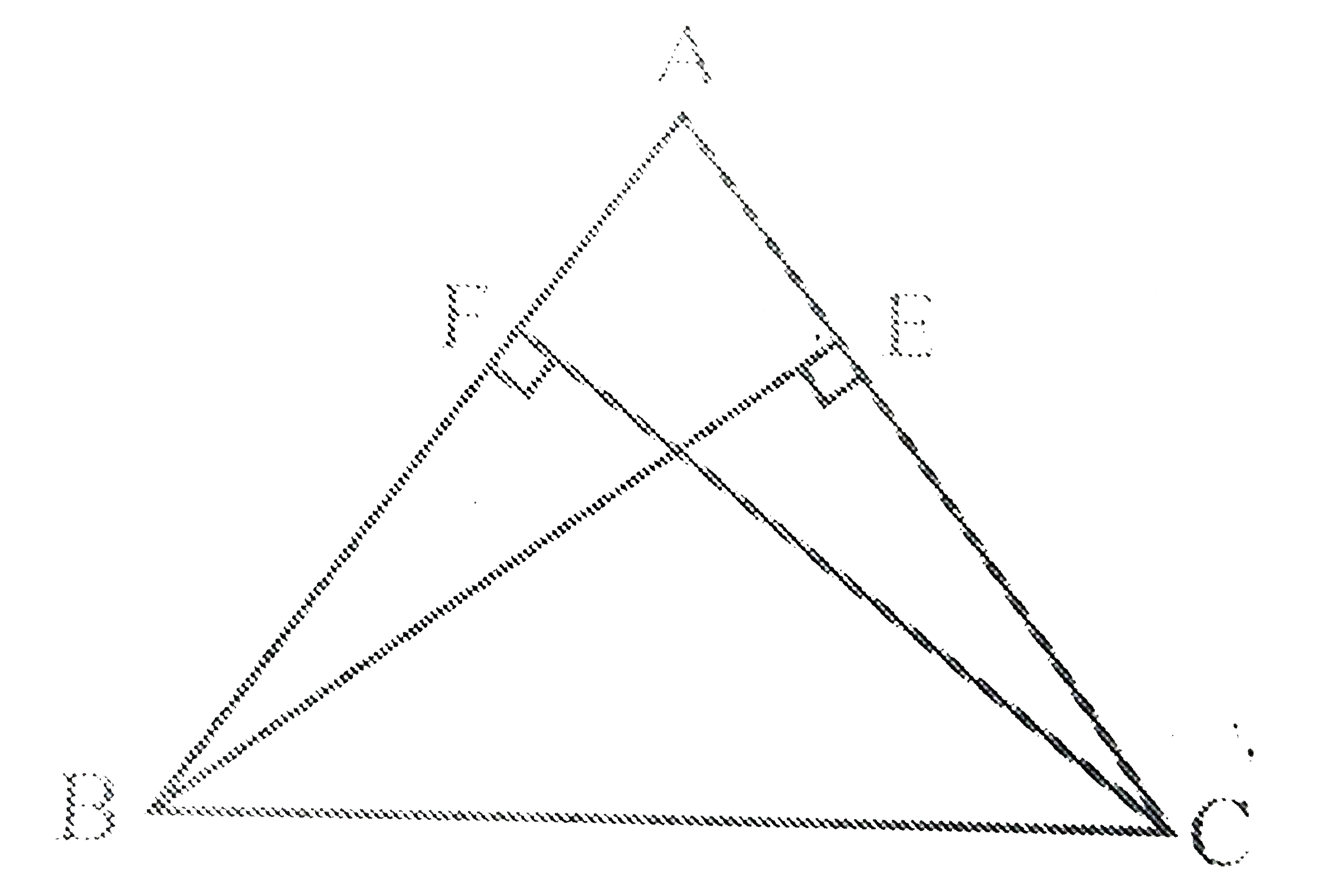
View SolutionABC is a triangle in which AB = AC and D is any point on BC. Prove that:
AB2−AD2=BD.CD
View SolutionConstruct an isosceles triangle ABC with AB = AC, base BC = 7 cm and altitude from A = 6.5 cm.
View SolutionIf D any point on the BC of an isosceles triangle ABC then prove that AB>AD and AC>AD.
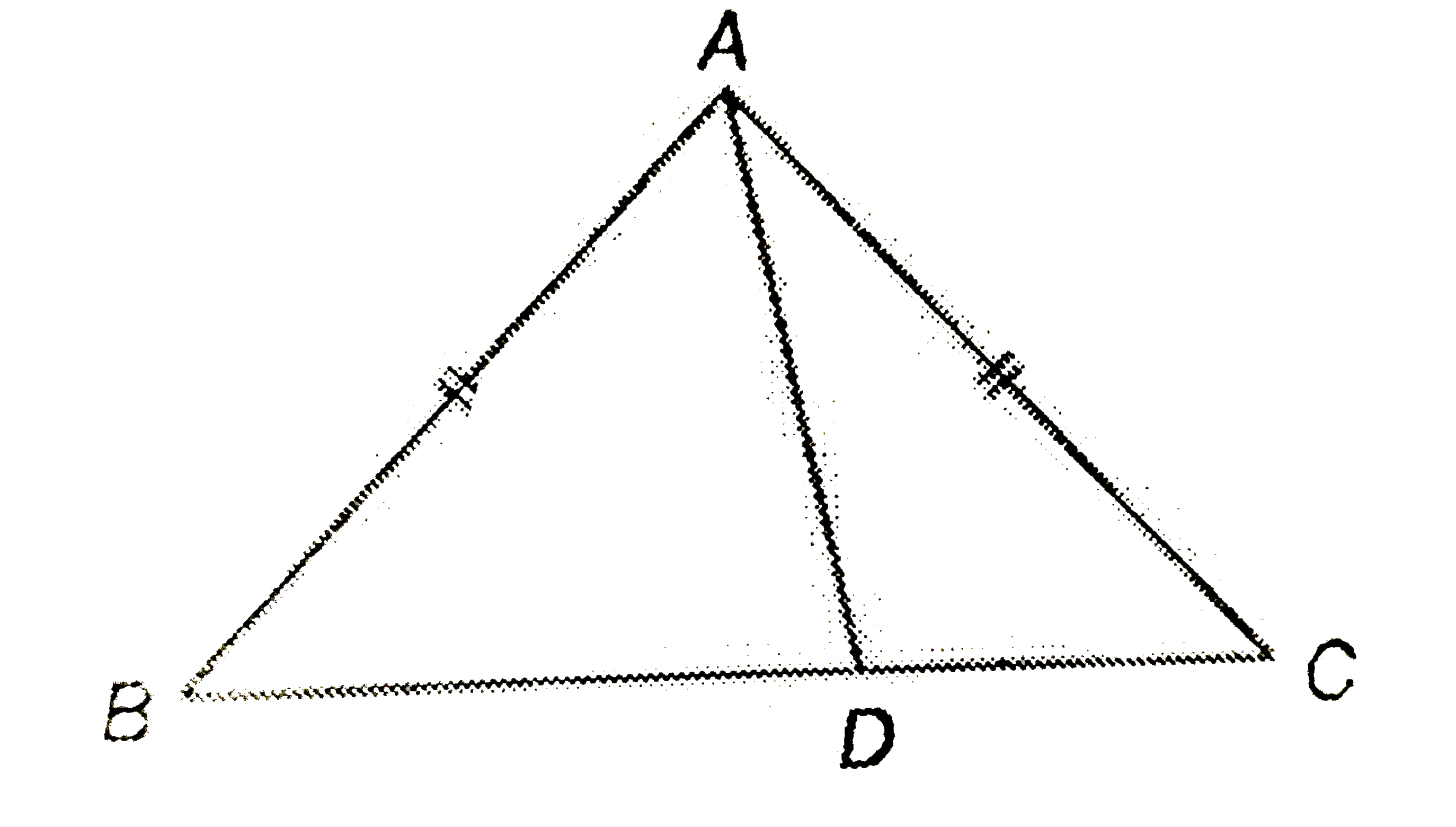
View SolutionBisectors of the angles B and C of an isosceles ΔABC with AB=AC intersect each other at O. Show that external angle adajcent to ∠ABC is equal to ∠BOC.
View Solution
