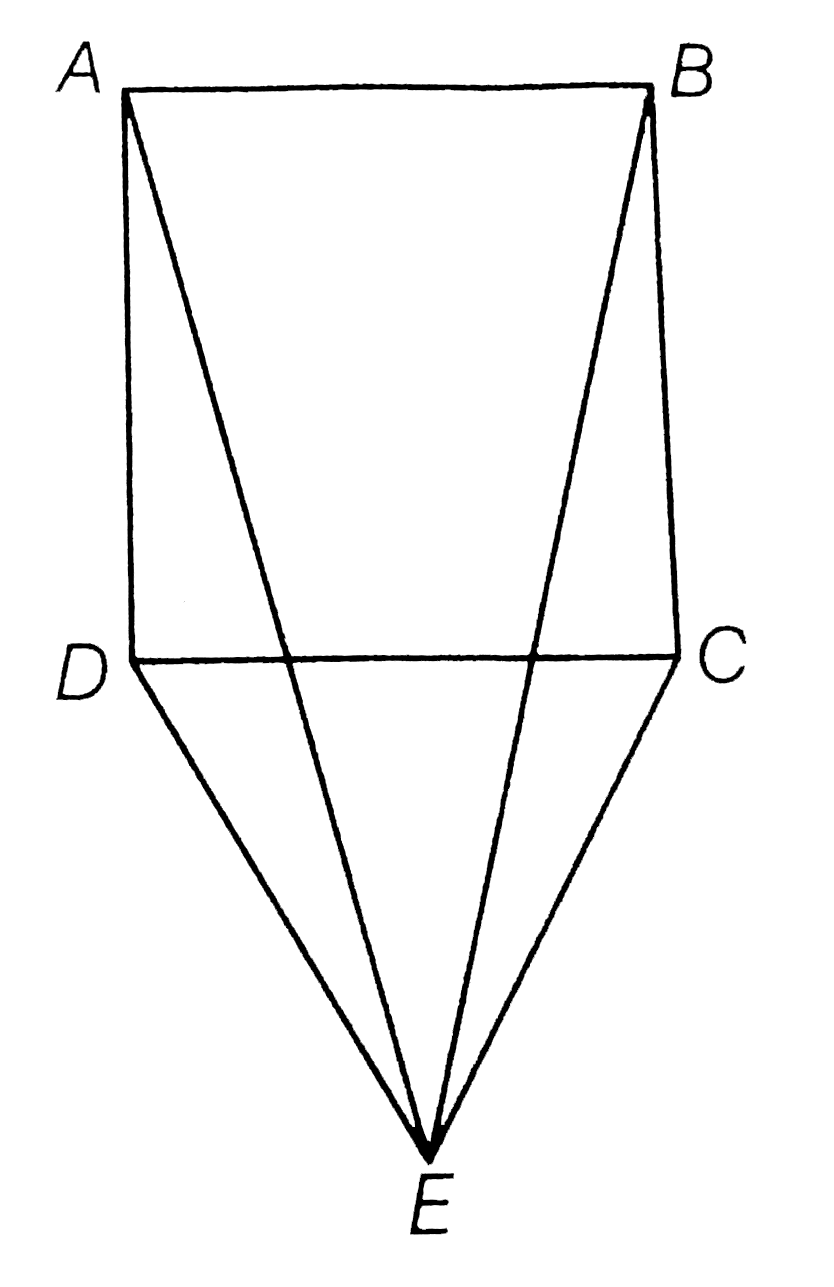
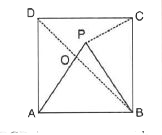
In the given figure, is an equilateral triangle triangle formed on a side of a square . Show that
Video Solution
Text Solution
Given in figure is an equilateral triangle formed on a side CD of a square ABCD.
To show
Proof in
|
Topper's Solved these Questions
- TRIANGLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIANGLESEXERCISE - LONG ANSWER TYPE QUESTIONS
21 Videos
- TRIANGLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIANGLESEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
12 Videos
- STATISTICS AND PROBABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - LONG ANSWER TYPE QUESTIONS
12 Videos
- VOLUME AND SURFACE AREABOOK - NCERT EXEMPLAR ENGLISHCHAPTER - VOLUME AND SURFACE AREAEXERCISE - LONG ANSWER TYPE QUESTIONS
8 Videos
Similar Questions
Knowledge Check
Find the area of an equilateral triangle with a side length of 12
A6√3B18√3C36√3D72√3
In the given figure, ΔABC is an equilateral triangle of side 3 units. Find the coordinates of the other two vertices.
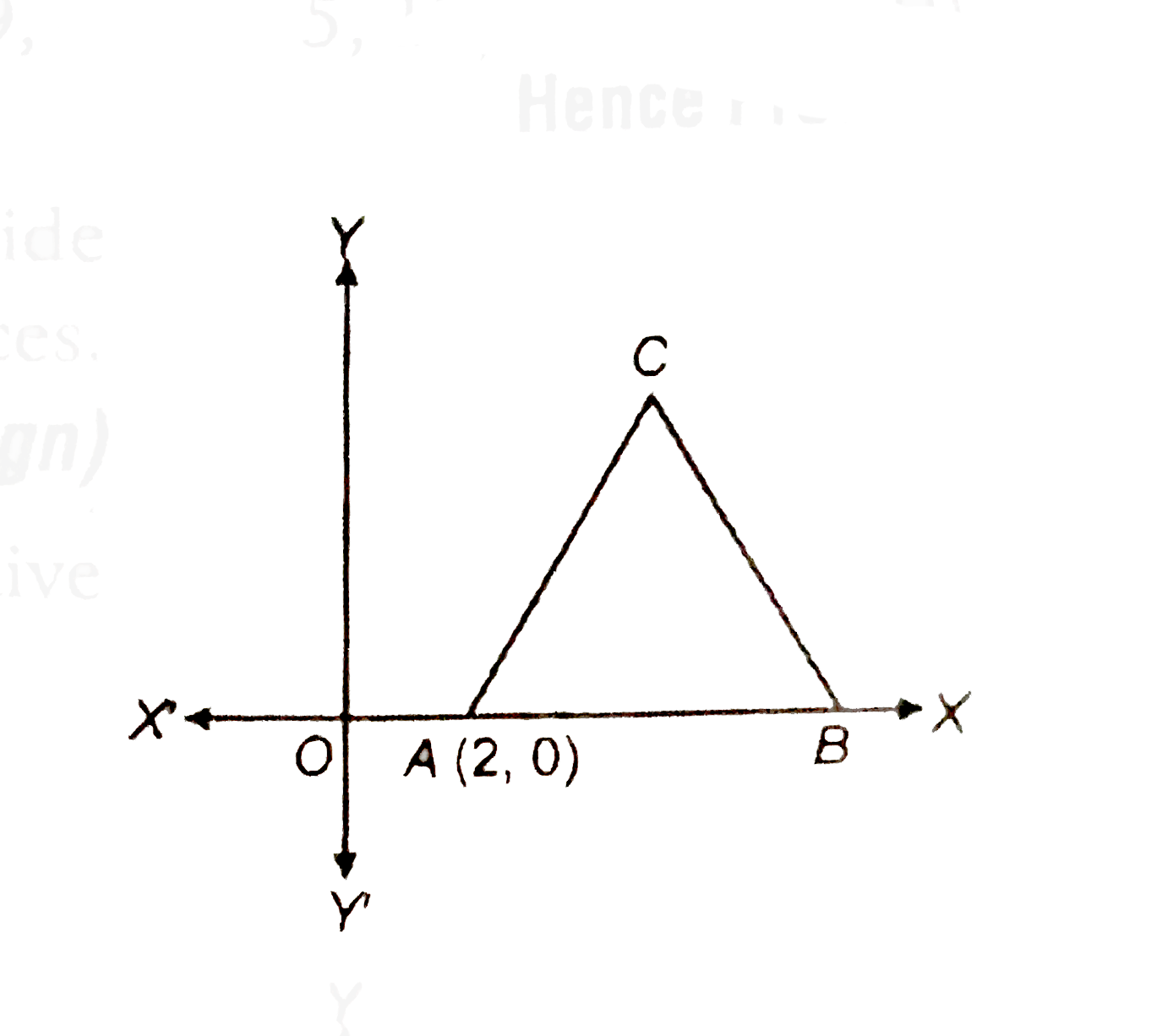
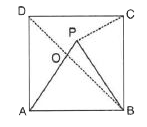
View SolutionThe given figure shows a square ABCD and an equilateral triangle ABP. Calculate:
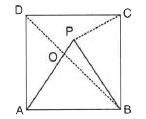
∠PCD
View SolutionThe given figure shows a square ABCD and an equilateral triangle ABP. Calculate:
∠BPC
View SolutionConstruct an equilateral triangle of sides 6 cm each.
View SolutionFind the perimeter of an equilateral triangle with side 9cm.
View SolutionFind the altitude of an equilateral triangle of side 8 cm.
View SolutionThe given figure shows a square ABCD and an equilateral triangle ABP. Calculate:
∠AOB
View Solution
