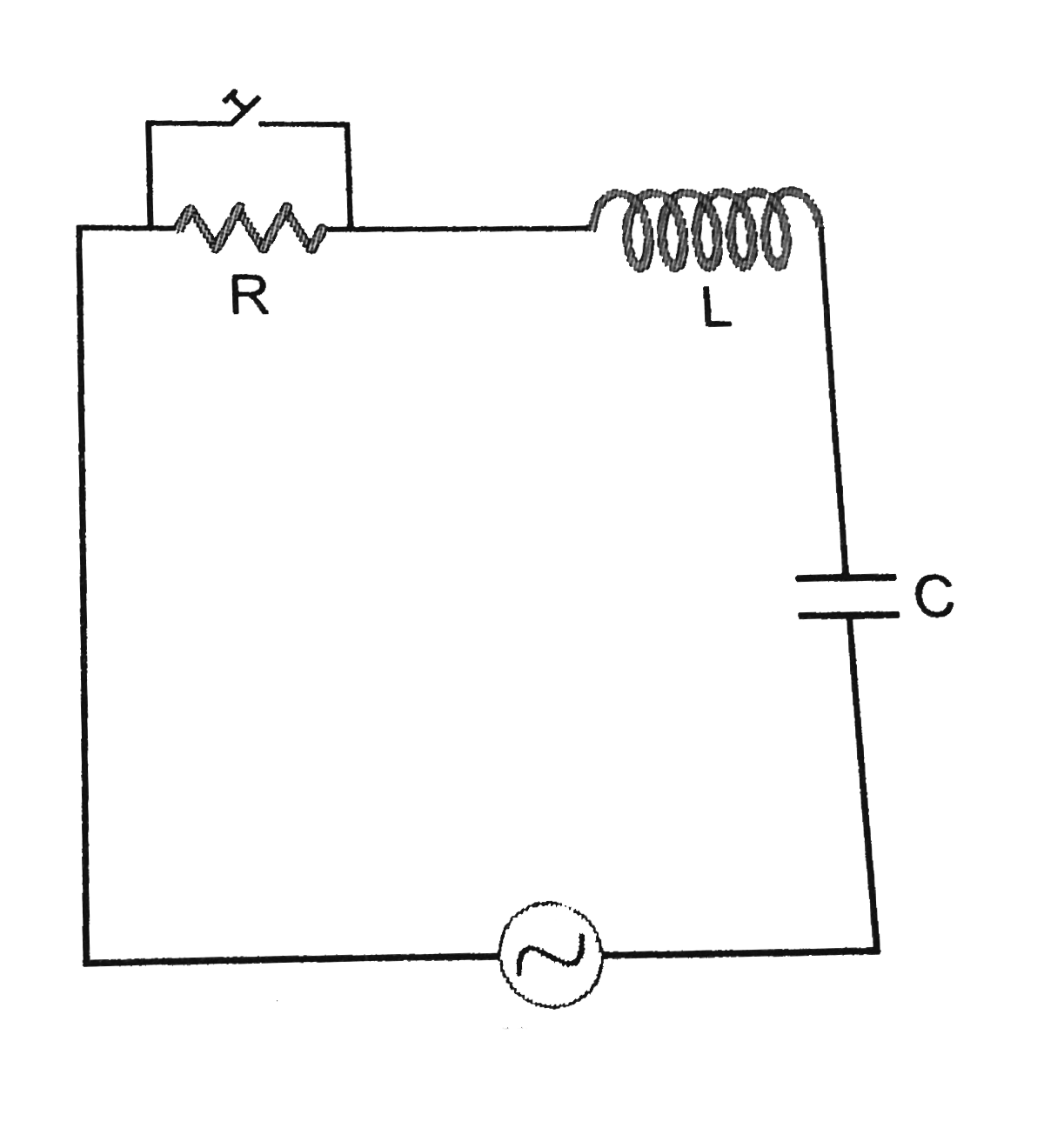
In the LCR circuit shown in Fig., the ac driving voltage is υ=υmsinωt.
(i) Write down the equation of motion for q (t).
(ii) At t=t0, the source stops and R is short circuited.
Now write down how much energy is stored in each of L and C.
(iii) Describe subsequent motion of charges.
The correct Answer is:A
Consider the R-L-C circuit shown in the adjacent diagram.
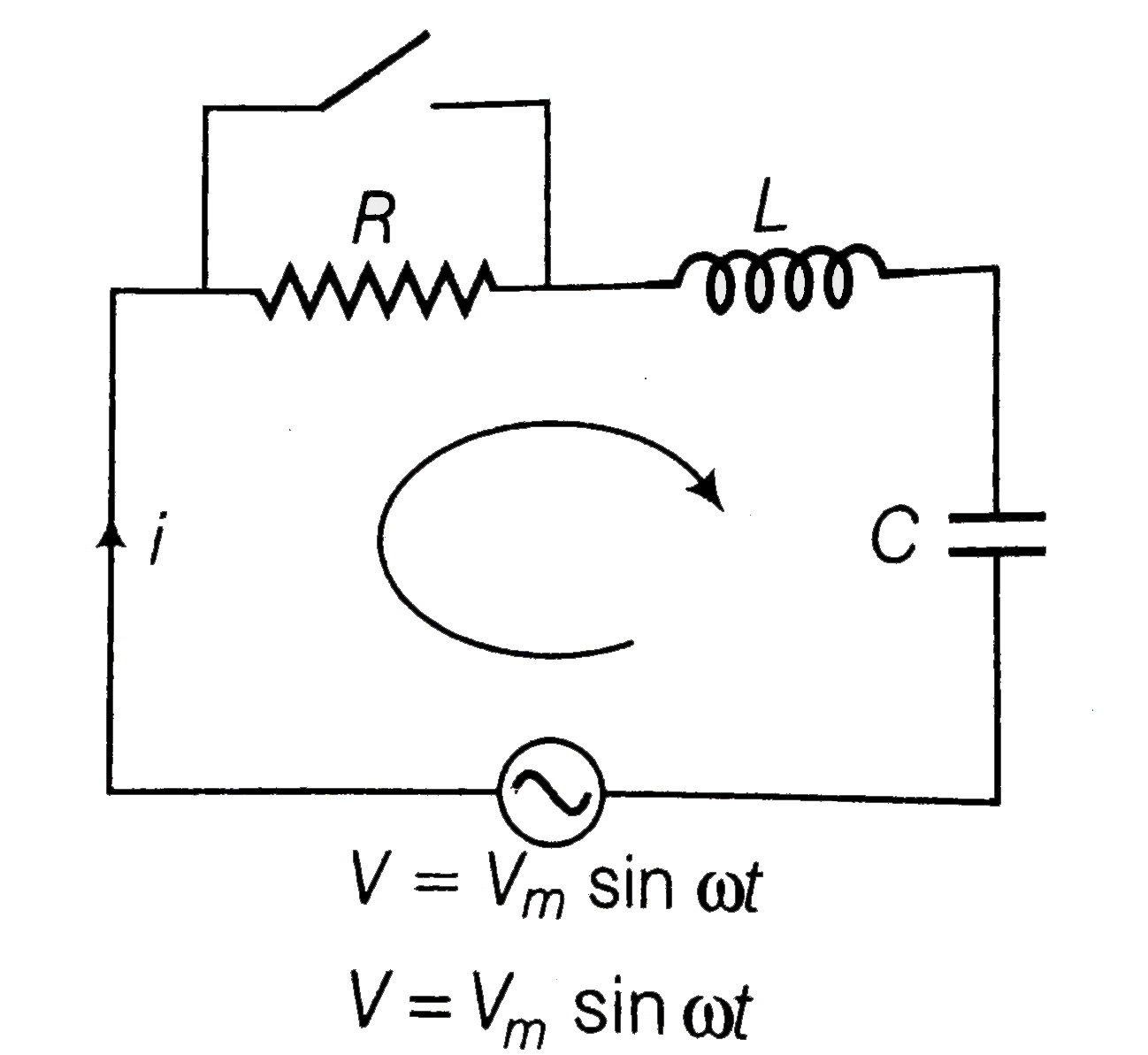
Given,
Let current at any instant be I
Applying KVL in the given circuit
iR+Ldidt+qC−Vmsinωt=0……….(i)
Now, we can write i=dqdt⇒didt=d2q(dt)2
From Eq. (i) dqdtR+Ld2q(dt)2+qC=Vmsinωt
⇒Ld2q(dt)2+Rdqdt+qC=Vmsinωt
This is the required equation of variation (motion) of charge.
b) Let q=qmsin(ωt+ϕ)=−qmcos(ωt+ϕ)
i=imsin(ωt+ϕ)=qmωsin(ωt+ϕ)
im=VmZ=Vm√R2+(XC−XL)2
ϕ=tan−1(Xc−XL)R
Where R is short circuited at t=t0, energy is stored in L and C.
UL=12Li2=12LVm√R2+(XC−XL)2sin2(ωt0+ϕ)
and UC=12×q2C=12C[q2mcos2(ωt0+ϕ)]
=12C⎡⎢
⎢
⎢⎣Vm√R2+(XC−XL)22⎤⎥
⎥
⎥⎦
=12C×(imω)2cos2(ωt0+ϕ)
=i2m2Cω2cos2(ωt0+ϕ) (∴im=qmω]
=12C⎡⎢
⎢
⎢⎣Vm√R2+(XC−XL)22⎤⎥
⎥
⎥⎦(cos2(ωt0+ϕ)
c) When R is a short circuited, the circuit becomes an L-C oscillaor. The capacitor will go on discharging and all energy will go to L and back and forth. Hence, there is oscillation of energy from electrostatic to magnetic and magnetic to electrostatic.
Topper's Solved these Questions
Similar Questions
A series R-L circuit is subjected to an alternating voltage given as (v=v0sinωt). Then the variation of peak current (i) with frequency (v) is denoted by
View SolutionFor an LCR circuit driven at frequency ω, the equation reads Ldidt+Ri+qC=υi=υmsinωt
(i) Multiply the equation by I and simplify where possible. (ii) Interpret each term physically.
(iii) Cast the equation in the form of a conservation of energy statement.
(iv) Intergrate the equation over one cycle to find that the phase difference between υ and i must be acute.
View Solution
Knowledge Check
An ac source of voltage V=Vmsinωt is connected across the resistance R as shown in figure. The phase relation between current and voltage for this circuit is
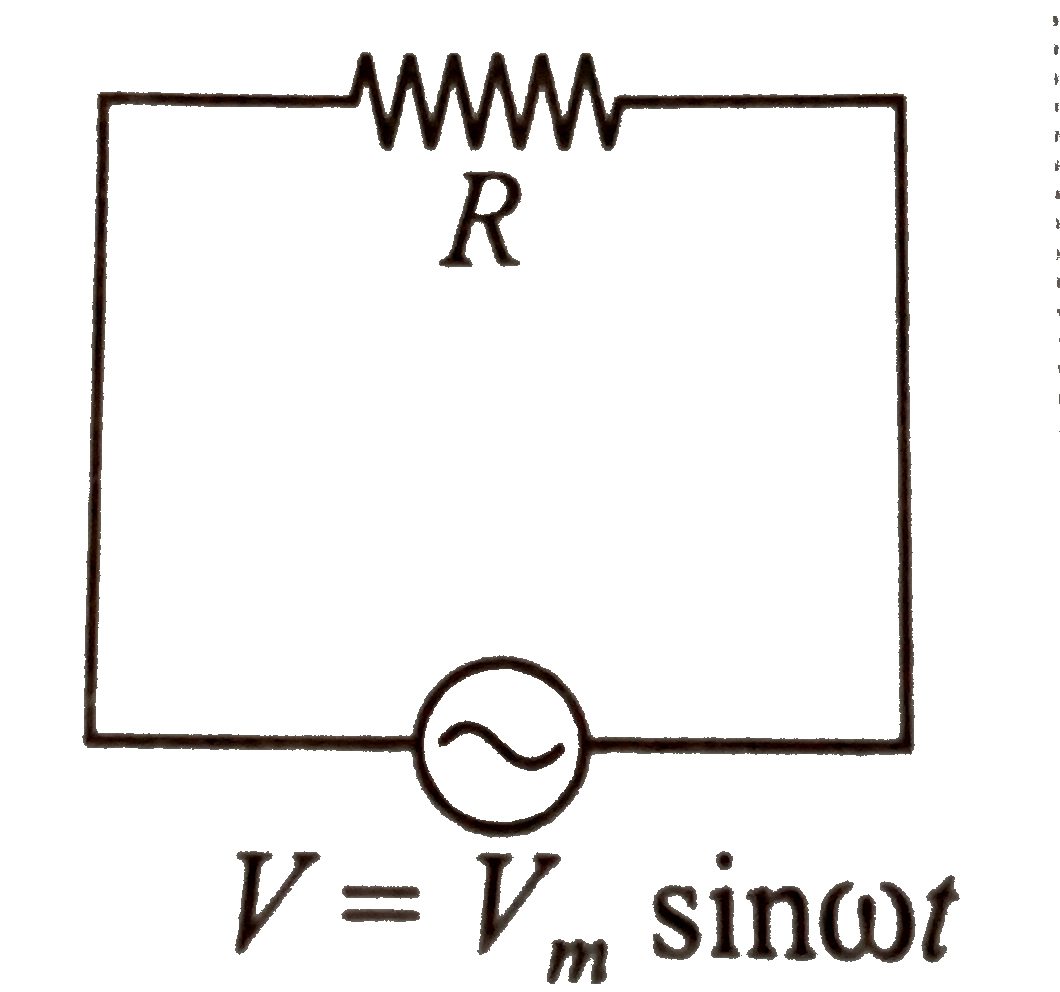 Aboth are in phaseBboth are out of phase by 90∘Cboth are out of phase by 120∘Dboth are out of phase by 180∘
Aboth are in phaseBboth are out of phase by 90∘Cboth are out of phase by 120∘Dboth are out of phase by 180∘
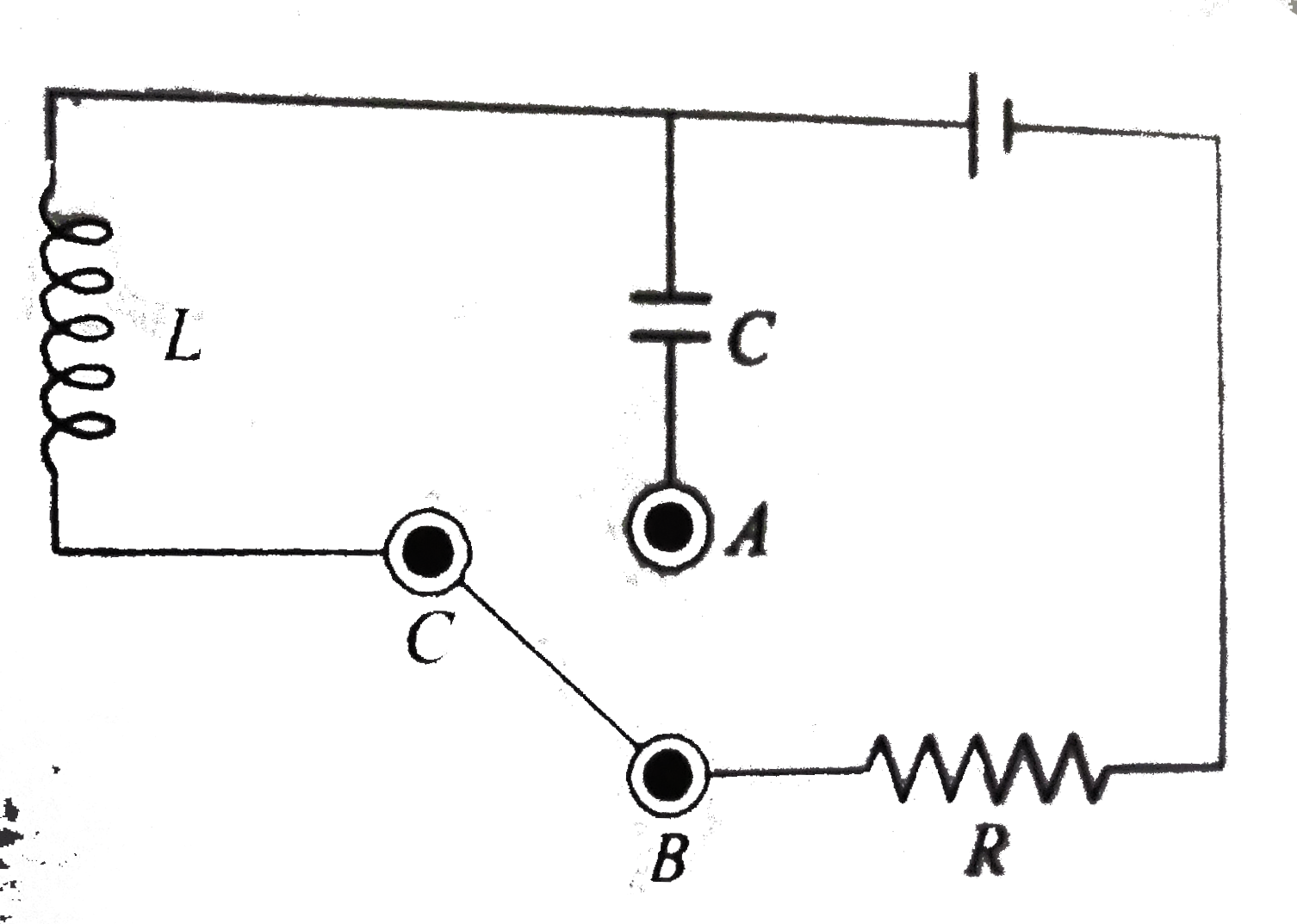
In the circuit shows in Fig the capacitor is initially uncharged and the two - way switch is connected in the position BC. Find the current through the resistence R as a function of time t. After time t=4 ms, the switch is connected in the position AC. Find the frequency of oscillation of the capacitor of the circuit in the position, and the maximum charge on the capacitor C. At what time will the energy stored in the capacitor be one-half of the total energy stored in the circuit? It is given L=2×10−4H,C=5mF,R=In210Ω and emf of the battery =1V.
View SolutionIn the circuit shown in Fig. the capacitor has capacitance C=20μF and is initially charged to 100V with the polarity shown. The resistor R0 has resistance 10Ω. At time t=0, the switch is closed. The smaller circuit is not connected in any way to teh larger one. the wire of the smaller circuit has a resistance of 1.0Ωm−1 and contains 25 loops. the larger circuit is a rectangle 2.0m by 4.0m, while the smaller one has dimensions a=10.0cm and b=20.0cm. the distance cis 5.0cm. (The figure is not drawn to scale.) Both circuit are held stationary. Assume that only the wire nearest to teh smaller circuit produces. an appreciable magnetic field through it.

The current in the circuit 200ms after closing S is
View SolutionIn the circuit shown in Fig. the capacitor has capacitance C=20μF and is initially charged to 100V with the polarity shown. The resistor R0 has resistance 10Ω. At time t=0, the switch is closed. The smaller circuit is not connected in any way to teh larger one. the wire of the smaller circuit has a resistance of 1.0Ωm−1 and contains 25 loops. the larger circuit is a rectangle 2.0m by 4.0m, while the smaller one has dimensions a=10.0cm and b=20.0cm. the distance cis 5.0cm. (The figure is not drawn to scale.) Both circuit are held stationary. Assume that only the wire nearest to teh smaller circuit produces. an appreciable magnetic field through it.

The curent in the smaller circuit 200μs after closing S is
View SolutionIn the circuit shown in Fig. the capacitor has capacitance C=20μF and is initially charged to 100V with the polarity shown. The resistor R0 has resistance 10Ω. At time t=0, the switch is closed. The smaller circuit is not connected in any way to teh larger one. the wire of the smaller circuit has a resistance of 1.0Ωm−1 and contains 25 loops. the larger circuit is a rectangle 2.0m by 4.0m, while the smaller one has dimensions a=10.0cm and b=20.0cm. the distance cis 5.0cm. (The figure is not drawn to scale.) Both circuit are held stationary. Assume that only the wire nearest to teh smaller circuit produces. an appreciable magnetic field through it.

The direction of current in the smaller circuit is
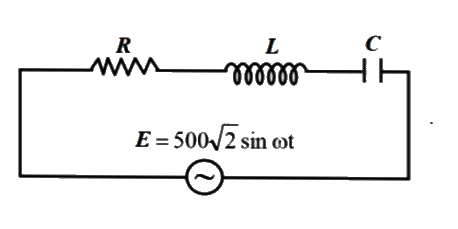
View SolutionIn the series L−C−R circuit shown in the figure, the rms voltage across the resistor and inductor are 400 V and 700 V respectively. If the applied voltage is E=500√2sin(ωt), then the peak voltage across the capacitor is
View SolutionIn a series LCR circuit connected to an ac source of variable frequency and voltage v=vmsinωt, draw a plot showing the variation of current (I) with angular frequency (ω) for two different values of resistance R1andR2(R1>R2). Write the condition under which the phenomenon of resonance occurs. For which value of the resistance out of the two curves, a sharper resonance is produced ? Define Q-factor of the circuit and give its significance.
View SolutionA charge Q is given to the plates of an isolated capacitor of capacitance C. Now, the terminals of this capacitor are connected to the terminals of an inductor L at t = 0. After how much time will the energy stored in the capacitor and the Inductor become equal?
View Solution
