If the rms current in a 50 Hz ac circuit is 5 A, the value of the current 1/300 second after its value becomes zero is
5√2A
5√3/2 A
5/6 A
5/√2 A
The correct Answer is:B
To solve the problem step by step, we will follow the outlined approach based on the information provided in the video transcript.
Step 1: Understand the relationship between RMS current and peak current
The RMS (Root Mean Square) current Irms is related to the peak current I0 by the formula:
Irms=I0√2
From this, we can express the peak current as:
I0=Irms×√2
Step 2: Substitute the given RMS current
Given that Irms=5A, we can calculate I0:
I0=5×√2
Step 3: Write the equation for instantaneous current
The instantaneous current I(t) in an AC circuit can be expressed as:
I(t)=I0sin(ωt)
where ω=2πf and f is the frequency.
Step 4: Calculate the angular frequency
Given that the frequency f=50Hz, we can calculate ω:
ω=2π×50=100πrad/s
Step 5: Calculate the time t
We need to find the current 1/300 seconds after it becomes zero. The time t is:
t=1300s
Step 6: Substitute values into the instantaneous current equation
Now we substitute I0 and ωt into the equation for I(t):
I(1300)=5√2sin(100π×1300)
Step 7: Simplify the argument of the sine function
Calculating the argument:
100π×1300=100π300=π3
Thus, the equation becomes:
I(1300)=5√2sin(π3)
Step 8: Calculate sin(π3)
We know that:
sin(π3)=√32
Substituting this back into the equation gives:
I(1300)=5√2×√32
Step 9: Simplify the expression
This simplifies to:
I(1300)=5√62A
Final Answer
The value of the current 1/300 seconds after it becomes zero is:
I(1300)=5√62A
The RMS (Root Mean Square) current Irms is related to the peak current I0 by the formula:
Irms=I0√2
From this, we can express the peak current as:
I0=Irms×√2
Given that Irms=5A, we can calculate I0:
I0=5×√2
The instantaneous current I(t) in an AC circuit can be expressed as:
I(t)=I0sin(ωt)
where ω=2πf and f is the frequency.
Given that the frequency f=50Hz, we can calculate ω:
ω=2π×50=100πrad/s
We need to find the current 1/300 seconds after it becomes zero. The time t is:
t=1300s
Now we substitute I0 and ωt into the equation for I(t):
I(1300)=5√2sin(100π×1300)
Calculating the argument:
100π×1300=100π300=π3
Thus, the equation becomes:
I(1300)=5√2sin(π3)
We know that:
sin(π3)=√32
Substituting this back into the equation gives:
I(1300)=5√2×√32
This simplifies to:
I(1300)=5√62A
The value of the current 1/300 seconds after it becomes zero is:
I(1300)=5√62A
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Very short Answer types questions
12 Videos
- ALTERNATING CURRENTBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Short Answer types questions
1 Videos
- ATOMSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - ATOMSEXERCISE - Long Answer Type
6 Videos
Similar Questions
Knowledge Check
The rms value of current in an ac circuit is 25 A, then peak current is
A35.36mAB35.36AC3.536AD49.38A
The r.m.s current in an AC circuit is 2A. If the wattless current be √3A, what is the power factor?
View SolutionIf the peak value of a current in 50Hz AC. Cirucit is 7.07 A. What is the mean value of current over half a cycle and the value of current 1/300s after it was zero?
View SolutionIn an AC circuit, the rms value of the current Irms is related to the peak current I0 as
View SolutionThe rms value of an alternating current
View SolutionThe value of current I in the circuit will be
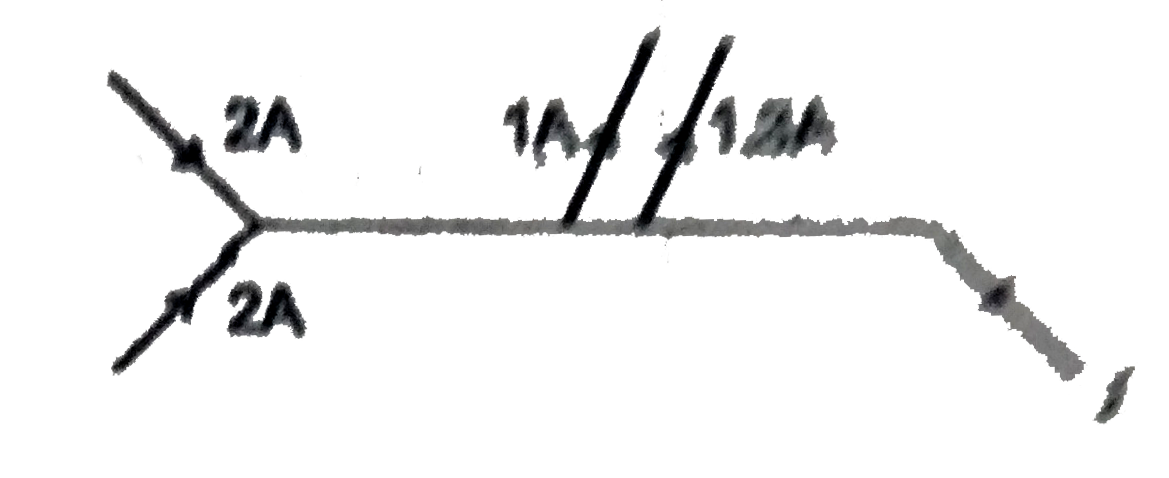
View SolutionThe peak value of an alternating current is 10. A. Find its rms value
View SolutionA resistor and an inductor are connected to an ac supply of 120 V and 50 Hz, the current in the circuit is 3 A. If the power consumed in the circuit is 108 W, then the resistance in the circuit is
View Solution