Obtain the formula for the electric field due to a long thin wire of uniform linear charge density E without using Gauss's law. (Hint: Use Coulomb's law directly and evaluate the necessary integral.)
Solution in Gujrati
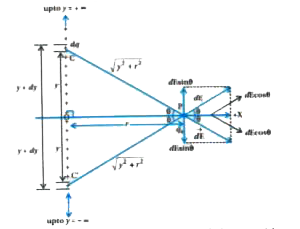
Consider infinitely extended line of charge with constant linear charge density . Let us determine its electric field at perpendicular distance r say at point P as shown in the figure. For this consider a charge element dq at distance y from central point O. Here,
Magnitude of Coulombian force exerted by above charge element dq on test charge placed at point P is,
........(1)
Magnitude of electric field of charge element dq at point P is,
.........(2)
Similary magnitude of electric field of charge element dq (selected on opposite side of central point O) at point P is also,
When we resolve above two electric fields into two mutually perpendicular components at point p we find that dEsinG components cancel each other (because of equal magnitudes and opposite directions). Hence, we get electric field at point P only due to components which are in same directions and so we can make their scalar addition to find resultant electric field E at point P Thus,
From eqn. (2) and from
...........(3)
In right angled
.......(4)
For a given point P value of r is constant and so from above equation,
........(5)
Now, (From equation (4))
.........(6)
From equation (4)
When
............(7)
From eqn (3), (5),(6) and (7),
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS Book:KUMAR PRAKASHANChapter:ELECTRIC CHARGES AND FIELDS Exercise:SECTION B NUMERICALS (NUMERICAL FOR .DARPAN. BASED ON TEXTBOOK)
Explore 8 Videos
- ELECTRIC CHARGES AND FIELDS Book:KUMAR PRAKASHANChapter:ELECTRIC CHARGES AND FIELDS Exercise:SECTION C NCERT EXEMPLAR SOLUTION (MULTIPLE CHOICE QUESTIONS (MCQs))
Explore 7 Videos
- ELECTRIC CHARGES AND FIELDS Book:KUMAR PRAKASHANChapter:ELECTRIC CHARGES AND FIELDS Exercise:SECTION B NUMERICALS (NUMERICAL FROM TEXTUAL EXERCISES)
Explore 24 Videos
- DUAL NATURE OF RADIATION AND MATTERBook:KUMAR PRAKASHANChapter:DUAL NATURE OF RADIATION AND MATTERExercise:Section-D (MCQs asked in GUJCET/Board Exam)
Explore 1 Video
- ELECTROMAGNETIC INDUCTIONBook:KUMAR PRAKASHANChapter:ELECTROMAGNETIC INDUCTIONExercise:Section D MCQs (MCQs asked in Competitive Exams )
Explore 38 Videos
Similar Questions
Two spheres of copper, having mass 1 g each, are kept 1 m apart. The number of electrons in them are 1 % less than the number of protons. The electrical force between them is Atomic wt. of copper is 63.54 g/mol, atomic number is 29, Avogadro's number NA=6.023×1023mol−1 and k=9×109 SI
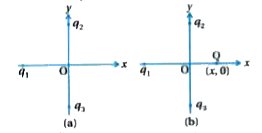
View SolutionIn figure, two positive charges q2 and q3 fixed along the y-axis, exert a net electric force in the + x direction on a charge q1 fixed along the x-axis. If a positive charge Q is added at (x, 0), the force on q1
View SolutionWhat is the force between two small charged spheres having charges of 2×10−7C and 3×10−7 C placed 30 cm apart in air ?
View SolutionMass of photon in motion is ………
View SolutionThree pure inductance each of 3 H are connected as shown in figure. The equivalent inductance of this connection between points A and B is ......

View SolutionObtain the formula for the electric field due to a long thin wire of uniform linear charge density λ without using Gauss's law. [Hint. use Coulomb's law directly and evaluate the necessary integral].
View Solutionगाउसीन नियम का उपयोग किये बिना एकसमान रैखिक आवेश घनत्व λ के लंबे एटले तार के कारण विधुत क्षेत्र के लिए सूत्र प्राप्त कीजिये [ साकेत : सीधे ही कुलाम नियमका उपयोग करके आवश्यक समाकलन का मान निकालिये ]
View SolutionState Gauss's law in electrostatics. Use this law to derive an expression for the electric field due to an infinitely long straight wire of linear charge density λcm−1
View Solutionगोस नियम का उपयोग किये बिना किसी एक्शमन रेखिक आवेश घनत्व λ के लम्बे पतले तार के कारण विधुत छेत्र के लिए सूत्र प्राप्त कीजिये संकेत शिंदे ही कलम नियम का उपयोग करके आवशयक समाकलन का मान निकालिये
View Solutionगॉस के नियम द्वारा रेखीय आवेश के कारण विद्युत् क्षेत्र की तीव्रता ज्ञात कीजिए ।
View SolutionObtain the formula for the electric field due to a long thin wire of uniform linear charge density λ without using Gauss's law.
View Solutionगाउस नियम का उपयोग किए बिना किइस एकसमान रैखिक आवेश घनत्व λ के लम्बे पतले तार के कारण विधुत क्षेत्र के लिए सूत्र प्राप्त कीजिए। [संकेत - सीधे ही कूलॉम नियम का उपयोग करके आवश्यक समाकलन का मान निकालिए ]।
View SolutionObtain the formula for the electric field due to a long thin wire of uniform linear charge density E without using gauss law
View SolutionObtain the formula for the electric field due to a long thin wire of uniform linear charge density E without using gauss law
View SolutionObtain the formula for the electric field due to a long thin wire of uniform linear charge density λ without using Gauss's law. [Hint.use Coulomb's law directly and evaluate the necessary integral ].
View Solution