A hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the hold is σ2πε0ˆn where ˆn is the unit vector in the outward normal direction, and a is the surface charge density near the hole.
 Solution in Gujrati
Solution in Gujrati
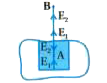
As shown in figure, assume that the hole on the conducting surface is filled by conductor. According to Gauss.s law, at A on surface,
∮→E.d→S=qε0
EdScos0∘=σdSε0[∴σ=qds]
∴E.dS=σdSε0
∴→E=σε0.ˆn
Which is electric field outside the conductor. Above electric field can be written as,
→E=→E1+→E2..........(2)
where →E1 = electric field at point P0 just outside the conductor (adjacent to point P on the surface) due to charge at point P and →E2= electric field at point P (or at P0) flue to remaining charges on the given charged hollow conductor.
When hollow charged conductor does not have any hole at point P on the surface we have →E1 and E2 pointing along ˆn and so from equation (2), we can write,
E=E1+E2=σε0...........(3)
Now consider point Pp , adjacent to point P and just inside the conductor in figure (a), where resultant electric field →E is →0. Hence,
→E=→E1+→E2=→0
∴→E1=−→E2
∴→E1=E2 (Taking magnitude).......(4)
From eqn. (3) and (4)
2E2=σε0
∴E2=σ2ε0..........(5)
Now, when we have a hole at point P electric field at point P,- in the hole due to remaining charges will be,
E2=σ2ε0ˆn.............(6)
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS Book:KUMAR PRAKASHANChapter:ELECTRIC CHARGES AND FIELDS Exercise:SECTION B NUMERICALS (NUMERICAL FOR .DARPAN. BASED ON TEXTBOOK)
Explore 8 Videos
- ELECTRIC CHARGES AND FIELDS Book:KUMAR PRAKASHANChapter:ELECTRIC CHARGES AND FIELDS Exercise:SECTION C NCERT EXEMPLAR SOLUTION (MULTIPLE CHOICE QUESTIONS (MCQs))
Explore 7 Videos
- ELECTRIC CHARGES AND FIELDS Book:KUMAR PRAKASHANChapter:ELECTRIC CHARGES AND FIELDS Exercise:SECTION B NUMERICALS (NUMERICAL FROM TEXTUAL EXERCISES)
Explore 24 Videos
- DUAL NATURE OF RADIATION AND MATTERBook:KUMAR PRAKASHANChapter:DUAL NATURE OF RADIATION AND MATTERExercise:Section-D (MCQs asked in GUJCET/Board Exam)
Explore 1 Video
- ELECTROMAGNETIC INDUCTIONBook:KUMAR PRAKASHANChapter:ELECTROMAGNETIC INDUCTIONExercise:Section D MCQs (MCQs asked in Competitive Exams )
Explore 38 Videos
Similar Questions
Two spheres of copper, having mass 1 g each, are kept 1 m apart. The number of electrons in them are 1 % less than the number of protons. The electrical force between them is Atomic wt. of copper is 63.54 g/mol, atomic number is 29, Avogadro's number NA=6.023×1023mol−1 and k=9×109 SI
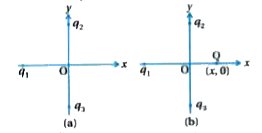
View SolutionIn figure, two positive charges q2 and q3 fixed along the y-axis, exert a net electric force in the + x direction on a charge q1 fixed along the x-axis. If a positive charge Q is added at (x, 0), the force on q1
View SolutionWhat is the force between two small charged spheres having charges of 2×10−7C and 3×10−7 C placed 30 cm apart in air ?
View SolutionMass of photon in motion is ………
View SolutionThree pure inductance each of 3 H are connected as shown in figure. The equivalent inductance of this connection between points A and B is ......

View SolutionA hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the holes is (σ/2∈0() ˆn, where ˆn is the unit vector in the outward normal direction, and σ is the surface charge density near ther hole.
View SolutionShow that the electric field at the surface of a charged conductor is given by vecE = (sigma)/(epsi_(0)) hatn , where sigma is the surface charge density and hatn is a unit vector normal to the surface in the outward direction .
View Solutionएक खोखले आवेशित गोले की सतह पर एक छोटा छिद्र काटा गया है। सिद्ध करो की छिद्र में वैधुत क्षेत्र (σ2ε0ˆn है, जहाँ ˆn अभिलम्बवत दिशा में बहिमुर्खी एकांक सदिश है तथा σ छिद्र के समीप आवेश पृष्ठ घनत्व है।
View SolutionA hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the hole is (sigma//epsi epsi_(0))hat(n) , where hat(n) is the unit vector in the outward normal direction and sigma is the surface charge density near the hole.
View SolutionA hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the hole is σ∈0ˆn, where ˆn is the unit vector in the outward direction, and σ is the surface density of charge near the hole .
View SolutionA hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the holes is (σ/2∈0 ˆn, where ˆn is the unit vector in the outward normal direction, and σ is the surface charge density near ther hole.
View SolutionA hollow charged conductor has a tiny hole cut in to its surface show that the electric field in the hole is n where n is the charge density near the hole
View SolutionA hollow charged conductor has a tiny hole cut in to its surface show that the electric field in the hole is n where n is the charge density near the hole
View SolutionA hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the holes is (σ/2ε0) ˆn, where ˆn is the unit vector in the outward normal direction, and σ is the surface charge density near the hole.
View SolutionA hollow charged conductor has a tiny hole cut into its surface. Show that the electric field in the hole is (σ2∈0)ˆn , where ˆn is the unit vector in the outward normal direction, and σ is the surface charge density near the hole,
View Solution