Two cells of emfs ε1andε2 and internal resistances r1andr2 respectively are connected in parallel . Obtain expressions for the equivalent (i) resistance and , (ii) emf of the combination.
According to Kirchhoff first law ,
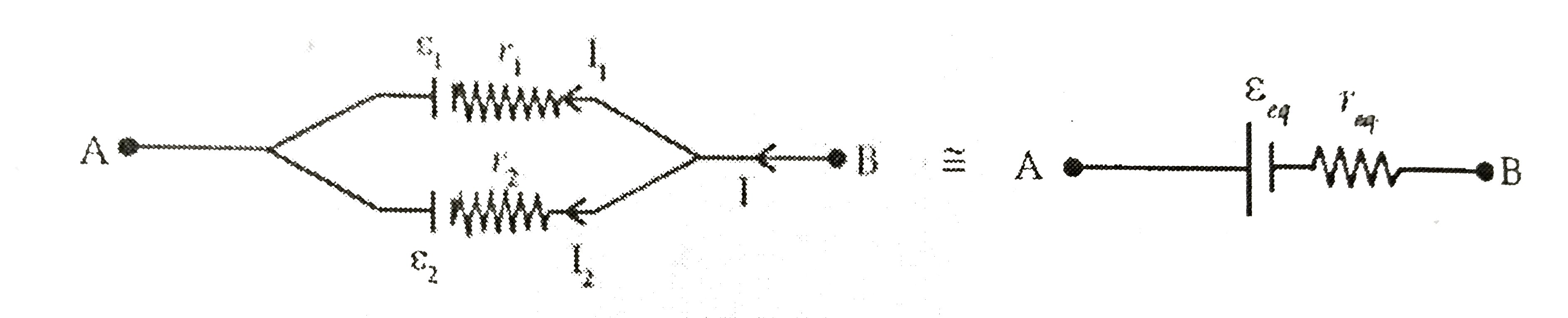
I=I1+I2 . . . (i)
Potential across upper branch V=ε1−I1r1 . . . (ii)
Potential across lower branch V=ε2−I2r2 . . . (iii)
Potential across equivalent branch V=εeq−Ireq . . . (iv)
Substituting the values of I , I1andI2 from equations (ii) , (iii) and (iv) in equation (i),
εeqreq−Vreq=ε1r1−Vr1+ε2r2−Vr2 εeqreq−Vreq=ε1r1+ε2r2+(1req+1req)V
Comparing both the sides , εeqreq=ε1r1+ε2r2and1req=1r1+1r2
Topper's Solved these Questions
- XII BOARDSBook:XII BOARDS PREVIOUS YEARChapter:XII BOARDSExercise:SECTION - D
Explore 6 Videos
- XII BOARDSBook:XII BOARDS PREVIOUS YEARChapter:XII BOARDSExercise:SECTION - E
Explore 6 Videos
- XII BOARDSBook:XII BOARDS PREVIOUS YEARChapter:XII BOARDSExercise:[SET -III, OUTSIDE DELHI]
Explore 5 Videos
- SAMPLE PAPER 2019Book:XII BOARDS PREVIOUS YEARChapter:SAMPLE PAPER 2019Exercise:SECTION D
Explore 6 Videos
Similar Questions
Two cells of emf 2ε and ε and internal resistance 2r and r respectively, are connected in parallel. Obtain the expressions for the equivalent emf and the internal resistance of the combination.
View SolutionTwo cells of emf ε1 and ε2 having internal resistances r1 and r2 respectively are connected in parallel as shown in Fig. Deduce the expressions for the equivalent emf and equivalent internal resistance of a cell which can replace the combination between the pointsB1 and B2 .

View Solution
Knowledge Check
Two batteris of emf ε1 and ε2 wit respective internal resistance r1 and r2 are connected in parallel. Now
AThe effective emf of this parallel combination is (ε1r1+ε2r2)(r1+r2)BThe effective internal resistance of this combination is 2r1r2(r1+r2)Cthe effective emf of this parallel combination is (ε1r2+ε2r1)(r1+r2)DThe effective internal resistance of this combination is r1r2(r1+r2)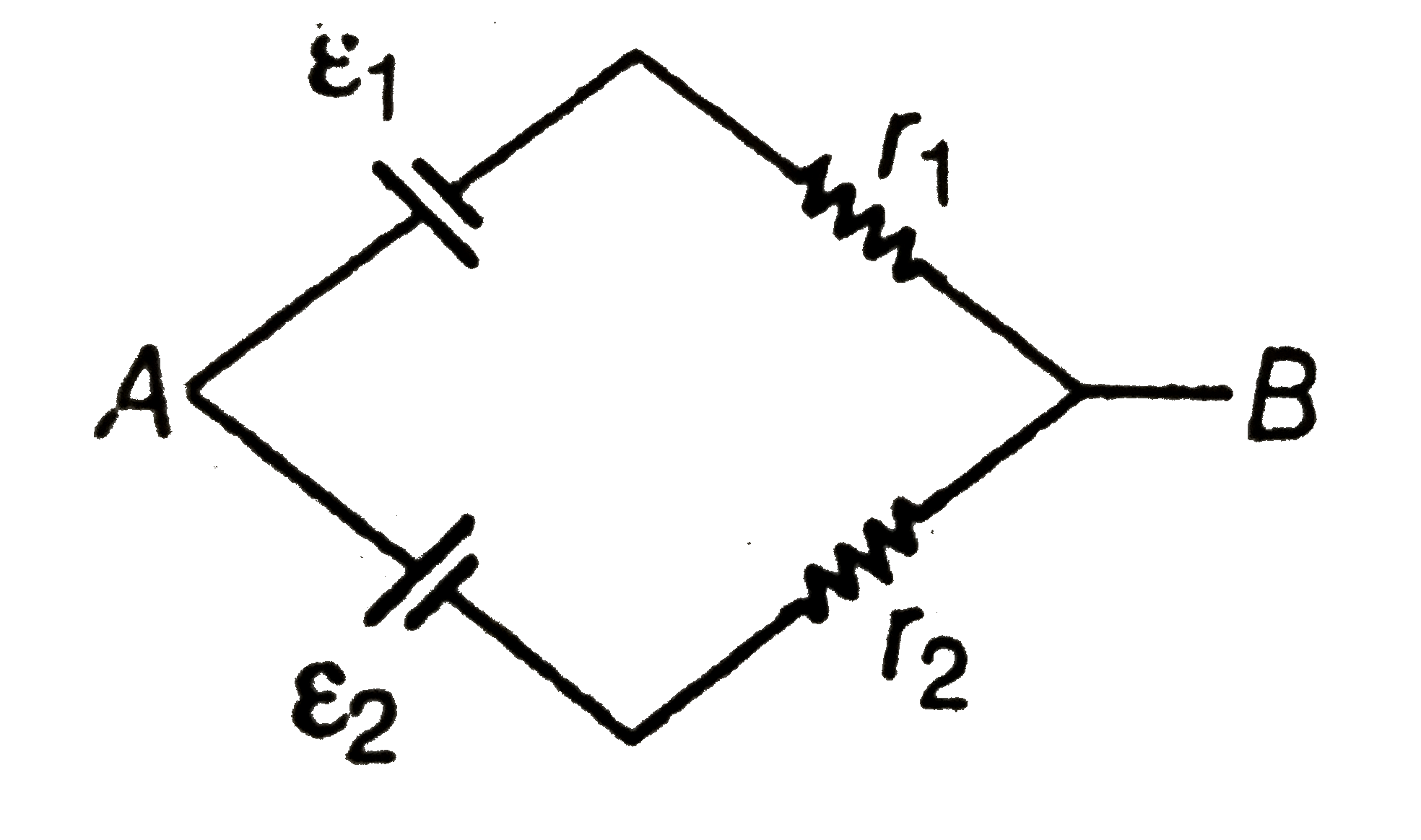
Two batteries of emf ε1andε2(ε2>ε1) and internal resistance r1andr2 respectively are connected in parallel as shown in figure.AThe equivalent emf εeq of the two cells is between ε1andε2, i.e., ε1<εeq<ε2Bthe equivalent emf εeq is smaller than ε1CThe εeq is given by εeq=ε1+ε2 always.Dεeq is independent of internal resistance r1andr2.Two batteries of emf ε1 and ε2(ε2>ε1 and internal resistances r1 and r2 respectively are connected in parallel as shown in Fig. 2 (EP).1.
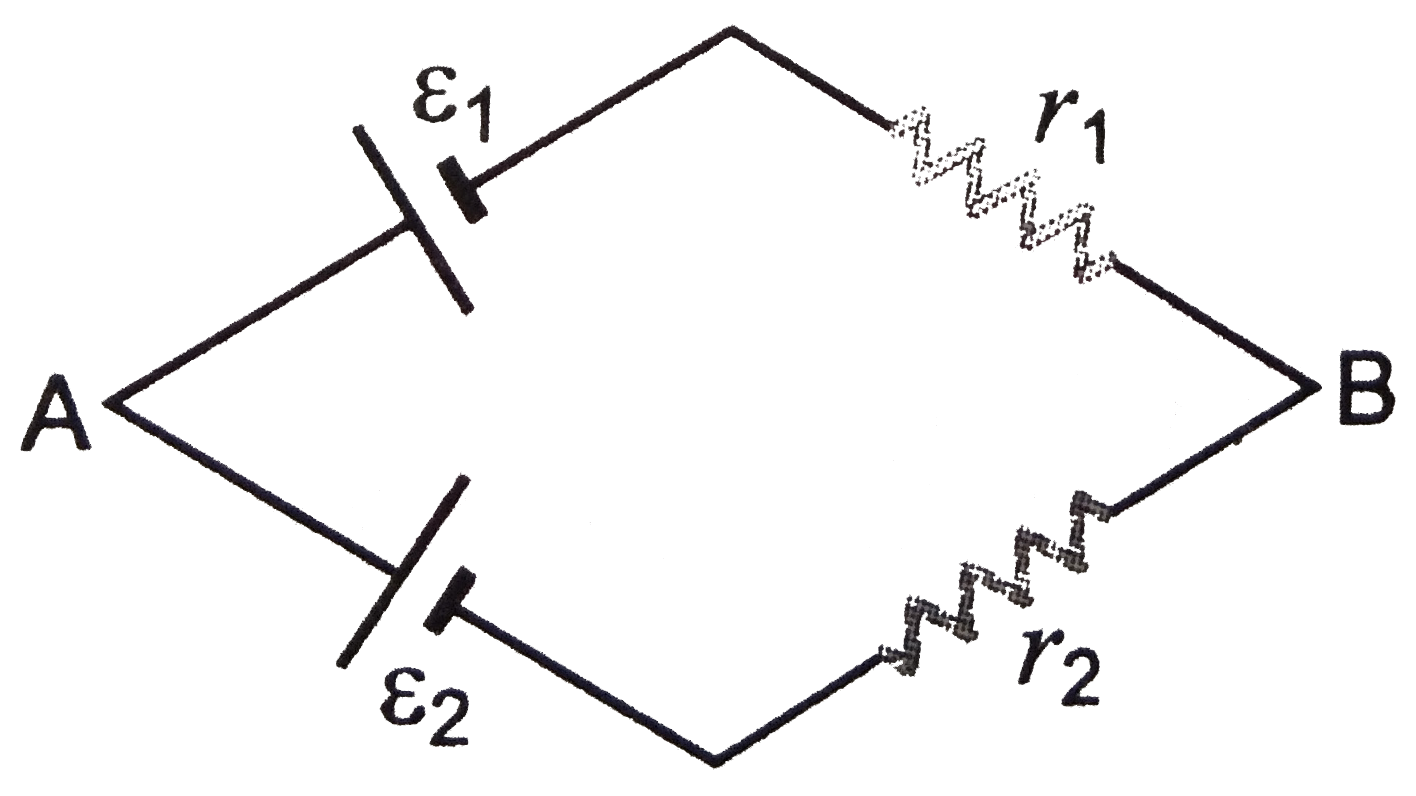 AThe equivalent emf εeq of the two cells is between ε1 and ε2,i.e.,ε1<εeq<ε2BThe quivalent emf εeq is smaller than ε1CThe εeq is given by εeq=ε1+ε2 alwaysDεeq is independent of internal resistances r1 and r2
AThe equivalent emf εeq of the two cells is between ε1 and ε2,i.e.,ε1<εeq<ε2BThe quivalent emf εeq is smaller than ε1CThe εeq is given by εeq=ε1+ε2 alwaysDεeq is independent of internal resistances r1 and r2
(a) Two cells of emf ε1 and ε2 having internal resistances r1 andr2 respectively are connected in parallel as shown in Fig. Deduce the expressions for the equivalent emf and equivalent internal resistance of a cell which can replace the combination between the points B1 and B2 . (b) Calculate the current shown by the ammeter in the circuit diagram given below
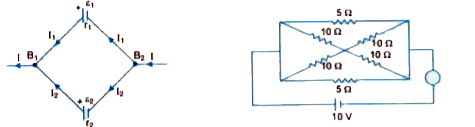
View SolutionTwo batteries of e.m.f. E1 and E2 and internal resistance r1 and r2 are connected in parallel. Determine their equivelent e.m.f.
View SolutionA parallel combination of two cells of emfs ε1 and ε2, and internal resistance r1 and r2 is used to supply current to a load of resistance R. Write the expression for the current through the load in terms of ε1,ε2,r1 and r2.
View SolutionTwo cells of emfs 1.5 V and 2.0v having internal resistance 0.2Σ and 0.3 Σ respectively are connected in parallel . Calculate the emf and internal resistance of the equivalent cell .
View SolutionTwo batteries of emf ε1 and ε2(ε2>ε1) and internal resistances r_1 and r2 respectively are connected in parallel as shown in Fig. Then

View Solution