Define SI unit of current in terms of the force between two parallel current carrying conductors.
(b) Two long straight parallel conductors carrying steady currents IaandIb along the same direction are separated by a distance d. How does one explain the force of attraction between them ? If a third conductor carrying a current Ic in the opposite direction is placed just in the middle of these conductors , find the resultant force acting on the third conductor.
(a) The ampere is the value of that steady current which , when maintained in each of the two very long , straight , parallel conductors of negligible cross -section , and placed one metre apart in vacuum , would produce on each of these conductors a force equal to 2×10−7 newtons per metre of length.
Consider two straight , parallel , long current carrying conductors AB and CD carrying currents IaandIb respectively in same direction . The distance between the two conductors is d .
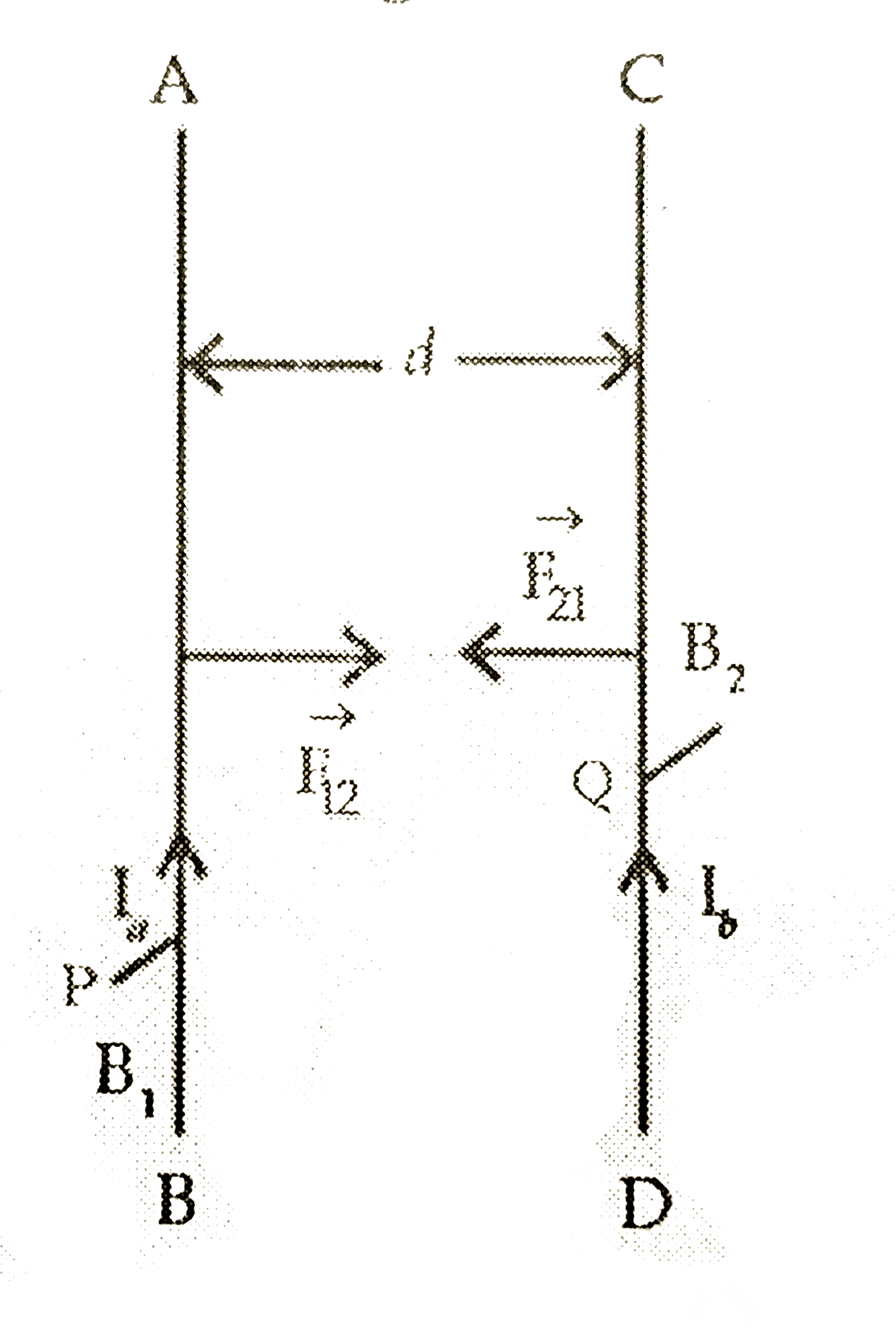
Magnetic field developed at point Q due to the conductor AB carrying current Ia will be given by
B2=μ0Ia2πd (acting normal into the plane of paper)
Force on conductor CD due to AB F21=B2Iblsinθ
F21=μ0Ia2πdIblsin90∘
(towards left according to Fleming 's left hand rule)
F21=μ04π2IaIbld
Force on unit length
F21l=μ04π2IaIbd
→F12=−→F21 (accoroding to third law of motion)
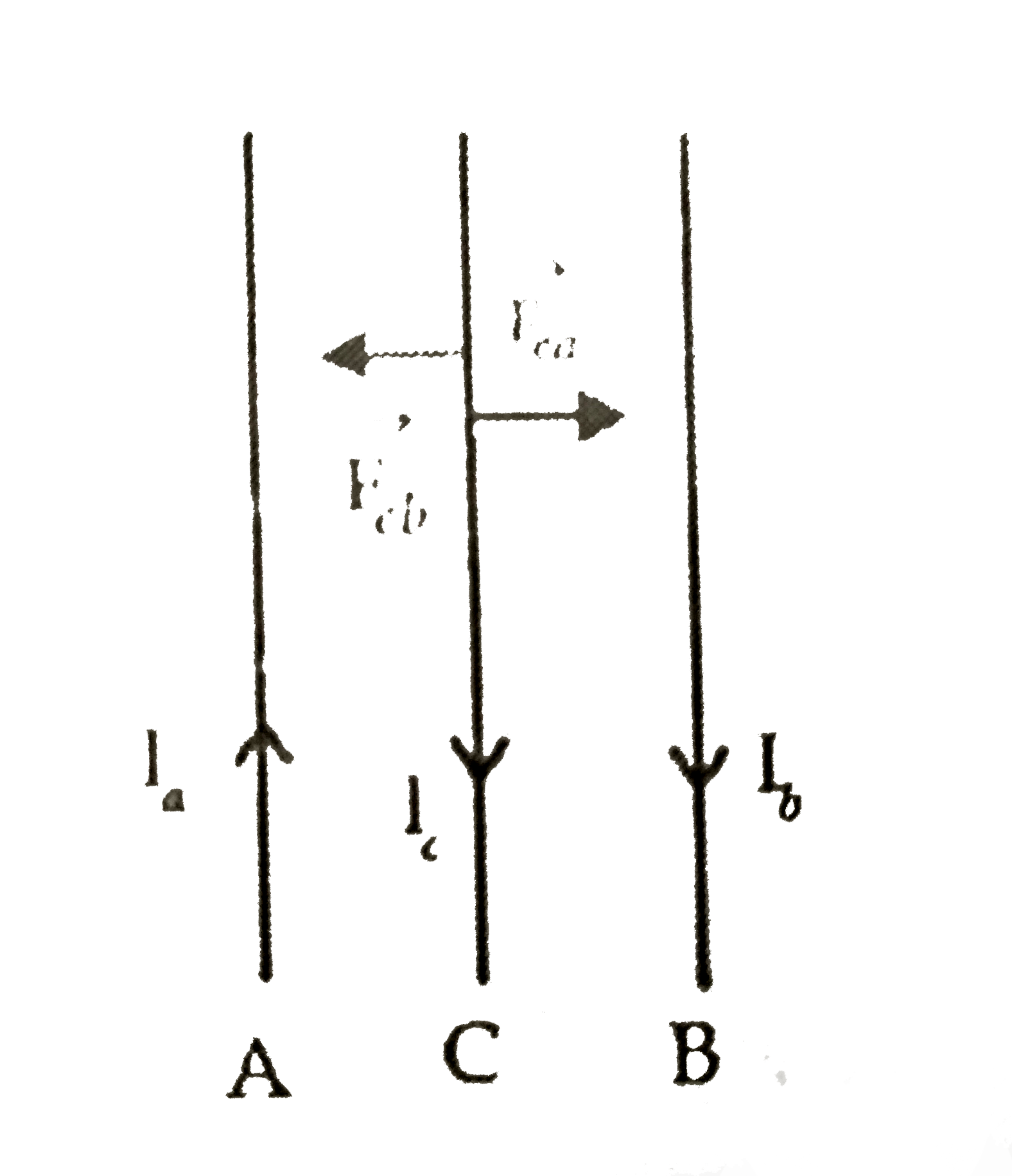
Force on conductor C due to the conductor A
Fca=μ04π2IaIcd/2 . . . (i) (torwards right)
Force on conductor C due to the conductor B
Fob=μ04π2IbIcd/2 . . . (ii) (towards left)
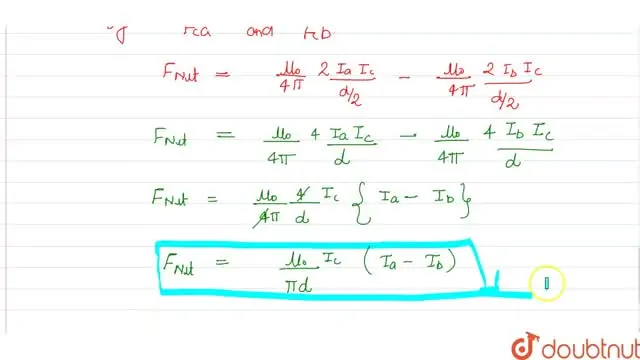
Net force on the conductor C will be the difference of Fcaandcb
Fnet=μ04π4IaIcd~μ04π4IbIcd
Fnet=μ0Icπd(Ia~Ib)
Related Playlists
- BOOK - XII BOARDS PREVIOUS YEARCHAPTER - XII BOARDSEXERCISE - SECTION - D6videos
XII BOARDS
- BOOK - XII BOARDS PREVIOUS YEARCHAPTER - XII BOARDSEXERCISE - SECTION - E6videos
XII BOARDS
- BOOK - XII BOARDS PREVIOUS YEARCHAPTER - XII BOARDSEXERCISE - [SET -III, OUTSIDE DELHI]5videos
XII BOARDS
- BOOK - XII BOARDS PREVIOUS YEARCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D6videos
SAMPLE PAPER 2019
Similar Questions
Two long straight parallel conductors carrying currents I1andI2 along the same direction are separated by a distance 'd'. How does one explain the force of attraction between them?
If a third conductors carrying a current I3 in the opposite direction is placed just in the middle of these conductors , find the resultant force acting on the third conductor.View SolutionTwo parallel wires carrying currents I1 and I2 in opposite directions and separated by a distance d experience a
View SolutionTwo parallel wires carrying currents I1 and I2 in opposite directions and separated by a distance d experience a
View SolutionThe force of attraction between two long parallel conductors 1 metre apart carrying unit ampere current in same direction is
View SolutionThe two linear parallel conductors carrying currents in the opposite direction…………………each other.
View SolutionDerive an expression for the force between two long parallel current carrying conductors. Use this expression to define SI unit of current.
View SolutionFind the force between two parallel conductors carrying currents (i) in the same direction (ii) in opposite directions and hence define one ampere.
View SolutionThe graph of force per unit legth between two long parallel current carrying conductors and the reciprocal of the distance between them is
View SolutionA and B are two conductors carrying a current I in the same direction, X and y are two electron beams moving in the same direction. Then there will be
 View Solution
View SolutionA and B are two conductors carrying a current i in the same direction x and y are two electron beams moving in the same direction. There will be
 View Solution
View Solution