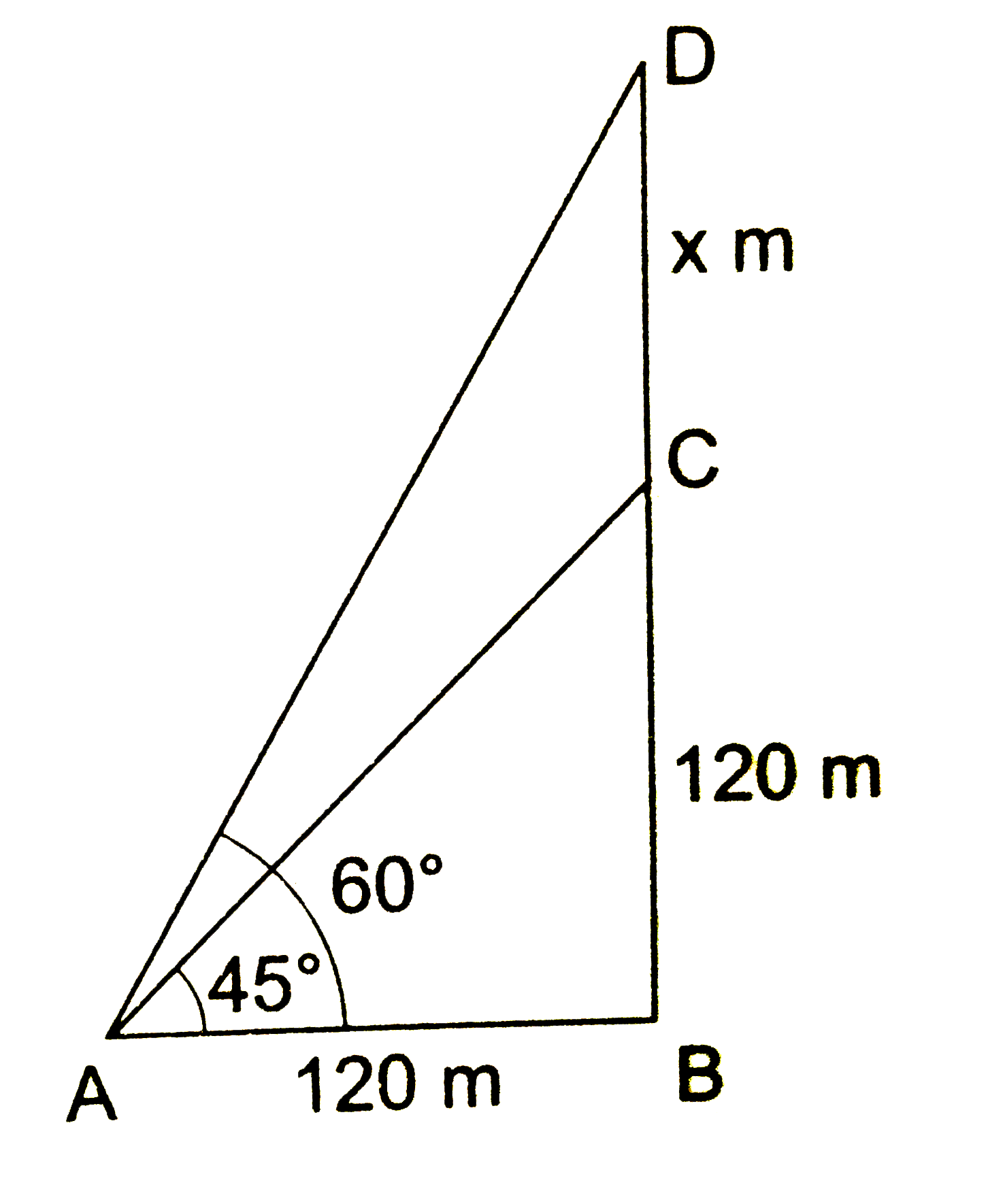
The angle of elevation of the top of a tower at a distance of 120 m from a point A on the ground is 45∘. If the angle of elevation of the top of a flagstaff fixed at the top of the tower, at A is 60∘, then find the height of the flagstaff. [Use √3=1.732.]
The correct Answer is:87.84m
Let BC be the tower and CD be the flagstaff and let AB be the horizontal ground such that AB=120m,∠BAC=45∘ and ∠BAD=60∘. Then,
BCAB=tan45∘=1⇒BC120m=1⇒BC=120m.
Let CD = x metres. Then,
BDAB=tan60∘=√3⇒120+x120=√3. Find x.
Topper's Solved these Questions
- HEIGHTS AND DISTANCESBook:RS AGGARWALChapter:HEIGHTS AND DISTANCESExercise:Multiple Choice Questions (Mcq)
Explore 25 Videos
- HEIGHTS AND DISTANCESBook:RS AGGARWALChapter:HEIGHTS AND DISTANCESExercise:Multiple Choice Questions (Mcq)
Explore 25 Videos
- COORDINATE GEOMETRYBook:RS AGGARWALChapter:COORDINATE GEOMETRYExercise:Multiple Choice Questions (Mcq)
Explore 34 Videos
- LINEAR EQUATIONS IN TWO VARIABLES Book:RS AGGARWALChapter:LINEAR EQUATIONS IN TWO VARIABLES Exercise:QUESTION
Explore 2 Videos
Similar Questions
The angle of elevation of the top of a tower at a point on the ground is 30o . What will be the angle of elevation, if the height of the tower is tripled?
View SolutionIf the angle of elevation of the top of a tower from a point distant 100 m from its base is 45∘, then find the height of the tower.
View Solution
Knowledge Check
The angle of elevation of the top of a half constructed tower at a distance of 150 m from the base of the tower is 30∘. If angle of elevation of top of tower from that point is to be 45∘, then by what amount height of the tower be increased ?
A59.4 mB61.4 mC62.4 mD63.4 mThe angle of elevation of the top of a tower from a point 20 m away from its base is 45∘.What is the height of the tower?
A10 mB20mC30mD40mThe angle of elevation of the top of a tower from the foot of a house, situated at a distance of 20 m from the tower is 60∘. From the top of the top of the house the angle of elevation of the top of the tower os 45∘. Find the height of house and tower.
A14.64m,34.64mB13m,34.64mC14.64m,32mDNone
The angle of elevation of the top of a tower at a point on the ground 20 m from the foot of the tower is 30∘. What is the height of the tower?
View SolutionFrom a point P on the ground the angle of elevation of the top of a tower is 30∘ and that of the top a flagstaff fixed on the top of the tower is 60∘. If the length of the flagstaff is 5m, find the height of the tower.
View SolutionThe angle of elevation of the top of a tower at a point on the ground, 50 m away from the foot of the tower, is 60°. Find the height of the tower.
View SolutionThe angle of elevation of the top of a vertical tower PQ from a point X on the ground is 60o . At a point Y , 40m vertically above X , the angle of elevation of the top is 45o . Calculate the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground is 30∘. After walking 40√3 m towards the tower, the angle of elevation becomes 60∘. Find the height of the tower.
View Solution
