A circle is inscribed ti.e. touches all four sides ) into a rhombous ABCD with one angle 60°. The distance from the centre of the circle to the nearest vertex is equal to 1. If P is any point of the circle then |PA|2+|PB|2+|PC|2+|PD|2 is equal to:
A
12
B
11
C
9
D
none of these
Video Solution
Text Solution
The correct Answer is:2
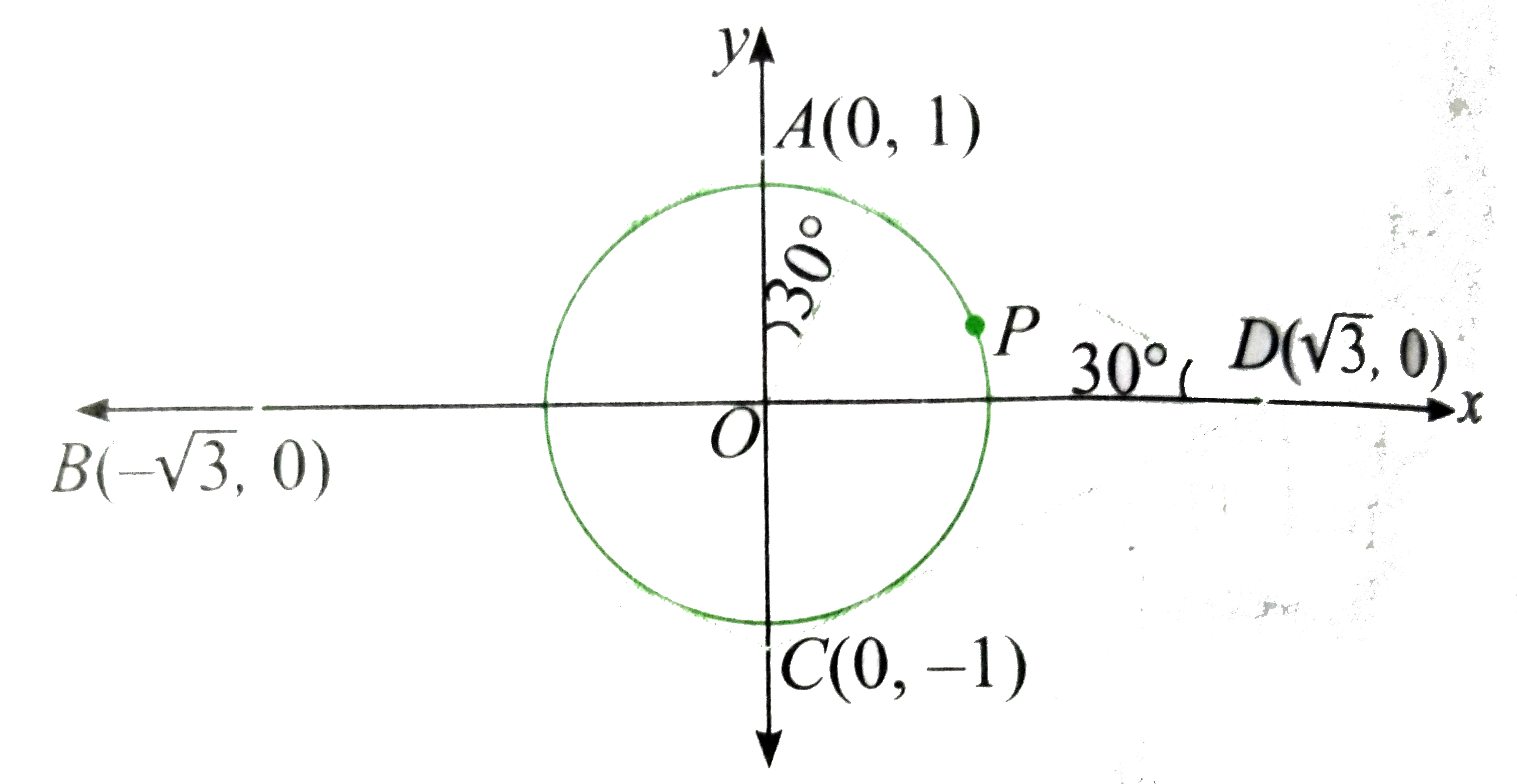
OA=1
r=OAcos30∘=√32
The equation of circle is
x2+y2=34
Let P≡(x1,y2).
PA2+PB2+PC2+PD2=x21+(y1−1)2+(x1+√3)2+y21+x21+(y1+1)2+(x1−√3)2+y21
=4x21+4y21+8=4(x21+y21)+8
=4×34+8=11
|
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Multiple)
25 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise (Comprehension)
46 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.6
5 Videos
- BINOMIAL THEORMBOOK - CENGAGECHAPTER - BINOMIAL THEORMEXERCISE - Question Bank
31 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
32 Videos
Similar Questions
Knowledge Check
O is the centre of a circle. There is a point P in the region of the circle. If PA = PB = PC where A, B and C are points on the circumference of the circle, then OP must be equal to :
APA+PB+PC3BPA+PB+PC2CAB+BC2DzeroFrom a point P, two tangents PA and PB are drawn to a circle with centre O. If OP is equal to diameter of the circle, then ∠APBis
A45∘B90∘C30∘D60∘From a point P two tangents PA and PB are drawn to a circle with centre O.if OP is equal to diameter of the circle then ∠APB is
A60∘B45∘C90∘D30∘
The centre of a circle passing through the points (0, 0), (1, 0) and touching the circle x2+y2=9, is
View SolutionThe centre of a circle passing through the points (0, 0), (1, 0) and touching the circle x2+y2=9, is
View SolutionLet ABCD be a rhombus such that ∠ABC=2π/3. Let S be a circle inscribed in te rhombus ABCD. If ength of a side of the rhombus is 1, then PA2+PB2+PC2+PD2 is equal to.
View SolutionFour circles each with radius 2 touches the axes, then the radius of the largest circle touching all the four circles, is
View SolutionA circle touches the parabola y2=2x at P(12,1) and cuts the parabola at its vertex V. If the centre of the circle is Q, then
View Solution
