Find the dimension of the rectangle of area whose perimeter is the least, Also, find the perimeter of the rectangle
The correct Answer is:length = , breadth , perimeter
To find the dimensions of the rectangle with an area of 96cm2 and the least perimeter, we can follow these steps:
Step 1: Define the Variables
Let the length of the rectangle be L and the width be B. The area of the rectangle is given by:
A=L×B=96cm2
Step 2: Express Width in Terms of Length
From the area formula, we can express B in terms of L:
B=96L
Step 3: Write the Perimeter Formula
The perimeter P of the rectangle is given by:
P=2L+2B
Substituting B from the previous step:
P=2L+2(96L)=2L+192L
Step 4: Differentiate the Perimeter Function
To find the minimum perimeter, we need to differentiate P with respect to L:
dPdL=2−192L2
Step 5: Set the Derivative to Zero
To find the critical points, set the derivative equal to zero:
2−192L2=0
Solving for L2:
192L2=2⟹L2=1922=96⟹L=√96
Step 6: Calculate the Width
Now, substituting L back to find B:
B=96L=96√96=√96
Step 7: Find the Dimensions
Thus, the dimensions of the rectangle are:
L=√96cmandB=√96cm
Step 8: Calculate the Perimeter
Now, we can find the perimeter:
P=2L+2B=2√96+2√96=4√96
Simplifying 4√96:
4√96=4×4√6=16√6cm
Final Answer
The dimensions of the rectangle are √96cm by √96cm, and the minimum perimeter is 16√6cm.
---
Let the length of the rectangle be L and the width be B. The area of the rectangle is given by:
A=L×B=96cm2
From the area formula, we can express B in terms of L:
B=96L
The perimeter P of the rectangle is given by:
P=2L+2B
Substituting B from the previous step:
P=2L+2(96L)=2L+192L
To find the minimum perimeter, we need to differentiate P with respect to L:
dPdL=2−192L2
To find the critical points, set the derivative equal to zero:
2−192L2=0
Solving for L2:
192L2=2⟹L2=1922=96⟹L=√96
Now, substituting L back to find B:
B=96L=96√96=√96
Thus, the dimensions of the rectangle are:
L=√96cmandB=√96cm
Now, we can find the perimeter:
P=2L+2B=2√96+2√96=4√96
Simplifying 4√96:
4√96=4×4√6=16√6cm
The dimensions of the rectangle are √96cm by √96cm, and the minimum perimeter is 16√6cm.
Topper's Solved these Questions
- APPLICATIONS OF DERIVATIVESBook:RS AGGARWALChapter:APPLICATIONS OF DERIVATIVESExercise:Exercise 11G
Explore 32 Videos
- APPLICATIONS OF DERIVATIVESBook:RS AGGARWALChapter:APPLICATIONS OF DERIVATIVESExercise:Exercise 11H
Explore 30 Videos
- APPLICATIONS OF DERIVATIVESBook:RS AGGARWALChapter:APPLICATIONS OF DERIVATIVESExercise:Exercise 11E
Explore 22 Videos
- ADJOINT AND INVERSE OF A MATRIXBook:RS AGGARWALChapter:ADJOINT AND INVERSE OF A MATRIXExercise:Exercise 7
Explore 37 Videos
- AREA OF BOUNDED REGIONS Book:RS AGGARWALChapter:AREA OF BOUNDED REGIONS Exercise:Exercise 17
Explore 39 Videos
Similar Questions
Find the perimeter of the given rectangle
View SolutionFind he perimeter of the rectangle MNOP.
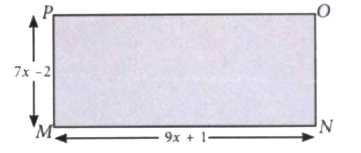
View SolutionArea and perimeter of a rectangle
View SolutionArea and Perimeter of rectangle
View SolutionThe area of a square and a rectangle are equal. If the side of the square is40 cm and the breadth of the rectangle is 25 cm, find the length of therectangle. Also, find the perimeter of the rectangle.
View SolutionThe area of a rectangle is 630 cm2 and its length is 35 cm. find the perimeter of the rectangle.
View SolutionThe area of a rectangle is 192 cm2 and its perimeter is 56 cm. Find the dimensions of the rectangle .
View SolutionThe area of a square and a rectangle are equal.If the side of the square is 40 cm and the breadth of the rectangle is 25 cm,find the length of the rectangle. Also,find the perimeter of the rectangle.
View SolutionFind the area of a rectangle whose length and breadth are 45 cm and 18 cm respectively. Also, find the perimeter of the rectangle.
View SolutionFind the perimeter of a rectangle whose area is 600cm2 and breadth is 25 cm.
View Solution