A cubical region of space with side lengths 0.05 m contains a uniform magnetic field. If the total magnetic energy stored in this region is π J, what is the magnitude of the magnetic field?
To solve the problem, we need to find the magnitude of the magnetic field (B) in a cubical region of space given the total magnetic energy stored in that region.
1. Identify the given values:
- Side length of the cube, L=0.05m
- Total magnetic energy, U=πJ
2. Calculate the volume of the cube:
V=L3=(0.05m)3=0.000125m3
3. Use the formula for magnetic energy density:
The magnetic energy density (energy per unit volume) is given by:
u=B22μ0
where μ0 (the permeability of free space) is approximately 4π×10−7H/m.
4. Relate total energy to energy density:
The total magnetic energy stored in the region can be expressed as:
U=u⋅V
Substituting for u:
U=B22μ0⋅V
5. Substitute the known values:
Now substituting the values we have:
π=B22⋅(4π×10−7)⋅0.000125
6. Simplify the equation:
Rearranging gives:
B2=2π⋅(4π×10−7)⋅0.0001251
7. Calculate B2:
B2=2⋅π⋅4π⋅10−7⋅0.000125
B2=10−7⋅10−3⋅8π2
B2=8⋅10−10⋅π2
8. Take the square root to find B:
B=√8⋅10−10⋅π2=√8⋅π⋅10−5
B=2√2⋅π⋅10−5
9. Convert to a more usable form:
Approximating √2≈1.414:
B≈2.828⋅π⋅10−5T
B≈0.08πTor80πmT
Final Answer:
The magnitude of the magnetic field is approximately 0.08πT or 80πmT.
- Side length of the cube, L=0.05m
- Total magnetic energy, U=πJ
V=L3=(0.05m)3=0.000125m3
The magnetic energy density (energy per unit volume) is given by:
u=B22μ0
where μ0 (the permeability of free space) is approximately 4π×10−7H/m.
The total magnetic energy stored in the region can be expressed as:
U=u⋅V
Substituting for u:
U=B22μ0⋅V
Now substituting the values we have:
π=B22⋅(4π×10−7)⋅0.000125
Rearranging gives:
B2=2π⋅(4π×10−7)⋅0.0001251
B2=2⋅π⋅4π⋅10−7⋅0.000125
B2=10−7⋅10−3⋅8π2
B2=8⋅10−10⋅π2
B=√8⋅10−10⋅π2=√8⋅π⋅10−5
B=2√2⋅π⋅10−5
Approximating √2≈1.414:
B≈2.828⋅π⋅10−5T
B≈0.08πTor80πmT
The magnitude of the magnetic field is approximately 0.08πT or 80πmT.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - PRACTICE QUESTIONS (Single Correct Choice Type)
45 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - PRACTICE QUESTIONS (More than One Correct Choice Type)
13 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHECKPOINTS
9 Videos
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - (PRACTICE QUESTIONS) Integer Type
1 Videos
- ELECTROMAGNETIC OSCILLATIONS AND ALTERNATING CURRENT BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC OSCILLATIONS AND ALTERNATING CURRENT EXERCISE - Practice Questions (Integer)
7 Videos
Similar Questions
Knowledge Check
Magnetic field energy stored in a coil is
ALi2B12LiCLiD12Li2Assertion (A): If an electron is not deflected when moving through a certain region of space, then the only possibility is that no magnetic field is present in that region.
Reason (R): Force on electron is directly proportional to the strength of the magnetic field.ABoth A and R are true and R is the correct explanation of ABBoth A and R are true but R is NOT the correct explanation of ACA is true but R is falseDA is false and R is true
Magnetic Dipole In Uniform Magnetic Field
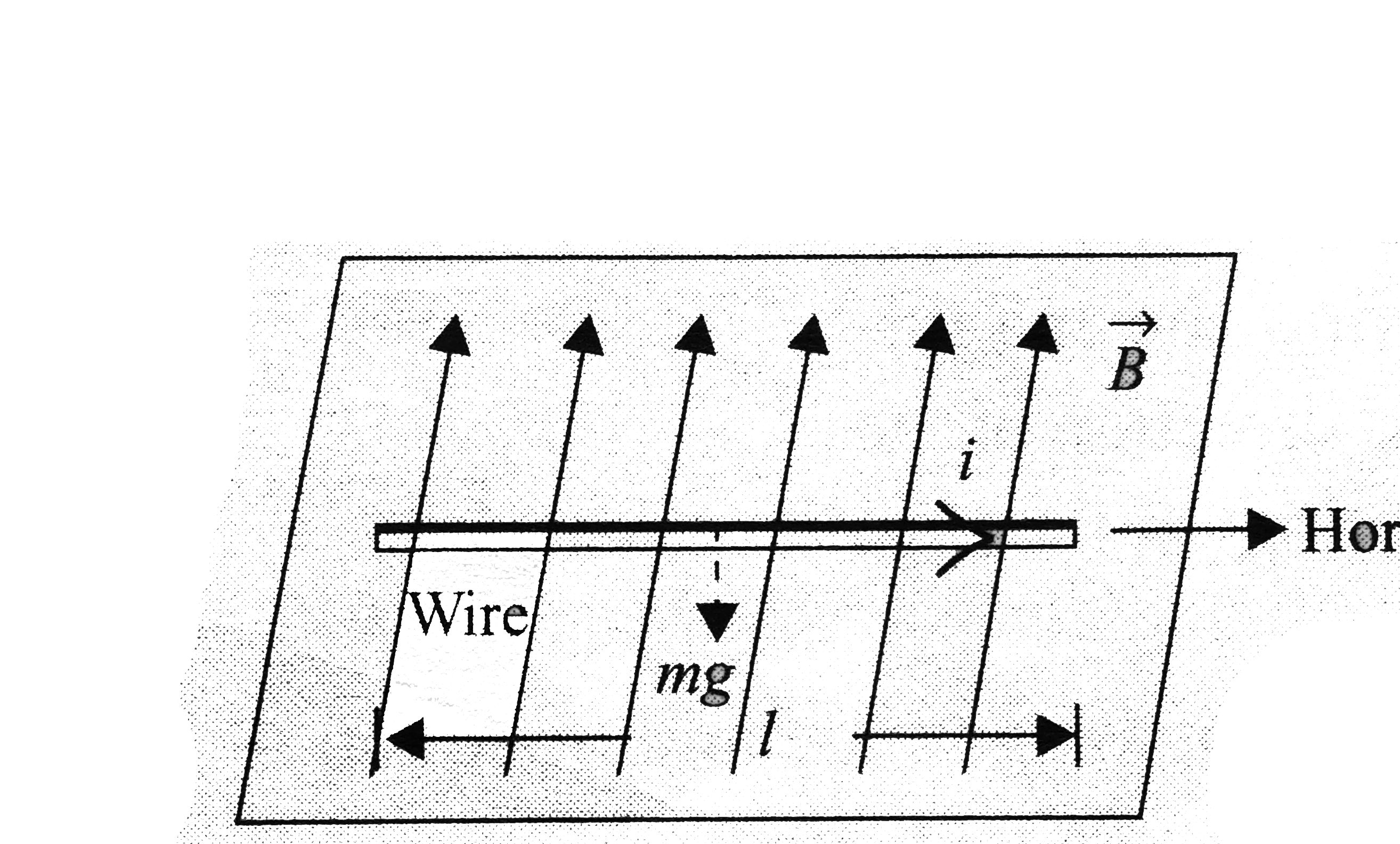
View SolutionA straight wire of mass 200 g and length 1.5 m carries a current of 2 A. It is suspended in mid-air by a uniform horizontal magnetic field B. What is the magnitude of the magnetic field?
View SolutionA short bar magnetic moment of magnetic moment 0.9JT−1, is placed with the axis at 45∘ to a uniform magnetic field. If it experience a torque of 0.063 J, (i) calculate the magnitude of the magnitudic field and (ii) what orientation of the bar magnet corresponds to the stzble equillibrium in the magnetic field ?
View SolutionA straight wire of mass 200 g and length 1.5 m carries a current of 2 A. It is suspended in mid-air by a uniform horizontal magnetic field B. What is the magnitude of the magnetic field?

View SolutionA straight wire, of length L, carrying a current I, stays suspended horizontally in mid air in a region where there is a uniform magnetic field →B. The linear mass density of the wire is λ. Obtain the magnetic of this magnetic field.
View SolutionA short bar magnet of magnetic moment 0⋅9JT−1 is placed with its axis at 30∘ to a uniform magnetic field. It experiences a torque of 0⋅063J. Calculate the magnitude of magnetic field. In which orientation will the bar magnet be in stable equilibrium in the magnetic field?
View Solution
