Two electrons are fixed 4.0 cm apart. Another electron is shot from infinity and stops midway between the two. What is its initial speed?
To solve the problem, we will use the principle of conservation of energy. The initial kinetic energy of the electron when it is shot from infinity will be converted into potential energy when it stops midway between the two fixed electrons.
1. Identify the Given Data:
- Distance between the two fixed electrons, d=4.0cm=0.04m.
- The distance from each fixed electron to the point where the moving electron stops is d2=2.0cm=0.02m.
- Charge of an electron, q=1.6×10−19C.
- Mass of an electron, m=9.1×10−31kg.
2. Calculate the Potential Energy at Midpoint:
- The potential energy U due to one electron at a distance r is given by:
U=k⋅q1⋅q2r
- Since there are two electrons, the total potential energy Utotal at the midpoint (where the moving electron stops) is:
Utotal=2⋅k⋅q2d2=2⋅k⋅q20.02
- Substitute k=9×109N m2/C2:
Utotal=2⋅9×109⋅(1.6×10−19)20.02
3. Calculate the Potential Energy Value:
- Calculate (1.6×10−19)2=2.56×10−38.
- Now, substituting this value into the potential energy equation:
Utotal=2⋅9×109⋅2.56×10−380.02
- Simplifying:
Utotal=2⋅9×2.560.02×10−29=2⋅1152×10−29=2304×10−29J=2.304×10−26J
4. Use Conservation of Energy:
- The initial kinetic energy K of the moving electron when it is shot from infinity is given by:
K=12mv2
- At the midpoint, all the kinetic energy has been converted to potential energy:
12mv2=Utotal
- Rearranging gives:
v2=2Utotalm
5. Substituting Values:
- Substitute Utotal=2.304×10−26J and m=9.1×10−31kg:
v2=2⋅2.304×10−269.1×10−31=4.608×10−269.1×10−31≈506.7×104≈5.067×102
6. Calculate the Final Speed:
- Taking the square root:
v=√5.067×102≈22.5m/s
Final Answer:
The initial speed of the electron when shot from infinity is approximately 22.5m/s.
- Distance between the two fixed electrons, d=4.0cm=0.04m.
- The distance from each fixed electron to the point where the moving electron stops is d2=2.0cm=0.02m.
- Charge of an electron, q=1.6×10−19C.
- Mass of an electron, m=9.1×10−31kg.
- The potential energy U due to one electron at a distance r is given by:
U=k⋅q1⋅q2r
- Since there are two electrons, the total potential energy Utotal at the midpoint (where the moving electron stops) is:
Utotal=2⋅k⋅q2d2=2⋅k⋅q20.02
- Substitute k=9×109N m2/C2:
Utotal=2⋅9×109⋅(1.6×10−19)20.02
- Calculate (1.6×10−19)2=2.56×10−38.
- Now, substituting this value into the potential energy equation:
Utotal=2⋅9×109⋅2.56×10−380.02
- Simplifying:
Utotal=2⋅9×2.560.02×10−29=2⋅1152×10−29=2304×10−29J=2.304×10−26J
- The initial kinetic energy K of the moving electron when it is shot from infinity is given by:
K=12mv2
- At the midpoint, all the kinetic energy has been converted to potential energy:
12mv2=Utotal
- Rearranging gives:
v2=2Utotalm
- Substitute Utotal=2.304×10−26J and m=9.1×10−31kg:
v2=2⋅2.304×10−269.1×10−31=4.608×10−269.1×10−31≈506.7×104≈5.067×102
- Taking the square root:
v=√5.067×102≈22.5m/s
The initial speed of the electron when shot from infinity is approximately 22.5m/s.
Topper's Solved these Questions
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - (PRACTICE QUESTIONS) Single Correct Choice Type
21 Videos
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - (PRACTICE QUESTIONS) More than One Correct Choice Type
8 Videos
- ELECTRIC POTENTIALBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - CHECKPOINT
6 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Questions (Integer Type )
3 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - PRACTICE QUESTIONS (Integer Type)
14 Videos
Similar Questions
An electron is projected with an initial velocity of 107ms−1 into an electric field between two parallel plates 1 cm apart. The electron enters the field at a point midway between the plates. If the electron just misses the positivity charged plate as it emerges from the field, find the magnnitude of the electric density between the plates. (Length of each plate is 2 cm, mass of electron=9×10−31kg and charge of electron =1.6×10−19C)
View SolutionTwo long, straight parallel conductors each carrying 2A current in the same direction are 10 cm apart. Calculate the magnetic field at a distance a) at a point which is midway between the wires b) at a point which is 15 cm from one wire and 5cm from the other.
View Solution
Knowledge Check
A covalent bond results from the sharing of electrons between two atoms of
Ametallic elementsBnonmetallic elementsCmetalloid elementsDzero or relatively small electronegativity differenceTwo particles, each of mass m, are a distance d apart. To bring a third particle, also having mass m, from far away to the point midway between the two particles an external agent does work given by:
A4Gm2/dB−4Gm2/dC4Gm2/d2D−4Gm2/d2An electron is projected with an initial speed v0=1.60×106ms−1 into the uniform field between the parallel plates as shown in fig. Assume that the field between the plates is uniform and directed vertically downward, and that the field outside the plates is zero. the electrons enters the field at a point midway between the plates. Mass of electron is 9.1×10−31kg.
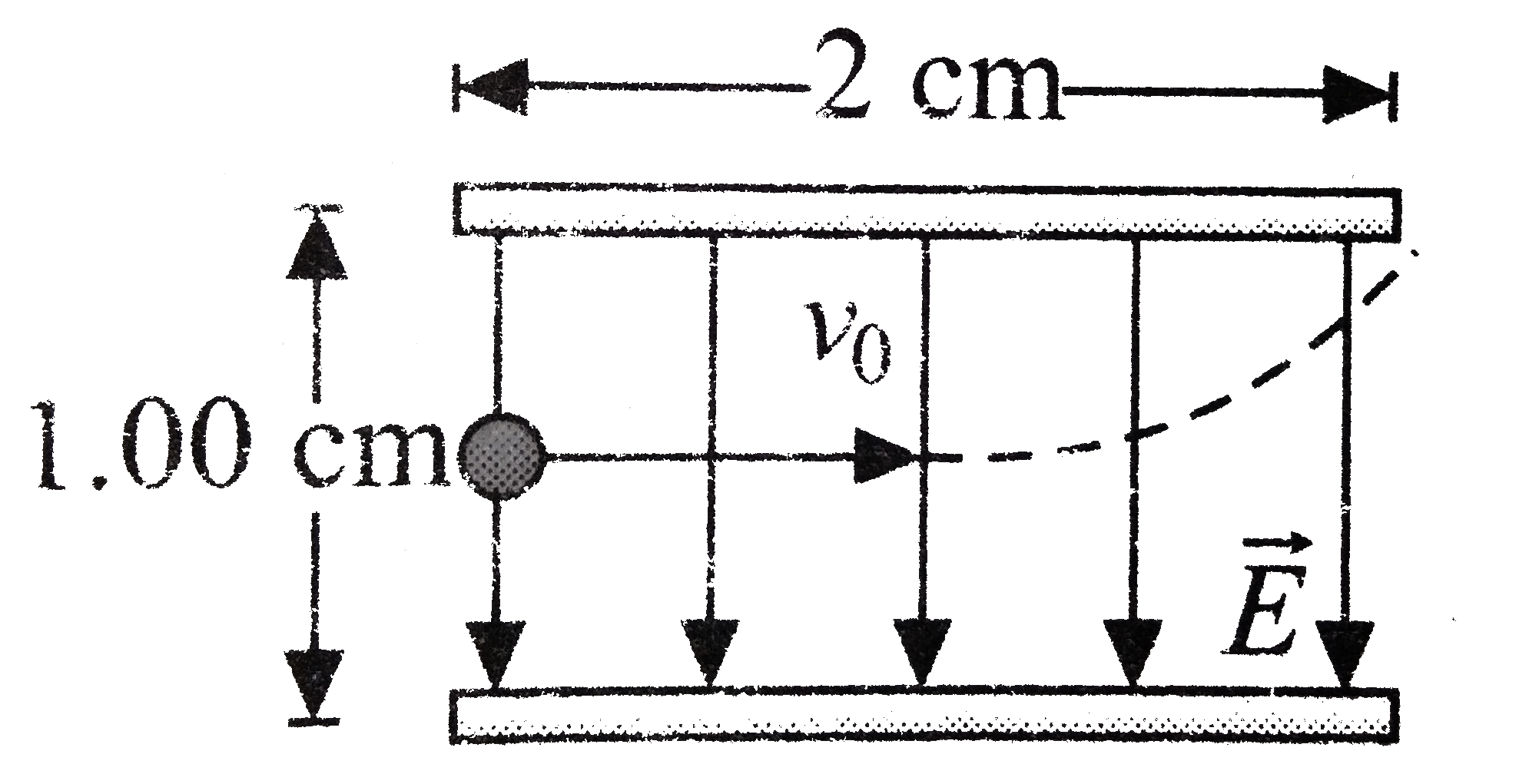
The vertical displacement traveled by the proton as it exits the region between the plated is (mass pf proton is 1.67×10−27kg).A1.6×10−8mB3.25×10−8mC5.25×10−6mD2.73×10−6m
When the terminals of a 100 V battery are connected to two large parallel plates 1 cm apart, a uniform field →E is set up inside the region between the two plates. If the direction of the filed →E is vertically upwards, determine the force on the electron in this field. Compare this force with the weight of the electron.
View SolutionTwo electrons are fixed at a seperation of 2d from each other.A proton is placed at the mid point and displaced slightly in a direction perpendicular to line joining the two electrons. Find frequency of oscillation of proton
View SolutionThe masses and radii of the Earth and the Moon are M1,R1andM2,R2 respectively. Their centres are at a distance d apart. The minimum speed with which a particel of mass m should be projected from a point midway between the two centres so as to escape to infinity is ........
View SolutionTwo parallel wires carry currents of 20A and 40A in opposite directions. Another wire carrying a current antiparallel to 20A is placed midway between the two wires. The magnetic force on it will be
View SolutionTwo charged metal plates in vacuum are 10cm apart. A uniform electric field of intensity (45/16)×103NC−1 is applied between the plates. An electron is released between the plates from rest at a point just outside the negative plate.
(i) Calculate how long (t) will the electron take to reach the other plate?
(ii) At what velocity (v) will it be electron take to hits the other plate?
View Solution
