ABC is triangle. D is the middle point of BC. If AD is perendicular to AC, then prove that
$cos A cos C = \frac{2 (c^{2} - a^{2})}{3 a c}$

Video Solution

Text Solution

Verified by Experts

In $Δ A D C,$ we have

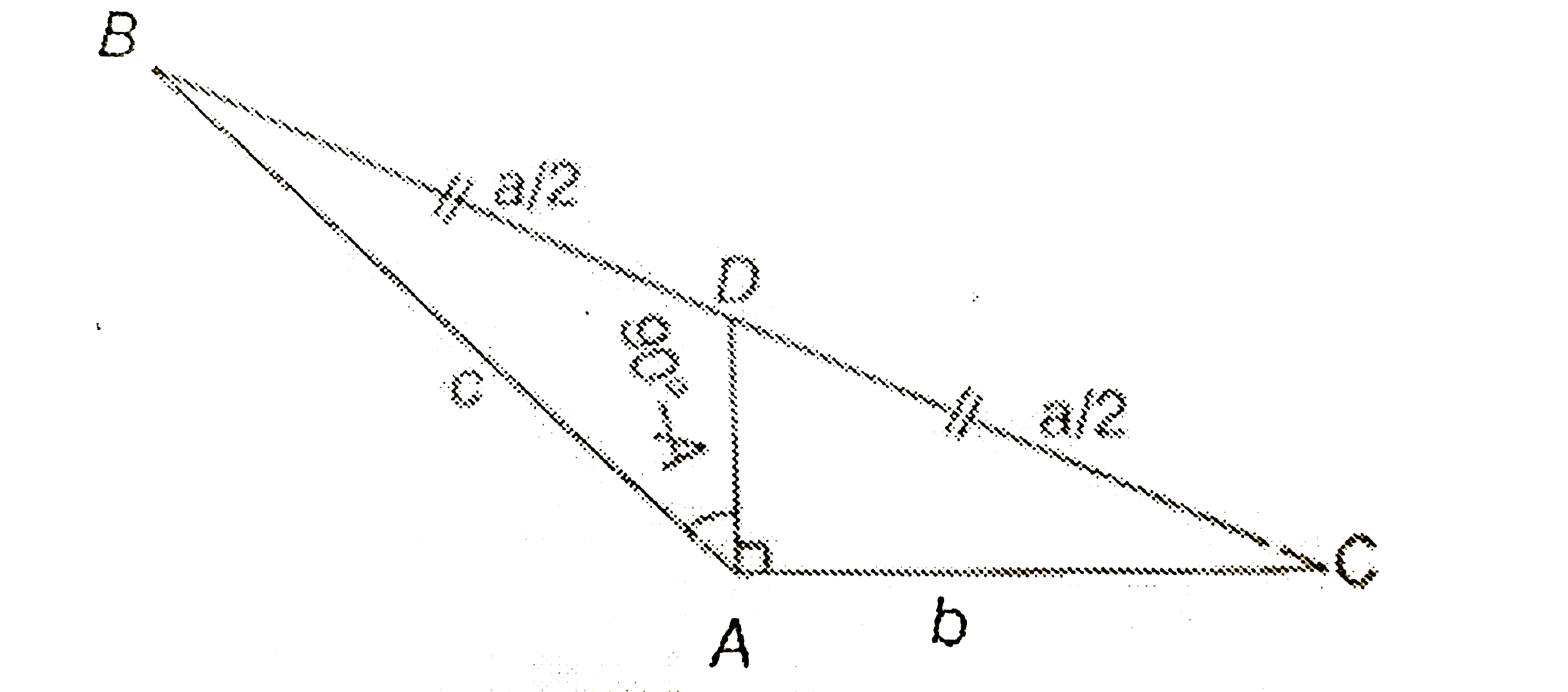
$cos C = \frac{A C}{C D}$
$cos C = \frac{2 b}{a} ... (i)$
Applying cosine formula in $Δ A B C$ , we have
$cos A = \frac{b^{2} + c^{2} - a^{2}}{2 b c}$
and $cos C = \frac{a^{2} + b^{2} - c^{2}}{2 a b} ... . (i i)$
From Eqs.(i) and (ii),
$\frac{a^{2} + b^{2} - c^{2}}{2 a b} = \frac{2 b}{a}$
$\Rightarrow a^{2} + b^{2} - c^{2} = 4 b^{2}$
$\Rightarrow a^{2} - c^{2} = 3 b^{2} ... . (i i i)$
Now, $cos A cos C = \frac{b^{2} + c^{2} - a^{2}}{2 b c} \cdot \frac{2 b}{a}$
$\frac{b^{2} + c^{2} - a^{2}}{b c} = \frac{3 b^{2} + 3 (c^{2} - a^{2})}{3 a c}$
$\frac{(a^{2} + c^{2}) + 3 (c^{2} - a^{2})}{3 a c} = \frac{2 (c^{2} - a^{2})}{3 a c}$

Updated on:21/07/2023

ABC is a triangle. D is the middle point of BC. If AD is perpendicular to AC, The value of cos A cos C , is
A $(3 \frac{c^{2} - a^{2}}{a c}$
B $\frac{a^{2} - c^{2}}{2 a c}$
C $(2 \frac{c^{2} - a^{2}}{3 a c}$
Dnone of these
If D id the mid-point of the side BC of a triangle ABC and AD is perpendicular to AC , then
A $b^{2} = a^{2} - c^{2}$
B $a^{2} + b^{2} = 5 c^{2}$
C $3 b^{2} = a^{2} - c^{2}$
D $3 a^{2} = b^{2} - 3 c^{2}$
If D id the mid-point of the side BC of a triangle ABC and AD is perpendicular to AC , then
A $a^{2} + b^{2} + c^{2} = 4 b^{2}$
B $a^{2} + b^{2} - c^{2} = 4 b^{2}$
C $a^{2} - b^{2} + c^{2} = 4 b^{2}$
D $a^{2} - b^{2} - c^{2} = 4 b^{2}$