In a triangle the sum of lengths of two sides sides x and the product of the lengths of the saem two sides is y. if x2−c2=y, where c is the length of the third side of then triangle, then the circumradius of the triangle is

A

c3

B

c√3

C

32y

D

y√3

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:B

We know that asinA=bsinB=csinC=2R
and given that, a+b=ab=yandx2−c2=y
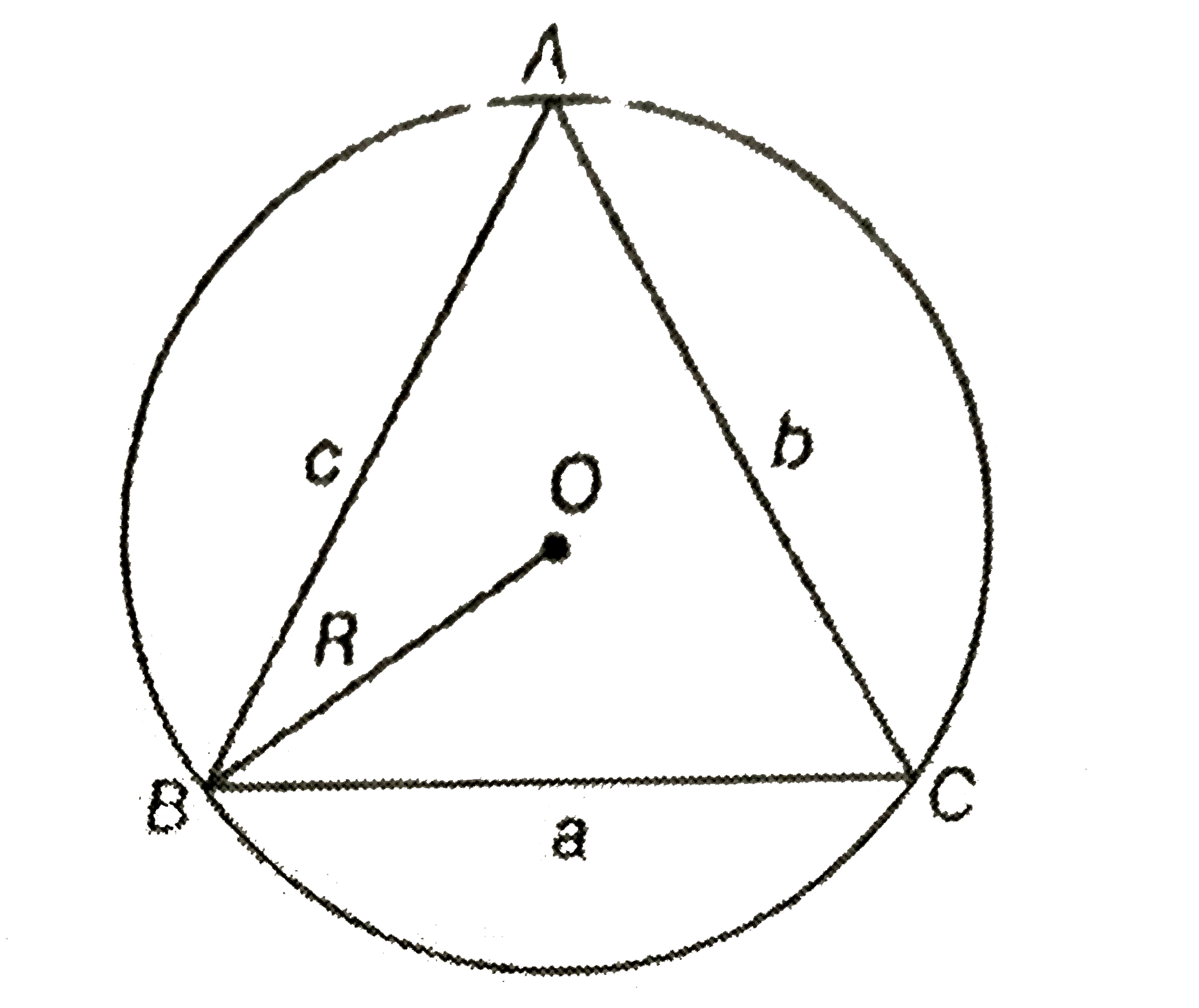
ocr_image
∴(a+b)2−c2=ab
⇒a2+b2−c2=−2ab+ab
⇒a2+b2−c2=−ab
⇒a2+b2−c22ab=−ab2ab=−12
∴cosC=−12⇒C=120∘
[Using cosine rule, cosC=a2+b2−c22ab]
Now, csinc=2R
⇒R=12csin(1202)=c2=2√3
∴R=c√3

Updated on:21/07/2023
|
Class 12MATHS PROPERTIES OF TRIANGLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • In a triangle the sum of two sides is x and the product of the same two sides is y .If x2−c2=y, where c is the third side of the triangle , then the ratio of the in-radius to the circum-radius of the triangle is

    A3y2x(x+c)
    B3y2c(x+c)
    C3y4x(x+c)
    D3y4c(x+c)

  • If a, b,c are the lengths of the sides of triangle , then

    Aa−b>c
    Ba+b<c
    Cc=a+b
    Dc<a+b

  • The sum of length of two sides of a triangle is always

    Aless than third
    Bgreater than third
    Cequal to third
    D23 to third